Abstract
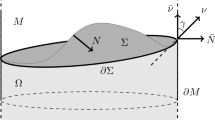
In this paper, we deal with marginally outer trapped surfaces (MOTS) immersed in the de Sitter space \(\mathbb {S}_1^{n+2}\). In this setting we are able to obtain a Simons formula for the null second fundamental form and under some appropriate constraints on the MOTS, we apply a weak maximum principle in order to guarantee that it must be either a totally geodesic submanifold or isometric to an open piece of an isoparametric submanifold with two distinct principal curvatures one of which is simple. In this last case, supposing that the initial data where the MOTS lying is a totally umbilical spacelike hypersurface of \(\mathbb {S}_1^{n+2}\), we conclude that it must be either isometric to a circular cylinder, a hyperbolic cylinder or a Clifford torus.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Alencar, H., do Carmo, M.: Hypersurfaces with constant mean curvature in spheres. Proc. Am. Math. Soc. 120, 1223–1229 (1994)
Alías, L.J., Mastrolia, P., Rigoli, M.: Maximum Principles and Geometric Applications, Springer Monographs in Mathematics. Springer, Cham (2016)
Alías, L.J., García-Martínez, S.C.: On the scalar curvature of constant mean curvature hypersurfaces in space forms. J. Math. Anal. Appl. 363(2), 579–587 (2010)
Andersson, L., Eichmair, M., Metzger, J.: Jang’s equation and its applications to marginally trapped surfaces. In: Complex Analysis and Dynamical Systems IV: Part 2. General Relativity, Geometry, and PDE, Contemporary Mathematics, vol. 554, (AMS and Bar-Ilan) (2011)
Andersson, L., Mars, M., Simon, W.: Local existence of dynamical and trapping horizons. Phys. Rev. Lett. 95, 111102 (2005)
Ashtekar, A., Krishnan, B.: Dynamical horizons and their properties. Phys. Rev. D (3) 68(10), 104030 (2003). 25
Chruściel, P.T., Galloway, G.J., Solis, D.: Topological censorship for Kaluza–Klein space-times. Ann. Henri Poincaré 10(5), 893–912 (2009)
Hawking, S.W., Ellis, G.F.R.: The Large Scale Structure of Space-Time. Cambridge Monographs on Mathematical Physics, vol. 1. Cambridge University Press, London (1973)
Hawking, S.W., Penrose, R.: The singularities of gravitational collapse and cosmology. Proc. R. Soc. Lond. A 314, 529–548 (1970)
Huber, A.: On subharmonic functions and differential geometry in the large. Comment. Math. Helv. 32, 13–72 (1957)
Lawson, H.B.: Local rigidity theorems for minimal hypersurfaces. Ann. Math. 89, 187–197 (1969)
Montiel, S.: An integral inequality for compact spacelike hypersurfaces in de Sitter space and applications to the case of constant mean curvature. Indiana Univ. Math. J. 37, 909–917 (1988)
Nomizu, K., Smith, B.: A formula of Simons’ type and hypersurfaces with constant mean curvature. J. Diff. Geom. 3, 367–377 (1969)
Okumura, M.: Hypersurfaces and a pinching problem on the second fundamental tensor. Am. J. Math. 96, 207–213 (1974)
Penrose, R.: Gravitational collapse and space-time singularities. Phys. Rev. Lett. 14, 57–59 (1965)
Pigola, S., Rigoli, M., Setti, A.G.: A remark on the maximum principle and stochastic completeness. Proc. Am. Math. Soc. 131, 1283–1288 (2003)
Schoen, R., Yau, S.T.: Proof of the positive mass theorem. II. Commun. Math. Phys. 79(2), 231–260 (1981)
Simons, J.: Minimal varieties in Riemannian manifolds. Ann. Math. 88, 62–105 (1968)
Xu, H.W.: A rigidity theorem for submanifolds with parallel mean curvature in a sphere. Arch. Math. (Basel) 61, 489–496 (1993)
Acknowledgements
The authors would like to thank the referee for his/her comments and suggestions which enabled them to improve this work. The first author is partially supported by CNPq, Brazil, Grant 456755/2014-4 and FAPEAL. The second author is partially supported by CNPq, Brazil, Grant 303977/2015-9. The third author is partially supported by CNPq, Brazil, Grant 431976/2018-0.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Batista, M., de Lima, H.F. & Santos, F.R.d. On the classification of MOTS in the de Sitter space. manuscripta math. 162, 159–169 (2020). https://doi.org/10.1007/s00229-019-01123-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00229-019-01123-y