The Sign Clusters of the Massless Gaussian Free Field Percolate on ${\mathbb{Z}^{d}, d \geqslant 3}$ (and more)

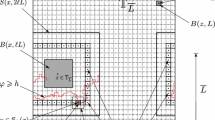
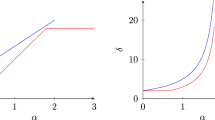
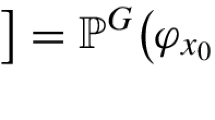Abstract

We investigate the percolation phase transition for level sets of the Gaussian free field on ${\mathbb{Z}^{d}}$, with ${d \geqslant 3}$, and prove that the corresponding critical parameter h_*(d) is strictly positive for all ${d \geqslant 3}$, thus settling an open question from (Rodriguez and Sznitman in Commun Math Phys 320(2):571–601, 2013). In particular, this implies that the sign clusters of the Gaussian free field percolate on ${\mathbb{Z}^{d}}$, for all ${d \geqslant 3}$. Among other things, our construction of an infinite cluster above small, but positive level h involves random interlacements at level u > 0, a random subset of ${\mathbb{Z}^{d}}$ with desirable percolative properties, introduced in Sznitman (Ann Math (2) 171(3):2039–2087, 2010) in a rather different context, a certain Dynkin-type isomorphism theorem relating random interlacements to the Gaussian free field (Sznitman in Electron Commun Probab 17(9):9, 2012), and a recent coupling of these two objects (Lupu in Ann Probab 44(3):2117–2146, 2016), lifted to a continuous metric graph structure over ${\mathbb{Z}^{d}}$.