Skip to main content
SpringerLink
Log in

On the Gromov–Witten/Donaldson–Thomas Correspondence and Ruan’s Conjecture for Calabi–Yau 3-Orbifolds

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

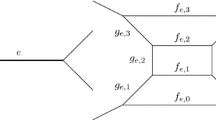
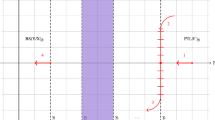
For any toric Calabi–Yau 3-orbifold with transverse A-singularities, we prove the Gromov–Witten/Donaldson–Thomas correspondence and Ruan’s crepant resolution conjecture in all genera.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Aganagic M., Klemm A., Mariño M., Vafa C.: The topological vertex. Commun. Math. Phys. 254(2), 425–478 (2005)

    Article  MATH  ADS  Google Scholar 

  2. Brini, A., Cavalieri, R., Ross, D.: Crepant resolutions and open strings (2013). arXiv:1309.4438

  3. Bryan J., Cadman C., Young B.: The orbifold topological vertex. Adv. Math. 229(1), 531–595 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  4. Behrend K.: Donaldson–Thomas type invariants via microlocal geometry. Ann. Math. (2) 170(3), 1307–1338 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  5. Coates T., Corti A., Iritani H., Tseng H.-H.: Computing genus-zero twisted Gromov–Witten invariants. Duke Math. J. 147(3), 377–438 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  6. Diaconescu D.-E., Florea B.: Localization and gluing of topological amplitudes. Commun. Math. Phys. 257, 119 (2005)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  7. Maulik D., Nekrasov N., Okounkov A., Pandharipande R.: Gromov–Witten theory and Donaldson–Thomas theory. I. Compos. Math. 142(5), 1263–1285 (2006)

    MATH  MathSciNet  Google Scholar 

  8. Maulik D., Oblomkov A., Okounkov A., Pandharipande R.: Gromov–Witten/Donaldson–Thomas correspondence for toric 3-folds. Invent. Math. 186(2), 435–479 (2011)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  9. Ross D.: Localization and gluing of orbifold amplitudes: the Gromov–Witten orbifold vertex. Trans. Am. Math. Soc. 366(3), 1587–1620 (2014)

    Article  MATH  Google Scholar 

  10. Ross, D.: Donaldson–Thomas theory and resolutions of toric transverse A-singularities (2014, preprint). arXiv:1409.7011 [math]

  11. Ross D., Zong Z.: The gerby Gopakumar-Mariño-Vafa formula. Geom. Topol. 17(5), 2935–2976 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  12. Ross, D., Zong, Z.: Two-partition cyclic Hodge integrals and loop Schur functions (2014). arXiv:1401.2217

  13. Zhou, J.: Crepant resolution conjecture in all genera for type A singularities (2008, preprint). arXiv:0811.2023 [math]

  14. Zong Z.: Generalized Mariño-Vafa formula and local Gromov–Witten theory of orbi-curves. J. Differ. Geom. 100(1), 161–190 (2015)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Michigan, Ann Arbor, MI, 48109, USA

    Dustin Ross

Authors
  1. Dustin Ross
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dustin Ross.

Additional information

Communicated by N. A. Nekrasov

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ross, D. On the Gromov–Witten/Donaldson–Thomas Correspondence and Ruan’s Conjecture for Calabi–Yau 3-Orbifolds. Commun. Math. Phys. 340, 851–864 (2015). https://doi.org/10.1007/s00220-015-2438-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-015-2438-1

Keywords

Search

Navigation