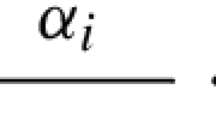Skip to main content
SpringerLink
Log in

Quivers, Quasi-Quantum Groups and Finite Tensor Categories

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

We study finite quasi-quantum groups in their quiver setting developed recently by the first author. We obtain a classification of finite-dimensional pointed Majid algebras of finite corepresentation type, or equivalently a classification of elementary quasi-Hopf algebras of finite representation type, over the field of complex numbers. By the Tannaka-Krein duality principle, this provides a classification of the finite tensor categories in which every simple object has Frobenius-Perron dimension 1 and there are finitely many indecomposable objects up to isomorphism. Some interesting information of these finite tensor categories is given by making use of the quiver representation theory.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abramsky S., Duncan R.: Mathematical Structures in Computer Science 16(3), 469–489 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  2. Assem, I., Simson, D., Skowronski, A.: Elements of the Representation Theory of Associative Algebras. Vol. 1. Techniques of Representation Theory. London Mathematical Society Student Texts, 65. Cambridge: Cambridge University Press, 2006

  3. Auslander, M., Reiten, I., Smalø, S.O.: Representation Theory of Artin Algebras. Cambridge Studies in Adv. Math. 36. Cambridge: Cambridge Univ. Press, 1995

  4. Bakalov, B., Kirilov, A. Jr.: Lectures on tensor categories and modular funtors. Providence, RI: Amer. Math. Soc., 2001

  5. Bulacu D., Caenepeel S.: Integrals for (dual) quasi-Hopf algebras. Applications. J. Algebra 266(2), 552–583 (2003)

    MATH  MathSciNet  Google Scholar 

  6. Calaque D., Etingof P.: Lectures on tensor categories. IRMA Lect. in Math. Theor. Phys. 12, 1–38 (2008)

    MathSciNet  Google Scholar 

  7. Chen X.-W., Huang H.-L., Ye Y., Zhang P.: Monomial Hopf algebras. J. Algebra 275, 212–232 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  8. Chen X.-W., Huang H.-L., Zhang P.: Dual Gabriel theorem with applications. Sci. in China series A Math. 49(1), 9–26 (2006)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  9. Chin, W.: A brief introduction to coalgebra representation theory. Lecture Notes in Pure and Appl. Math. Vol. 237, New York: Dekker, 2004, pp. 109–131

  10. Cibils C.: A quiver quantum group. Commun. Math. Phys. 157, 459–477 (1993)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  11. Cibils C., Rosso M.: Algèbres des chemins quantiques. Adv. Math. 125, 171–199 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  12. Cibils C., Rosso M.: Hopf quivers. J. Algebra 254, 241–251 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  13. Drinfeld, V.G.: Quantum groups. Proceedings of the International Congress of Mathematicians, Vol. 1, 2 (Berkeley, Calif., 1986), Providence, RI: Amer. Math. Soc., 1987, pp. 798–820

  14. Drinfeld V.G.: Quasi-Hopf algebras. Leningrad Math. J. 1, 1419–1457 (1990)

    MathSciNet  Google Scholar 

  15. Etingof P., Gelaki S.: Finite dimensional quasi-Hopf algebras with radical of codimension 2. Math. Res. Lett. 11, 685–696 (2004)

    MATH  MathSciNet  Google Scholar 

  16. Etingof P., Gelaki S.: On radically graded finite-dimensional quasi-Hopf algebras. Mosc. Math. J. 5(2), 371–378 (2005)

    MATH  MathSciNet  Google Scholar 

  17. Etingof P., Gelaki S.: Liftings of graded quasi-Hopf algebras with radical of prime codimension. J. Pure Appl. Algebra 205(2), 310–322 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  18. Etingof P., Nikshych D., Ostrik V.: On fusion categories. Ann. of Math. 162, 581–642 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  19. Etingof P., Ostrik V.: Finite tensor categories. Moscow Math. J. 4, 627–654 (2004)

    MATH  MathSciNet  Google Scholar 

  20. Gaberdiel M.R.: An algebraic approach to logarithmic conformal field theory. Int. J. Mod. Phys. A 18(25), 4593–4638 (2003)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  21. Gaberdiel M.R., Kausch H.G.: A rational logarithmic conformal field theory. Phys. Lett. B 386, 131–137 (1996)

    Article  ADS  MathSciNet  Google Scholar 

  22. Gabriel P.: Unzerlegbare Darstellungen I. Manus. Math. 6, 71–103 (1972)

    Article  MATH  MathSciNet  Google Scholar 

  23. Gabriel, P.: Indecomposable representations II. In: Symposia Mathematica, Vol. XI (Convegno di Algebra Commutativa, INDAM, Rome, 1971), London: Academic Press, 1973, pp. 81–104

  24. Gabriel, P.: Finite representation type is open. In: Representations of Algebras, Lecture Notes in Math., Vol. 488, Berlin-Heidelberg-New York: Springer Verlag, 1975, pp. 132–155

  25. Gelaki S.: Basic quasi-Hopf algebras of dimension n 3. J. Pure Appl. Algebra 198(1–3), 165–174 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  26. Gerstenhaer M.: On the deformation of rings and algebras. Ann. of Math. 79(2), 59–103 (1964)

    Article  MathSciNet  Google Scholar 

  27. Gerstenhaber M., Schack S.D.: Bialgebra cohomology, deformations. and quantum groups. Proc. Nat. Acad. Sci. 87, 478–481 (1990)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  28. Green E.L.: Constructing quantum groups and Hopf algebras from coverings. J. Algebra 176, 12–33 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  29. Green E.L., Solberg Ø.: Basic Hopf algebras and quantum groups. Math. Z. 229, 45–76 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  30. Gurarie V.: Logarithmic operators in conformal field theory. Nucl. Phys. B 410, 535–549 (1993)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  31. Hausser, F., Nill, F.: Integral theory for quasi-Hopf algebras. http://arxiv.org/abs/math./9904164.v2 [math.QA], 1999

  32. Huang H.-L.: Quiver approaches to quasi-Hopf algebras. J. Math. Phys. 50(4), 043501-1–043501-9 (2009)

    Article  ADS  Google Scholar 

  33. Huang, H.-L.: From projective representations to quasi-quantum groups. http://arxiv.org/0903.1472.v1 [math.QA], 2009

  34. Huang H.-L., Chen H.-X., Zhang P.: Generalized Taft algebras. Algebra Colloq. 11(03), 313–320 (2004)

    MATH  MathSciNet  Google Scholar 

  35. Huang, H.-L., Liu, G.: On coquasitriangular pointed Majid algebras. http://arxiv.org/labs/1002.0518.v1 [math.QA], 2010

  36. Karpilovsky G.: Projecive Representations of Finite Groups. Marcel Dekker, New York (1985)

    Google Scholar 

  37. Kassel, C.: Quantum Groups. Graduate Texts in Math. 155, New York: Springer-Verlag, 1995

  38. Liu G.: Classification of finite dimensional basic Hopf algebras according to their representation type. Contemp. Math. 478, 103–124 (2009)

    Article  Google Scholar 

  39. Liu G., Li F.: Pointed Hopf algebras of finite corepresentation type and their classifications. Proceedings of AMS 135(3), 649–657 (2007)

    Article  MATH  Google Scholar 

  40. Lusztig G.: Finite dimensional Hopf algebras arising from quantized universal enveloping algebras. J. AMS 3, 257–296 (1990)

    MATH  MathSciNet  Google Scholar 

  41. Majid S.: Representations, duals and quantum doubles of monoidal categories. Rend. Circ. Mat. Palermo (2) Suppl. No. 26, 197–206 (1991)

    MathSciNet  Google Scholar 

  42. Majid S.: Foundations of Quantum Group Theory. Cambridge University Press, Cambridge (1995)

    Book  MATH  Google Scholar 

  43. Majid S.: Quantum double for quasi-Hopf algebras. Lett. Math. Phys. 45(1), 1–9 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  44. Rosso M.: Quantum groups and quantum shuffles. Invent. Math. 133, 399–416 (1998)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  45. Shnider, S., Sternberg, S.: Quantum Groups: From Coalgebras to Drinfeld Algebras. Boston, MA: International Press Inc., 1993

  46. Van Oystaeyen F., Zhang P.: Quiver Hopf algebras. J. Algebra 280(2), 577–589 (2004)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics, Shandong University, Jinan, Shandong, 250100, China

    Hua-Lin Huang

  2. Department of Mathematics, Nanjing University, Nanjing, Jiangsu, 210093, China

    Gongxiang Liu

  3. Department of Mathematics, University of Science and Technology of China, Hefei, Anhui, 230026, China

    Yu Ye

Authors
  1. Hua-Lin Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Gongxiang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yu Ye
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Gongxiang Liu.

Additional information

Communicated by A. Connes

Rights and permissions

Reprints and permissions

About this article

Cite this article

Huang, HL., Liu, G. & Ye, Y. Quivers, Quasi-Quantum Groups and Finite Tensor Categories. Commun. Math. Phys. 303, 595–612 (2011). https://doi.org/10.1007/s00220-011-1229-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-011-1229-6

Keywords

Search

Navigation