Fractional Moment Bounds and Disorder Relevance for Pinning Models

Abstract

We study the critical point of directed pinning/wetting models with quenched disorder. The distribution K(·) of the location of the first contact of the (free) polymer with the defect line is assumed to be of the form K(n) = n ^−α-1 L(n), with α ≥ 0 and L(·) slowly varying. The model undergoes a (de)-localization phase transition: the free energy (per unit length) is zero in the delocalized phase and positive in the localized phase. For α < 1/2 disorder is irrelevant: quenched and annealed critical points coincide for small disorder, as well as quenched and annealed critical exponents [3,28]. The same has been proven also for α = 1/2, but under the assumption that L(·) diverges sufficiently fast at infinity, a hypothesis that is not satisfied in the (1 + 1)-dimensional wetting model considered in [12,17], where L(·) is asymptotically constant. Here we prove that, if 1/2 < α < 1 or α > 1, then quenched and annealed critical points differ whenever disorder is present, and we give the scaling form of their difference for small disorder. In agreement with the so-called Harris criterion, disorder is therefore relevant in this case. In the marginal case α = 1/2, under the assumption that L(·) vanishes sufficiently fast at infinity, we prove that the difference between quenched and annealed critical points, which is smaller than any power of the disorder strength, is positive: disorder is marginally relevant. Again, the case considered in [12,17] is out of our analysis and remains open.

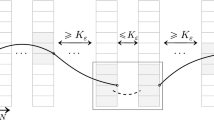
The results are achieved by setting the parameters of the model so that the annealed system is localized, but close to criticality, and by first considering a quenched system of size that does not exceed the correlation length of the annealed model. In such a regime we can show that the expectation of the partition function raised to a suitably chosen power ${\gamma \in (0, 1)}$ is small. We then exploit such an information to prove that the expectation of the same fractional power of the partition function goes to zero with the size of the system, a fact that immediately entails that the quenched system is delocalized.