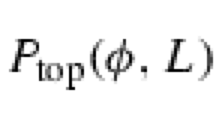Skip to main content
SpringerLink
Log in

Equilibrium States for Interval Maps: Potentials with sup φ − inf φ < h top (f)

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

An Erratum to this article was published on 14 April 2011

Abstract

We study an inducing scheme approach for smooth interval maps to prove existence and uniqueness of equilibrium states for potentials φ with the ‘bounded range’ condition sup φ − inf φ < h top (f), first used by Hofbauer and Keller [HK]. We compare our results to Hofbauer and Keller’s use of Perron-Frobenius operators. We demonstrate that this ‘bounded range’ condition on the potential is important even if the potential is Hölder continuous. We also prove analyticity of the pressure in this context.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abramov L.M.: The entropy of a derived automorphism. Dokl. Akad. Nauk SSSR 128, 647–650 (1959)

    MATH  MathSciNet  Google Scholar 

  2. Baladi, V.: Positive transfer operators and decay of correlations. Advanced Series in Nonlinear Dynamics 16, River Edge, NJ: World Scientific Publishing Co., Inc., 2000

  3. Baladi V., Keller G.: Zeta functions and transfer operators for piecewise monotone transformations. Math. Phys. 127, 459–477 (1990)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  4. Block, L., Guckenheimer, J., Misiurewicz, M., Young, L.-S.: Periodic points and topological entropy of one-dimensional maps, Lecture Notes in Math. 819, Berlin: Springer Verlag, 1980, pp. 18–34

  5. Blokh, A.: Decomposition of dynamical systems on an interval. (Russian) Usp. Mat. Nauk 38, 179–180 (1983); English translation: Russ. Math. Surve. 38, 133–134 (1983)

  6. Bowen, R.: Equilibrium states and the ergodic theory of Anosov diffeomorphisms. Lecture Notes in Math. 470, Berlin: Springer, 1975, pp. 51–58

  7. Brucks, K., Bruin, H.: Topics from one–dimensional dynamics. London Mathematical Society, Student Texts 62, Cambridge: Cambridge University Press, 2004

  8. Bruin H.: Induced maps, Markov extensions and invariant measures in one–dimensional dynamics. Commun. Math. Phys. 168, 571–580 (1995)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  9. Bruin H.: Topological conditions for the existence of absorbing Cantor sets. Trans. Amer. Math. Soc. 350, 2229–2263 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  10. Bruin H., Keller G.: Equilibrium states for S-unimodal maps. Ergod. Theory Dyn. Sys. 18, 765–789 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  11. Bruin H., Rivera-Letelier J., Shen W., van Strien S.: Large derivatives, backward contraction and invariant densities for interval maps. Invent. Math. 172(3), 509–533 (2008)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  12. Bruin, H., Todd, M.: Equilibrium states for interval maps: the potential  − t log |Df|. http://arXiv.org/abs/0704.2199v.[math.DS], 2007

  13. de Bruyn N.G.: Asymptotic methods in analysis. Dover Publ, New York (1981)

    Google Scholar 

  14. Buzzi J.: Specification on the interval. Trans. Amer. Math. Soc. 349, 2737–2754 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  15. Buzzi J.: Markov extensions for multi-dimensional dynamical systems. Israel J. Math. 112, 357–380 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  16. Buzzi J., Sarig O.: Uniqueness of equilibrium measures for countable Markov shifts and multidimensional piecewise expanding maps. Ergod. Theory Dyn. Sys. 23, 1383–1400 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  17. Denker M., Keller G., Urbański M.: On the uniqueness of equilibrium states for piecewise monotone mappings. Studia Math. 97, 27–36 (1990)

    MATH  MathSciNet  Google Scholar 

  18. Denker M., Urbański M.: Ergodic theory of equilibrium states for rational maps. Nonlinearity 4, 103–134 (1991)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  19. Downarowicz T., Serafin J.: Fiber entropy and conditional variational principles in compact non–metrizable spaces. Fund. Math. 172, 217–247 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  20. Fiebig D., Fiebig U.-R., Yuri M.: Pressure and equilibrium states for countable state Markov shifts. Israel J. Math. 131, 221–257 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  21. Hofbauer F.: Examples for the nonuniqueness of the equilibrium state. Trans. Amer. Math. Soc. 228, 223–241 (1977)

    Article  MATH  MathSciNet  Google Scholar 

  22. Hofbauer F.: Piecewise invertible dynamical systems. Probab. Theory Relat. Fields 72(3), 359–386 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  23. Hofbauer F., Keller G.: Equilibrium states for piecewise monotonic transformations. Ergod. Theory Dyn. Sys. 2, 23–43 (1982)

    MATH  MathSciNet  Google Scholar 

  24. Keller G.: Lifting measures to Markov extensions. Monatsh. Math. 108, 183–200 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  25. Keller, G.: Equilibrium states in ergodic theory. London Mathematical Society Student Texts 42, Cambridge: Cambridge University Press, 1998

  26. Melbourne I., Nicol M.: Almost sure invariance principle for nonuniformly hyperbolic systems. Commun. Math. Phys. 260, 131–146 (2005)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  27. Melbourne, I., Nicol, M.: Large deviations in nonuniformly hyperbolic dynamical systems. to appear in Trans. Amer. Math. Soc., doi:S0002-9947(08)04520-0, June 4, 2008

  28. Misiurewicz M., Szlenk W.: Entropy of piecewise monotone mappings. Studia Math. 67, 45–63 (1980)

    MATH  MathSciNet  Google Scholar 

  29. Oliveira K.: Equilibrium states for non-uniformly expanding maps. Ergod. Theory Dyn. Sys. 23, 1891–1905 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  30. Paccaut F.: Statistics of return times for weighted maps of the interval. Ann. Inst. H. Poincaré Probab. Statist. 36, 339–366 (2000)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  31. Pesin, Y., Senti, S.: Thermodynamical formalism associated with inducing schemes for one–dimensional maps. Moscow J. Math. 5, 3 669–678, 743–744 (2005)

    Google Scholar 

  32. Pesin Y., Zhang K.: Phase transitions for uniformly expanding maps. J. Stat. Phys. 122, 1095–1110 (2006)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  33. Przytycki F.: Lyapunov characteristic exponents are nonnegative. Proc. Amer. Math. Soc. 119, 309–317 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  34. Ruelle D.: An inequality for the entropy of differentiable maps. Bol. Soc. Brasil. Mat. 9, 83–87 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  35. Ruelle D.: Thermodynamic formalism. Reading MA, Addison Wesley (1978)

    MATH  Google Scholar 

  36. Sarig, O.: Thermodynamic formalism for Markov shifts. PhD. thesis, Tel–Aviv, 2000

  37. Sarig O.: Phase transitions for countable Markov shifts. Commun. Math. Phys. 217, 555–577 (2001)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  38. Sarig O.: Existence of Gibbs measures for countable Markov shifts. Proc. Amer. Math. Soc. 131, 1751–1758 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  39. Sinai, Y.: Gibbs measures in ergodic theory, (Russian). Usp. Mat. Nauk 27, 21–64 (1972); English translation: Russ. Math. Surv. 27, 21–69 (1972)

  40. Walters, P.: Some results on the classification of non-invertible measure preserving transformations. Lecture Notes in Math. 318, Berlin: Springer, 1973, pp. 266–276

  41. Young L.-S.: Recurrence times and rates of mixing. Israel J. Math. 110, 153–188 (1999)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Surrey, Guildford, Surrey, GU2 7XH, UK

    Henk Bruin & Mike Todd

  2. Departamento de Matemática Pura, Faculdade de Ciências da Universidade do Porto, Rua do Campo Alegre, 687, 4169-007, Porto, Portugal

    Mike Todd

Authors
  1. Henk Bruin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mike Todd
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Henk Bruin.

Additional information

Communicated by G. Gallavotti

This research was supported by EPSRC grant GR/S91147/01. MT was partially supported by FCT grant SFRH/BPD/26521/2006 and CMUP.

An erratum to this article is available at http://dx.doi.org/10.1007/s00220-011-1241-x.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bruin, H., Todd, M. Equilibrium States for Interval Maps: Potentials with sup φ − inf φ < h top (f). Commun. Math. Phys. 283, 579–611 (2008). https://doi.org/10.1007/s00220-008-0596-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-008-0596-0

Keywords

Search

Navigation