Skip to main content
SpringerLink
Log in

Symmetry Breaking in Laughlin’s State on a Cylinder

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

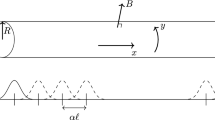
We investigate Laughlin’s fractional quantum Hall effect wave function on a cylinder. We show that it displays translational symmetry breaking in the axial direction for sufficiently thin cylinders. At filling factor 1/p, the period is p times the period of the filled lowest Landau level. The proof uses a connection with one-dimensional polymer systems and discrete renewal equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Akkermans E., Avron J.E., Narevich R., Seiler R.: Boundary Conditions for Bulk and Edge States in Quantum Hall Systems. Eur. Phys. J. B 1, 117–121 (1998)

    Article  ADS  Google Scholar 

  2. Aizenman M., Goldstein S., Lebowitz J.L.: Bounded Fluctuations and Translation Symmetry Breaking in One-Dimensional Particle Systems. J. Stat. Phys. 103, 601–618 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  3. Aizenman M., Martin P.A.: Structure of Gibbs States of one Dimensional Coulomb Systems. Commun. Math. Phys. 78, 99–116 (1980)

    Article  ADS  MathSciNet  Google Scholar 

  4. Bergholtz, E.J., Karlhede, A.: One-dimensional theory of the Quantum Hall systems. J. Stat. Mech. L04001 (2006); Quantum Hall system in the Tao-Thouless limit, Phys. Rev. B 77, 55308 (2008)

    Google Scholar 

  5. Brascamp, H.J., Lieb, E.H.: Some inequalities for Gaussian measures and the long-range order of the one-dimensional plasma. In: Functional Integration and its Applications. A.M. Arthurs, ed., Oxford: Clarendon Press, 1975, pp. 1–14

  6. Bratteli O., Robinson D.W.: Operator Algebras and Quantum Statistical Mechanics. Vol. 1. Berlin-Heidelberg-New York, Springer -Verlag (1979)

    Google Scholar 

  7. Choquard P., Forrester P.J., Smith E.R.: The two-dimensional one-component plasma at Γ  = 2: the semiperiodic strip. J. Stat. Phys. 33, 13–22 (1983)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  8. Dunne G.V.: Slater Decomposition of Laughlin States. Int. J. Mod. Phys. B 7, 4783–4813 (1993)

    Article  ADS  MathSciNet  Google Scholar 

  9. Feller W.: An introduction to probability theory and its applications. 2 ed., Vol. 1. John Wiley & Sons, New York (1962)

    Google Scholar 

  10. Di Francesco P., Gaudin M., Itzykson C., Lesage F.: Laughlin’s wave function, Coulomb gases and expansions of the discriminant. Int. J. Mod. Phys. A 9, 4287–4352 (1994)

    ADS  MathSciNet  Google Scholar 

  11. Forrester P.J.: Finite-Size Corrections to the Free Energy of Coulomb Systems with a Periodic Boundary Condition. J. Stat. Phys. 63, 491–504 (1991)

    Article  ADS  MathSciNet  Google Scholar 

  12. Gruber C., Kunz H.: General properties of polymer systems. Commun. Math. Phys. 22, 133–161 (1971)

    Article  ADS  MathSciNet  Google Scholar 

  13. Heilmann O.J., Lieb E.H.: Theory of Monomer-Dimer Systems. Commun. Math. Phys. 25, 190–232 (1972)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  14. Hardy G.H., Littlewood J.E., Pólya G.: Inequalities. Cambridge University Press, Cambridge (1964)

    Google Scholar 

  15. Haldane F.D.M., Rezayi E.H.: Periodic Laughlin-Jastrow wave functions for the fractional quantized Hall effect. Phys. Rev. B 31, 2529–2531 (1985)

    Article  ADS  Google Scholar 

  16. Ioffe D., Velenik Y., Zahradnik M.: Entropy-Driven Phase Transition in a Polydisperse Hard-Rods Lattice System. J. Stat. Phys. 122, 761–786 (2006)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  17. Jancovici B., Lebowitz J.L.: Bounded Fluctuations and Translation Symmetry Breaking: a Solvable Model. J. Stat. Phys. 103, 619–624 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  18. Jansen S., Lieb E.H., Seiler R.: Laughlin’s function on a cylinder: plasma analogy and representation as a quantum polymer. Phys. Stat. Sol. (b) 245, 439–446 (2008)

    Article  Google Scholar 

  19. King R.C., Toumazet F., Wybourne B.G.: The square of the Vandermonde determinant and its q-generalisation. J. Phys. A 37, 737–767 (2001)

    MathSciNet  Google Scholar 

  20. Kunz H.: The One-Dimensional Classical Electron Gas. Ann. Phys. 85, 303–335 (1974)

    Article  ADS  MathSciNet  Google Scholar 

  21. Laughlin R.B.: Quantized Hall conductivity in two dimensions. Phys. Rev. B 23, 5632–5633 (1981)

    Article  ADS  Google Scholar 

  22. Laughlin R.B.: Anomalous Quantum Hall Effect: An Incompressible Quantum Fluid with Fractionally Charged Excitations. Phys. Rev. Lett. 50, 1395–1398 (1983)

    Article  ADS  Google Scholar 

  23. Lenard A.: Exact Statistical Mechanics of a One-Dimensional System with Coulomb Forces. J. Math. Phys. 2, 682–693 (1961)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  24. Lebowitz J.L., Lieb E.H.: Existence of Thermodynamics for Real Matter with Coulomb Forces. Phys. Rev. Lett. 22, 631–634 (1969)

    Article  ADS  Google Scholar 

  25. Lee D.-H., Leinaas J.M.: Mott Insulators without Symmetry Breaking. Phys. Rev. Lett. 92, 096401 (2004)

    Article  ADS  Google Scholar 

  26. Rezayi E.H., Haldane F.D.M.: Laughlin state on stretched and squeezed cylinders and edge excitations in the quantum Hall effect. Phys. Rev. B 50, 17199–17201 (1994)

    Article  ADS  Google Scholar 

  27. Seidel A., Fu H., Lee D.-H., Leinaas J.M., Moore J.: Incompressible Quantum Liquids and New Conservation Laws. Phys. Rev. Lett 95, 266405 (2005)

    Article  ADS  Google Scholar 

  28. Šamaj L., Wagner J., Kalinay P.: Translation Symmetry Breaking in the One-Component Plasma on the Cylinder. J. Stat. Phys. 117, 159–178 (2004)

    Article  MATH  ADS  Google Scholar 

  29. Thouless D.J.: Theory of the quantized Hall effect. Surf. Sci. 142, 147–154 (1984)

    Article  ADS  Google Scholar 

  30. Tsui D.C., Störmer H.L., Gossard A.C.: Two-Dimensional Magnetotransport in the Extreme Quantum Limit. Phys. Rev. Lett. 48, 1559–1562 (1982)

    Article  ADS  Google Scholar 

  31. Tao R., Thouless D.J.: Fractional quantization of Hall conductance. Phys. Rev. B 28, 1142–1144 (1983)

    Article  ADS  Google Scholar 

  32. Tao R., Wu Y.-S.: Gauge invariance and fractional quantum Hall effect. Phys. Rev. B 30, 1097–1098 (1984)

    Article  ADS  Google Scholar 

  33. Westerberg E., Hansson T.H.: Quantum mechanics on thin cylinders. Phys. Rev. B 47, 16554–16562 (1993)

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Mathematik, Technische Universität Berlin, Str. des 17. Juni 136, 10623, Berlin, Germany

    S. Jansen & R. Seiler

  2. Department of Mathematics, Princeton University, P. O. Box 708, Princeton, NJ, 08544, USA

    E. H. Lieb

Authors
  1. S. Jansen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. E. H. Lieb
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. R. Seiler
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Jansen.

Additional information

Communicated by H. Spohn

© 2008 by the Authors. This paper may be reproduced, in its entirety, for non-commercial purposes.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Jansen, S., Lieb, E.H. & Seiler, R. Symmetry Breaking in Laughlin’s State on a Cylinder. Commun. Math. Phys. 285, 503–535 (2009). https://doi.org/10.1007/s00220-008-0576-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-008-0576-4

Keywords

Search

Navigation