Skip to main content
SpringerLink
Log in

Integrable Nonlinear Evolution Equations on a Finite Interval

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

Let q(x,t) satisfy an integrable nonlinear evolution PDE on the interval 0<x<L, and let the order of the highest x-derivative be n. For a problem to be at least linearly well-posed one must prescribe N boundary conditions at x=0 and n−N boundary conditions at x=L, where if n is even, N=n/2, and if n is odd, N is either (n−1)/2 or (n+1)/2, depending on the sign of ∂ n x q. For example, for the sine-Gordon (sG) equation one must prescribe one boundary condition at each end, while for the modified Korteweg-de Vries (mKdV) equations involving q t +q xxx and q t −q xxx one must prescribe one and two boundary conditions, respectively, at x=0. We will refer to these two mKdV equations as mKdV-I and mKdV-II, respectively.

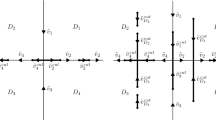
Here we analyze the Dirichlet problem for the sG equation, as well as typical boundary value problems for the mKdV-I and mKdV-II equations. We first show that the unknown boundary values at each end (for example, q x (0,t) and q x (L,t) in the case of the Dirichlet problem for the sG equation) can be expressed in terms of the given initial and boundary conditions through a system of four nonlinear ODEs. We then show that q(x,t) can be expressed in terms of the solution of a 2×2 matrix Riemann-Hilbert problem formulated in the complex k-plane. This problem has explicit (x,t) dependence in the form of an exponential; for example, for the case of the sG this exponential is exp {i(k−1/k)x+i(k+1/k)t}. Furthermore, the relevant jump matrices are explicitly given in terms of the spectral functions {a(k),b(k)}, {A(k),B(k)}, and , which in turn are defined in terms of the initial conditions, of the boundary values of q and of its x-derivatives at x=0, and of the boundary values of q and of its x-derivatives at x=L, respectively. This Riemann-Hilbert problem has a global solution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adler, V.E., Gürel, B., Gürses, M., Habibullin, I.: Boundary conditions for integrable equations. J. Phys. A 30, 3505–3513 (1997)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  2. Boutet de Monvel, A., Fokas, A.S., Shepelsky, D.: Analysis of the global relation for the nonlinear Schrödinger equation on the half-line. Lett. Math. Phys. 65, 199–212 (2003)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  3. Boutet de Monvel, A., Fokas, A.S., Shepelsky, D.: The mKdV equation on the half-line. J. Inst. Math. Jussieu 3(2), 139–164 (2004)

    Article  MathSciNet  Google Scholar 

  4. Boutet de Monvel, A., Kotlyarov, V.: Generation of asymptotic solitons of the nonlinear Schrödinger equation by boundary data. J. Math. Phys. 44(8), 3185–3215 (2003)

    Article  Google Scholar 

  5. Boutet de Monvel, A., Shepelsky, D.: The modified KdV equation on a finite interval. C. R. Math. Acad. Sci. Paris 337(8), 517–522 (2003)

    Google Scholar 

  6. Boutet de Monvel, A., Shepelsky, D.: Initial boundary value problem for the mKdV equation on a finite interval. Ann. Inst. Fourier (Grenoble) 54(5), 1477–1495 (2004)

    Google Scholar 

  7. Deift, P., Venakides, S., Zhou, X.: New results in small dispersion KdV by an extension of the steepest descent method for Riemann-Hilbert problems. Intl. Math. Res. Notices 1997, No. 6, pp. 286–299

  8. Degasperis, A., Manakov, S.V., Santini, P.M.: On the initial-boundary value problems for soliton equations. JETP Letters 74(10), 481–485 (2001)

    Google Scholar 

  9. Degasperis, A., Manakov, S.V., Santini, P.M.: Initial-boundary problems for linear and soliton PDEs. Theoret. and Math. Phys. 133(2), 1475–1489 (2002)

    Article  MathSciNet  Google Scholar 

  10. Degasperis, A., Manakov, S.V., Santini, P.M.: Integrable and nonintegrable initial boundary value problems for soliton equations. J. Nonlinear Math. Phys. 12, 228–243 (2005)

    MathSciNet  Google Scholar 

  11. Deift, P., Zhou, X.: A steepest descent method for oscillatory Riemann-Hilbert problems. Asymptotics for the MKdV equation. Ann. Math. (2) 137, 295–368 (1993)

    Google Scholar 

  12. Fokas, A.S.: A unified transform method for solving linear and certain nonlinear PDEs. Proc. Roy. Soc. London Ser. A 453, 1411–1443 (1997)

    ADS  MATH  MathSciNet  Google Scholar 

  13. Fokas, A.S.: On the integrability of linear and nonlinear partial differential equations. J. Math. Phys. 41, 4188–4237 (2000)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  14. Fokas, A.S.: Two dimensional linear PDEs in a convex polygon. Proc. Roy. Soc. London Ser. A 457, 371–393 (2001)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  15. Fokas, A.S.: Integrable nonlinear evolution equations on the half-line. Commun. Math. Phys. 230, 1–39 (2002)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  16. Fokas, A.S.: The generalized Dirichlet-to-Neumann map for certain nonlinear evolution PDEs. Commun. Pure Appl. Math. 58, 639–670 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  17. Fokas, A.S., Its, A.R.: An initial-boundary value problem for the sine-Gordon equation. Theor. Math. Physics 92, 388–403 (1992)

    MathSciNet  Google Scholar 

  18. Fokas, A.S., Its, A.R.: An initial-boundary value problem for the Korteweg-de Vries equation. Math. Comput. Simul. 37, 293–321 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  19. Fokas, A.S., Its, A.R.: The linearization of the initial-boundary value problem of the nonlinear Schrödinger equation. SIAM J. Math. Anal. 27, 738–764 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  20. Fokas, A.S., Its, A.R.: The nonlinear Schrödinger equation on the interval. J. Phys. A 37, 6091–6114 (2004)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  21. Fokas, A.S., Its, A.R., Sung, L.Y.: The nonlinear Schrödinger equation on the half-line. Nonlinearity 18, 1771–1822 (2005)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  22. Fokas, A.S., Pelloni, B.: A transform method for evolution PDEs on the interval. IMA J. Appl. Math. 70(4), 564–587(2005)

    Article  MathSciNet  Google Scholar 

  23. Gardner, C.S., Greene, J.M., Kruskal, M.D., Miura, R.M.: Methods for solving the Korteweg–de Vries equation. Phys. Rev. Lett. 19, 1095–1097 (1967)

    Article  ADS  MATH  Google Scholar 

  24. Grinevich, P.G., Santini, P.M.: The initial-boundary value problem on the interval for the nonlinear Schrödinger equation. The algebro-geometric approach. I. In: V.M. Buchstaber, I.M.Krichever, (eds.), Geometry, Topology, and Mathematical Physics: S.P. Novikov Seminar 2001-2003, Volume 212 of AMS Translations Ser. 2, Providence, R.I.: Amer. Math. Soc., 2004, pp. 157–178

  25. Habibullin, I.T.: Bäcklund transformation and integrable boundary-initial value problems. In: Nonlinear world, Volume 1 (Kiev, 1989), River Edge, N.J.: World Sci. Publishing, 1990, pp. 130–138

  26. Kamvissis, S.: Semiclassical nonlinear Schrödinger on the half line. J. Math. Phys. 44, 5849–5868 (2003)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  27. Lax, P.D.: Integrals of nonlinear equations of evolution and solitary waves. Commun. Pure Appl. Math. 21, 467–490 (1968)

    MATH  MathSciNet  Google Scholar 

  28. Sklyanin, E.K.: Boundary conditions for integrable equations. Funct. Anal. Appl. 21, 86–87 (1987)

    Article  MATH  MathSciNet  Google Scholar 

  29. Tarasov, V.O.: An boundary value problem for the nonlinear Schrödinger equation. Zap. Nauchn. Sem. LOMI 169, 151–165 (1988); [transl.: J. Soviet Math. 54, 958–967 (1991)]

    MATH  Google Scholar 

  30. Zakharov, V.E., Shabat, A.B.: A scheme for integrating the nonlinear equations of mathematical physics by the method of the inverse scattering problem. I and II. Funct. Anal. Appl. 8, 226–235 (1974) and 13, 166–174(1979)

    Article  MATH  Google Scholar 

  31. Zhou, X.: The Riemann-Hilbert problem and inverse scattering. SIAM J. Math. Anal. 20, 966–986 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  32. Zhou, X.: Inverse scattering transform for systems with rational spectral dependence. J. Differ. Eqs. 115, 277–303 (1995)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut de Mathématiques de Jussieu, case 7012, Université Paris 7, 2 place Jussieu, 75251, Paris, France

    Anne Boutet de Monvel

  2. Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Cambridge, CB3 0WA, U.K

    Athanassis S. Fokas

  3. Mathematical Division, Institute for Low Temperature Physics, 47 Lenin Avenue, 61103, Kharkiv, Ukraine

    Dmitry Shepelsky

Authors
  1. Anne Boutet de Monvel
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Athanassis S. Fokas
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dmitry Shepelsky
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Anne Boutet de Monvel.

Additional information

Communicated by P. Constantin

Rights and permissions

Reprints and permissions

About this article

Cite this article

Monvel, A., Fokas, A. & Shepelsky, D. Integrable Nonlinear Evolution Equations on a Finite Interval. Commun. Math. Phys. 263, 133–172 (2006). https://doi.org/10.1007/s00220-005-1495-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-005-1495-2

Keywords

Search

Navigation