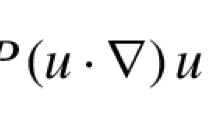Abstract
Consider the stationary Navier–Stokes equations in a bounded domain \({\Omega \subset \mathbb{R}^n}\) whose boundary \({\partial\Omega}\) consists of L + 1 smooth (n − 1)-dimensional closed hypersurfaces Γ0, Γ1, . . . , Γ L , where Γ1, . . . , Γ L lie inside of Γ0 and outside of one another. The Leray inequality of the given boundary data β on \({\partial\Omega}\) plays an important role for the existence of solutions. It is known that if the flux \({\gamma_i \equiv \int_{\Gamma_i}\beta \cdot \nu dS = 0}\) on Γ i (ν: the unit outer normal to Γ i ) is zero for each i = 0, 1, . . . , L, then the Leray inequality holds. We prove that if there exists a sphere S in Ω separating \({\partial\Omega}\) in such a way that Γ1, . . . , Γ k (1 ≦ k ≦ L) are contained inside of S and that the others Γ k+1, . . . , Γ L are outside of S, then the Leray inequality necessarily implies that γ 1 + · · · + γ k = 0. In particular, suppose that there are L spheres S 1, . . . , S L in Ω lying outside of one another such that Γ i lies inside of S i for all i = 1, . . . , L. Then the Leray inequality holds if and only if γ 0 = γ 1 = · · · = γ L = 0.
Similar content being viewed by others
References
Amick C.J.: Existence of solutions to the nonhomogeneous steady Navier–Stokes equations. Indiana Univ. Math. J. 33, 817–830 (1984)
Borchers W., Sohr H.: On the equations rot v = g and div u = f with zero boundary conditions. Hokkaido Math. J. 19, 67–87 (1990)
Fujita H.: On the existence and regularity of the steady-state solutions of the Navier–Stokes equations. J. Fac. Sci. Univ. Tokyo Sec.I. A. 9, 59–102 (1960)
Fujita, H.: On stationary solutions to Navier–Stokes equation in symmetric plane domains under general outflow condition. In: Navier–Stokes equations: theory and numerical methods, Varenna 1997 Pitman Res. Notes Math. Ser., vol. 388, pp. 16–30. Longman, Harlow, 1998.
Galdi G.P.: An Introduction to the Mathematical Theory of the Navier–Stokes Equations. vol. II. Springer-Verlag, New York (1994)
Kobayashi., T.: Takeshita’s examples for Leray’s inequality (Preprint)
Kozono H., Yanagisawa T.: L r-variational inequality for vector fields and the Helmholtz–Weyl decomposition in bounded domains. Indiana Univ. Math. J. 58, 1853–1920 (2009)
Kozono H., Yanagisawa T.: Leray’s problem on the stationary Navier–Stokes equations with inhomogeneous boundary data. Math. Z. 262, 27–39 (2009)
Ladyzhenskaya O.A.: The mathematical theory of viscous incompressible flow. Gordon and Breach, London (1969)
Leray J.: Etude de diverses équations intégrales non linéaires et de quelques problèmes que pose l’hydrodynamique. J. Math. Pures Appl. 12, 1–82 (1933)
Morimoto, H.: General outflow condition for Navier–Stokes flow. In: Kozono, H., Shibata, Y. (ed.) Recent topics in mathematical theory of viscous incompressible fluids, Tsukuba 1996. Lect. Notes Num. Appl., vol. 16, pp. 209–224. Kinokuniya, Tokyo (1998)
Takeshita A.: A remark on Leray’s inequality. Pacific J. Math. 157, 151–158 (1993)
Temam R.: Navier–Stokes equations. Theory and Numerical Analysis. North-Holland Pub. Co., Amsterdam (1979)
Ziemer W.P.: Weakly differentiable functions. Springer-Verlag, New York (1989)
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Professor Izumi Takagi on the occasion of his 60th birthday.
Rights and permissions
About this article
Cite this article
Farwig, R., Kozono, H. & Yanagisawa, T. Leray’s inequality in general multi-connected domains in \({\mathbb{R}^n}\) . Math. Ann. 354, 137–145 (2012). https://doi.org/10.1007/s00208-011-0716-6
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00208-011-0716-6