A note on simultaneous Diophantine approximation on planar curves

Abstract

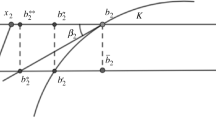
Let $\mathcal{S}_{n}(\psi_{1},\dots,\psi_{n})$ denote the set of simultaneously $(\psi_{1},\dots,\psi_{n})$- approximable points in $\mathbb{R}^{n}$ and $\mathcal{S}^{*}_{n}(\psi)$ denote the set of multiplicatively ψ-approximable points in $\mathbb{R}^{n}$. Let $\mathcal{M}$ be a manifold in $\mathbb{R}^{n}$. The aim is to develop a metric theory for the sets $\mathcal{M} \cap \mathcal{S}_{n}(\psi_1,\dots,\psi_n)$ and $\mathcal{M} \cap \mathcal{S}^{*}_{n}(\psi)$ analogous to the classical theory in which $\mathcal{M}$ is simply $\mathbb{R}^{n}$. In this note, we mainly restrict our attention to the case that $\mathcal{M}$ is a planar curve $\mathcal{C}$. A complete Hausdorff dimension theory is established for the sets $\mathcal{C} \cap \mathcal{S}_{2}(\psi_{1},\psi_{2})$ and $\mathcal{C} \cap \mathcal{S}^{*}_{2}(\psi)$. A divergent Khintchine type result is obtained for $\mathcal{C} \cap \mathcal{S}_{2}(\psi_1,\psi_2)$; i.e. if a certain sum diverges then the one-dimensional Lebesgue measure on $\mathcal{C}$ of $\mathcal{C} \cap \mathcal{S}_{2}(\psi_1,\psi_2)$ is full. Furthermore, in the case that $\mathcal{C}$ is a rational quadric the convergent Khintchine type result is obtained for both types of approximation. Our results for $\mathcal{C} \cap \mathcal{S}_{2}(\psi_1,\psi_2)$ naturally generalize the dimension and Lebesgue measure statements of Beresnevich et al. (Mem AMS, 179 (846), 1–91 (2006)). Moreover, within the multiplicative framework, our results for $\mathcal{C} \cap \mathcal{S}^{*}_{2}(\psi)$ constitute the first of their type.