Skip to main content
SpringerLink
Log in

Steady flows of compressible fluids in a rigid container with upper free boundary

  • Published:
Mathematische Annalen Aims and scope Submit manuscript

Abstract.

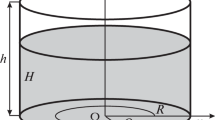
We consider fluid in a smooth rigid container whose lateral boundary is a piece of vertical cylinder, bounded above by a free upper surface. As basic flow we consider the non homogeneous rest state in the presence of gravity, and of a surface tension. Under these assumptions, we study the existence of a steady free boundary Γ and a steady motion in Ω of an isothermal viscous gas, resulting as perturbation to the rest state in correspondence of small non potential perturbations to the (large potential) gravitational force. We linearize the problem by prescribing the unknown domain Ω, then we make use of the iterative scheme introduced by Heywood and Padula. Our method is based on an iteration between the Neumann problem for a non homogeneous Stokes system for the velocity, the Neumann problem for an elliptic problem on Γ for height, and a steady transport equation for the perturbation to the density. The difference of boundary condition between lateral boundary and free upper surfaces causes a singularity at the intersection (contact line). To avoid singularities on the contact line, we adopt weighted Sobolev spaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bause, M., Heywood, J.G., Novotny, A., Padula, M.: Numerical approximation schemes for the Poisson-Stokes equations of steady compressible viscous flow 1. Math. Fluid Mech., Birkhauser-Verlag ed., 2001

  2. Bause, M., Heywood, J.G., Novotny, A., Padula, M.: Numerical approximation schemes for the Poisson-Stokes equations of steady compressible viscous flow 2. Math. Fluid Mech., Birkhauser-Verlag ed., 2001

  3. Beale, J.T.: Large time regularity of viscous surface waves. Arch. Ration. Mech. Anal. 84(4), 307–352 (1984)

    MATH  Google Scholar 

  4. Beiraó da Veiga, H.: Existence results in Sobolev spaces for a stationary transport equations. Ricerche Mat. Vol. in honor of C. Miranda, 1987

  5. Beiraó da Veiga, H.: An L p-theory for the n-dimensional, stationary, compressible Navier-Stokes equations, and the incompressible limit for compressible fluids. The equilibrium solutions. Commun. Math. Phys. 109, 229–248 (1987)

    MATH  Google Scholar 

  6. Borchers, W., Pileckas, K.: Existence, uniqueness and asymptotics of steady jets. Arch. Rat. Mech. Anal. 120, 1–49 (1992)

    MathSciNet  MATH  Google Scholar 

  7. Choe, H.J., Jin, B.J.: Existence of solutions of stationary compressible Navier-Stokes equations with large force. Journal of Functional Analysis, 2000

  8. Finn, R.: Equilibrium capillary surfaces. Grundelehren der mathematischen Wissenschaften 284, Springer-Verlag, New-York, Berlin, Heidelberg, Tokyo, 1986

  9. Finn, R.: Equations of capillarity. JMFM 3, 139–151 (2001)

    MathSciNet  MATH  Google Scholar 

  10. Farwig, R.: Stationary solutions of the Navier-Stokes equations with slip boundary conditions. Comm. Partial Diff. Eqs. 14, 1579–1606 (1989)

    MATH  Google Scholar 

  11. Galdi, G.P.: An introduction to the mathematical theory of the Navier-Stokes equations. Vol. I, Springer-Verlag New York, 1994

  12. Gilbarg and Trudinger: Elliptic partial differential equations of second order. Springer-Verlag, Berlin and New York, 1983

  13. Grisvard, P.: Elliptic problems in nonsmooth domains. Boston, London, Melbourne, Pitman Pub. Co., 1985

  14. Kondratev, V.: Boundary value problems for elliptic equations in domains with conical or angular points. Trudy Moscow Math. Soc. 16, 227–313 (1967)

    MATH  Google Scholar 

  15. Massari, U., Miranda, M.: Minimal surfaces of codimension one. Math. Studies 91, ed. L. Nachbin North Holland, Amsterdam, New York, Oxford, 1984

  16. Jin, B.J., Padula, M.: On the existence of compressible viscous flow in a horizontal layer with free upper surface. To appear in Comm. Pure and Applied Analysis

  17. Heywood, J.G., Padula, M.: On the Existence and Uniqueness Theory for steady compressible Viscous flow, Fundamental directions in Mathematical Fluid Mechanics. Advances in Mathematical Fluid Mechanics, Birkhäuser Verlag, Basel-Boston-Berlin, 1999

  18. Matsumura, A., Nishida, T.: Initial boundary value problems for the equations of motions of compressible viscous and heat-conductive fluids. Comm. Math. Phys. 89, 445–464 (1983)

    MathSciNet  MATH  Google Scholar 

  19. Matsumura, A., Padula, M.: Stability of the stationary solutions of compressible Viscous fluids with large external potential forces. Stab. Appl. Anal. Cont. Media 2, 183–202 (1992)

    Google Scholar 

  20. Nazarov, S.A., Novotny, A., Pileckas, K.: On steady compressible Navier-Stokes equations in plane domain with corners. Math. Ann. 302, 121–150 (1996)

    MathSciNet  Google Scholar 

  21. Nazarov, S.A., Plamenevsky, B.A.: Elliptic problems in domains with piecewise smooth boundaries. De Gruyter expositions in Mathematics 13, Berlin, New-York, 1994

  22. Novotny, A.: About the steady transport equations. L p approach in domains with sufficiently smooth boundaries. Comment. Math. Univ. Carolin., 1996

  23. Novotny, A., Padula, M.: Existence and uniqueness of stationary solutions for viscous compressible heat conductive fluid with large potential and small nonpotential forces. Siberian Math. J. 34, 120–146 (1993)

    MathSciNet  MATH  Google Scholar 

  24. Novotny, A., Pileckas, K.: Steady compressible Navier-Stokes equations with large potential forces via a method of decomposition. Math. Meth. Appl. Sci. 21, 665–684 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  25. Padula, M.: Existence and uniqueness for viscous steady compressible motions. Arch. Rat. Mech. Anal. 97, 89–102 (1987)

    MathSciNet  MATH  Google Scholar 

  26. Padula, M., Solonnikov, V.A.: On Reyleigh-Taylor stability. Annali dell’Universita’ di Ferrara, vol. 45, 2000

  27. Padula, M.: On the uniqueness of viscous compressible steady flows. Trends in Appl. of Pure Math. to Mech. Vol. IV, ed. Brilla, Pitman, London, 1981, pp. 186–196

  28. Padula, M.: Steady flows of barotropic viscous fluids. Quaderni di Matematica 1, 253–345 (1997)

    MATH  Google Scholar 

  29. Pileckas, K., Zajaczkowski, W.M.: On the free boundary problem for a stationary compressible Navier-Stokes equations. Commun. Math. Phys. 129, 169–204 (1990)

    MathSciNet  MATH  Google Scholar 

  30. Sattinger, D.H.: On the free surface of a viscous fluid motion. Proc. R. Soc: London, A 349, 183–204 (1976)

    Google Scholar 

  31. Scadilov, Solonnikov, V.A.: Solvability of the initial boundary value problem for the equations of a viscous compressible fluid. J. Sov. Math 14, (1980)

  32. Solonnikov, V.A.: Solvability of a problem on the plane motion of a heavy viscous incompressible capillary liquid partially filling a container. Isv. Ahad. Nauk SSSR, ser. Mat. 43, (1979); Math. USSR Izvestija 14, 193–221 (1980)

  33. Solonnikov, V.A.: On the Stokes equation in domains with nonsmooth boundaries and on a viscous incompressible flow with a free surface. Nonlinear Part. Diff. Equations~3, College de France Seminar, 1980/1981, pp. 340–423

  34. Solonnikov, V.A.: Solvability of two stationary free boundary problems for the Navier-Stokes equations. Bollettino U.M.I. (8) 1-B, 283–342 (1998)

  35. Solonnikov, V.A.: Solvability of the initial boundary value problem for the equations of a viscous compressible fluid. J. Sov. Math 14 (1980)

  36. Solonnikov, V.A.: Free boundary problems for the Navier-Stokes equations with moving contact points. Proceedings of the Conference on Nonlinear Evolution Equations and Infinite-dimensional Dynamics Systems, 1995, pp. 180–189

  37. Solonnikov, V.A.: Free boundary problems for the Navier-Stokes equations with moving contact points. Free boundary problems: theory and applications, (Toledo, 1993). Pitman Res. Notes Math. Ser., 323. Longman Sci. Tech, Harlow, 1995, pp. 203–214

  38. Solonnikov, V.A.: Solvability of two-dimensional free boundary problem for the Navier-Stokes equations for limiting values of contact angle. Recent developments in partial differential equations, Quad. Mat,, 2. Arance, Rome, 1998, pp. 163–210

  39. Solonnikov, V.A.: On some free boundary problems for the Navier-Stokes equations with moving contact points and lines. Math. Ann. 302(4), 743–772 (1995)

    MATH  Google Scholar 

  40. Solonnikov, V.A., Tani, A.: Evolution free boundary problem for equations of motion of viscous compressible barotropic liquids. The Navier-Stokes equation II: Theory and numerical methods, Proceedings Oberwolfach, Lecture notes in Math., 1530, 1991, pp. 30–58

  41. Stein, E.M.: Singular integrals and differentiability properties of functions. Princeton Univ. press, 1970

  42. Tani, A.: On the free boundary value problem for compressible viscous fluid motion. J. Math. Kyoto. Univ. 839(859), 21–4 (1981)

    MATH  Google Scholar 

  43. Tani, A.: On the first initial-boundary value problem of compressible viscous fluid motion. Publ. RIMS. Kyoto Univ 13, 193–253 (1977)

    MATH  Google Scholar 

  44. Valli, A.: Periodic and stationary solutions for compressible Navier-Stokes equations by stability method. Ann. Sc. Norm. Super. pisa 607–647 (1984)

  45. Valli, A.: On the existence of stationary solutions for compressible Navier-Stokes equation. Ann. Inst. H. Poincaré 4, 99–113 (1987)

    MathSciNet  MATH  Google Scholar 

  46. Maxwell, J.C.: Capillary attraction. Encyclopedia Britannica, 9th ed., vol. 5, S.L. Hall, New York, 1878

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Seoul National University, Seoul, 151-747, Korea

    Bum Ja Jin

  2. Department of Mathematics, University of Ferrara, Via Machiavelli 35, 44100, Ferrara, Italy

    Mariarosaria Padula

Authors
  1. Bum Ja Jin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mariarosaria Padula
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mariarosaria Padula.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ja Jin, B., Padula, M. Steady flows of compressible fluids in a rigid container with upper free boundary. Math. Ann. 329, 723–770 (2004). https://doi.org/10.1007/s00208-004-0535-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00208-004-0535-0

Keywords

Search

Navigation