Abstract
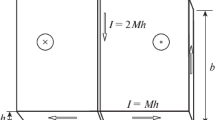
Charged domain walls are a type of domain wall in thin ferromagnetic films which appear due to global topological constraints. The non-dimensionalized micromagnetic energy for a uniaxial thin ferromagnetic film with in-plane magnetization \(m \in {\mathbb {S}}^1\) is given by
where M is an arbitrary fixed background field to ensure global neutrality of magnetic charges. We consider a material in the form a thin strip and enforce a charged domain wall by suitable boundary conditions on m. In the limit \(\varepsilon \rightarrow 0\) and for fixed \(\lambda > 0\), corresponding to the macroscopic limit, we show that the energy \(\Gamma \)-converges to a limit energy where jump discontinuities of the magnetization are penalized anisotropically. In particular, in the subcritical regime \(\lambda \leqq 1\), one-dimensional charged domain walls are favorable, in the supercritical regime \(\lambda > 1\), the limit model allows for zigzaging two-dimensional domain walls.





Similar content being viewed by others
References
Acerbi, E., Fusco, N., Morini, M.: Minimality via second variation for a nonlocal isoperimetric problem. Comm. Math. Phys. 322(2), 515–557, 2013
Alberti, G., Bellettini, G.: A non-local anisotropic model for phase transitions: asymptotic behaviour of rescaled energies. Eur. J. Appl. Math. 9(3), 261–284, 1998
Alouges, F., Rivière, T., Serfaty, S.: Néel and cross-tie wall energies for planar micromagnetic configurations. ESAIM Control Optim. Calc. Var. 8, 31–68 (electronic), 2002. A tribute to J. L. Lions.
Ambrosio, L., Da Prato, G., Mennucci, A.: Introduction to Measure Theory and Integration, volume 10 of Appunti. Scuola Normale Superiore di Pisa (Nuova Serie). Edizioni della Normale, Pisa, 2011
Anzellotti, G., Baldo, S., Visintin, A.: Asymptotic behavior of the Landau–Lifshitz model of ferromagnetism. Appl. Math. Optim. 23(1), 171–192, 1991
Bonacini, M., Cristoferi, R.: Local and global minimality results for a nonlocal isoperimetric problem on \({\mathbb{R}}^N\). SIAM J. Math. Anal. 46(4), 2310–2349, 2014
Burago, Y., Zalgaller, V.: Geometric inequalities, vol. 285. Grundlehren der Mathematischen Wissenschaften. Springer, Berlin, 1988
Carbou, G.: Thin layers in micromagnetism. Math. Models Methods Appl. Sci. 11(09), 1529–1546, 2001
Cerruti, B., Zapperi, S.: Dynamic hysteresis from zigzag domain walls: discrete model and Monte Carlo simulations. Phys. Rev. B 75(6), 2007
Choksi, R., Peletier, M.A.: Small volume fraction limit of the diblock copolymer problem: I. Sharp-interface functional. SIAM J. Math. Anal. 42(3), 1334–1370, 2010
Choksi, R., Peletier, Mark A: Small volume-fraction limit of the diblock copolymer problem: II. Diffuse-interface functional. SIAM J. Math. Anal. 43(2), 739–763, 2011
Conti, S., Fonseca, I., Leoni, G.: A \(\Gamma \)-convergence result for the two-gradient theory of phase transitions. Comm. Pure Appl. Math. 55(7), 857–936, 2002
DeSimone, A., Knüpfer, H., Otto, F.: 2-d stability of the Néel wall. Calc. Var. Partial Differ. Equ. 27(2), 233–253, 2006
DeSimone, A., Kohn, R.V., Müller, S., Otto, F.: Recent analytical developments in micromagnetics. In: Bertotti, G., Mayergoyz, I.D. (eds.) The Science of Hysteresis. Physical Modelling, Micromagnetics, and Magnetization Dynamics, vol. 2, pp. 269–381. Academic Press, Oxford 2006
DeSimone, A., Müller, S., Kohn, R.V., Otto, F.: A compactness result in the gradient theory of phase transitions. Proc. R. Soc. Edinb. Sect. A 131(4), 833–844, 2001
Engel-Herbert, R., Schaadt, D.M., et al.: The nature of charged zig-zag domains in MnAs thin films. J. Mag. Mag. Mater. 305(2), 457–463, 2006
Fonseca, I., Popovici, C.: Coupled singular perturbations for phase transitions. Asymptot. Anal. 44(3–4), 299–325, 2005
Garcia-Cervera, C.: Magnetic Domains and Magnetic Domain Walls. PhD thesis, New York University, 1999
Goldman, D., Muratov, C., Serfaty, S.: The \(\Gamma \)-limit of the two-dimensional Ohta–Kawasaki energy. Droplet arrangement via the renormalized energy. Arch. Ration. Mech. Anal. 212, 445–501, 2014
Hamzaoui, S., Labrune, M., Puchalska, I.: Static and dynamic Zig-Zag magnetic domains. Appl. Phys. Lett. 45(11), 1246–1248, 1984
Hubert, A.: Charged walls in thin magnetic films. IEEE Trans. Magn. 15(5), 1251–1260, 1979
Hubert, A., Schäfer, R.: Magnetic Domains: The Analysis of Magnetic Microstructures. Springer, Berlin 1998
Ignat, R., Knüpfer, H.: Vortex energy and \(360^{\circ }\) Néel walls in thin-film micromagnetics. Commun. Pure Appl. Math. 63(12), 1677–1724, 2010
Ignat, R., Moser, R.: A zigzag pattern in micromagnetics. J. Math. Pures Appl. (9) 98(2), 139–159, 2012
Ignat, R., Otto, F.: A compactness result in thin-film micromagnetics and the optimality of the Néel wall. J. Eur. Math. Soc. 10, 909–956, 2008
Julin, Vesa, Pisante, Giovanni: Minimality via second variation for microphase separation of diblock copolymer melts. J. Reine Angew. Math. 729, 81–117, 2017
Kaplan, B., Kaplan, R.: Two-dimensional zigzag domain wall structure in ultrathin films. J. Supercond. Novel Magn. 29(11), 2987–2990, 2016
Knüpfer, H., Muratov, C.: On an isoperimetric problem with a competing non-local term. I. The planar case. Comm. Pure Appl. Math. 66, 1129–1162, 2013
Knüpfer, H., Muratov, C.: On an isoperimetric problem with competing non-local term. II. The general case. Comm. Pure Appl. Math. 67, 1974–1994, 2014
Knüpfer, H., Muratov, C., Nolte, F.: Magnetic domains in thin ferromagnetic films with strong perpendicular anisotropy. Arch. Ration. Mech. Anal. 232(2), 727–761, 2019
Kohn, R.V., Slastikov, V.: Another thin-film limit of Micromagnetics. Arch. Ration. Mech. Anal. 178(2), 227–245, 2005
Landau, L., Lifshitz, E.: On the theory of the dispersion of magnetic permeability in ferromagnetic bodies. Phys. Z. Sowjetunion 8(153), 101–114, 1935
Lieb, E.H., Loss, M.: Analysis, volume 14 of Graduate Studies in Mathematics, 2nd ed. American Mathematical Society, Providence 2001.
Lions, J.L., Magenes, E.: Non-homogeneous Boundary Value Problems and Applications, Vol. I. Springer, New York 1972. Die Grundlehren der mathematischen Wissenschaften, Band 181.
Maggi, F.: Sets of Finite Perimeter and Geometric Variational Problems, volume 135 of Cambridge Studies in Advanced Mathematics. Cambridge University Press, Cambridge 2012. An introduction to geometric measure theory
Melcher, C.: The logarithmic tail of Néel walls. Arch. Ration. Mech. Anal. 168(2), 83–113, 2003
Melcher, C.: Logarithmic lower bounds for Néel walls. Calc. Var. Part. Differ. Equ. 21(2), 209–219, 2004
Moser, R.: On the energy of domain walls in ferromagnetism. Interfaces Free Bound. 11(3), 399–419, 2009
Reshetnyak, Y.: Weak convergence of completely additive vector functions on a set. Siber. Math. J. 9(6), 1039–1045, 1968
Rivière, T., Serfaty, S.: Limiting domain wall energy for a problem related to micromagnetics. Comm. Pure Appl. Math. 54(3), 294–338, 2001
Rivière, T., Serfaty, S.: Compactness, kinetic formulation, and entropies for a problem related to micromagnetics. Comm. Part. Differ. Equ. 28(1–2), 249–269, 2003
Sander, L., Jones, R., Collins, A.: An investigation of saw-tooth domain walls in ni/fe/co films. J. Phys. D 10, 1977
Ukleev, V., Moubah, R., et al.: Imprinted magnetic anisotropy and zigzag domain structure of amorphous TbCo films. J. Supercond. Novel Mag. 28(12), 3571–3577, 2015
Acknowledgements
We are grateful to the referee for carefully reading the file and his useful comments. We also thank J. Fabiszisky for carefully proofreading and drawing some of the pictures. Hans Knüpfer was partially supported by the German Research Foundation (DFG) by the project #392124319 and under Germany’s Excellence Strategy—EXC-2181/1—390900948. Wenhui Shi was partially supported by the German Research Foundation (DFG) by the Project SH 1403/1-1.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by A. Braides
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix A: Real Space Representation of the Stray Field
Appendix A: Real Space Representation of the Stray Field
We first recall the following standard representation of the homogeneous \(H^{\frac{1}{2}}\)-norm. We give the short proof since the constant in front of the identity is essential in our arguments:
Lemma A.1
(Finite difference representation of \(H^{\frac{1}{2}}\)-norm) For \(m \in H^{\frac{1}{2}}(Q_\ell )\) we have
Proof
Using Plancherels identity (8) and Fubini’s theorem, we obtain
The last identity follows with the change of variables \(h\mapsto \frac{h}{|\xi |}\) and since \(\int _{\mathbb {R}^2} \frac{|1-e^{ih_1}|^2}{|h|^3} \ \mathrm{d}h = 4\pi \) (cf. [13, (39)]). \(\square \)
The next lemma yields another representation for the \(H^{\frac{1}{2}}\)-norm when \(j \rightarrow - \infty \) and \(k \rightarrow \infty \).
Lemma A.2
(\(H^{\frac{1}{2}}\)-norm vs. \(H^{-\frac{1}{2}}\)-norm) For \(f \in C^\infty _c(Q_\ell ;\mathbb {R}^2)\) we have
where \(\tilde{Q}_\ell = \mathbb {R}\times [0, \ell )\) and \(\tilde{P}_\ell = \mathbb {R}\times [j \ell , k \ell )\) for any \(j < k \in \mathbb {Z}\).
Proof
Let I (resp. J) be first (second) integral on the second line of (98). We recall the identity \(D (\frac{z}{|z|^3})\) = \(D^t (\frac{z}{|z|^3})\) \(=\) \(\frac{1}{|z|^5}(z^\perp \otimes z^\perp \) − \(2 z \otimes z)\) where \(z^\perp := (-z_2,z_1)\). Integrating by parts in x and y, since \(\nabla _z (\frac{1}{|z|}) = - \frac{z}{|z|^3}\) and since \(\nabla (f \cdot g) = (D^t g) f + (D^tf) g\) then yields
First, integrating in y then in x similarly yields that
Taking the sum of these two expressions, one gets
Since \(\nabla \times f = \nabla \cdot f^\perp \), the same calculation as before, replacing f by \(f^\perp \), yields
The identity (98) follows by taking the sum of the last two equations. The inequality in (98) follows from (99). \(\square \)
We have the following singular integral characterization for the magnetostatic energy:
Lemma A.3
(Integral representations of magnetostatic energy) Let \(\sigma \in L^2(Q_\ell )\) with
Then there is a unique \(q \in H^1(Q_\ell ;\mathbb {R}^2)\) with \(\nabla \cdot q = \sigma \) and \(\nabla \times q = 0\) such that
Proof
By assumption (100) we have \({\widehat{\sigma }}(0) = 0\) and \(\nabla {\widehat{\sigma }} \in L^\infty (\mathbb {R}\times \frac{2\pi }{\ell } \mathbb {Z})\). This implies that \(q \in H^1(Q_\ell ;\mathbb {R}^2)\), where q is defined by its Fourier transform \(\widehat{q} := -i \frac{\xi }{|\xi |^2} {\widehat{\sigma }}\). By construction q satisfies \(\nabla \cdot q = \sigma \) and \(\nabla \times q = 0\). This solution is unique by the uniqueness of the Helmholtz decomposition. By (9), since \(|{\widehat{\sigma }}| = |\xi | |{\widehat{q}}|\) and by (97) we then get
Rights and permissions
About this article
Cite this article
Knüpfer, H., Shi, W. \(\Gamma \)-Limit for Two-Dimensional Charged Magnetic Zigzag Domain Walls. Arch Rational Mech Anal 239, 1875–1923 (2021). https://doi.org/10.1007/s00205-021-01606-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00205-021-01606-x