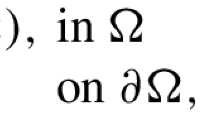Skip to main content
SpringerLink
Log in

Uniqueness of Equilibrium with Sufficiently Small Strains in Finite Elasticity

  • Published:
Archive for Rational Mechanics and Analysis Aims and scope Submit manuscript

Abstract

The uniqueness of equilibrium for a compressible, hyperelastic body subject to dead-load boundary conditions is considered. It is shown, for both the displacement and mixed problems, that there cannot be two solutions of the equilibrium equations of Finite (Nonlinear) Elasticity whose nonlinear strains are uniformly close to each other. This result is analogous to the result of John (Commun Pure Appl Math 25:617–634, 1972), who proved that, for the displacement problem, there is a most one equilibrium solution with uniformly small strains. The proof in this manuscript utilizes Geometric Rigidity, a new straightforward extension of the Fefferman–Stein inequality to bounded domains, and an appropriate adaptation, for Elasticity, of a result from the Calculus of Variations. Specifically, it is herein shown that the uniform positivity of the second variation of the energy at an equilibrium solution implies that this mapping is a local minimizer of the energy among deformations whose gradient is sufficiently close, in \({{\rm BMO}\cap\, L^1}\) , to the gradient of the equilibrium solution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adams, R.A., Fournier, J.J.F.: Sobolev Spaces. \(2^{nd}\) edition. Elsevier/Academic Press, Amsterdam 2003

  2. Alt, H.W.: Linear Functional Analysis. An Application-oriented Introduction. Translated from the German edition by Robert Nürnberg. Springer, London, 2016

  3. Antman, S.S.: The eversion of thick spherical shells. Arch. Ration. Mech. Anal. 70, 113–123 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  4. Ball, J.M.: Convexity conditions and existence theorems in nonlinear elasticity. Arch. Ration. Mech. Anal. 63, 337–403 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  5. Ball, J.M.: Some open problems in elasticity. In: Newton, P., Holmes, P., Weinstein, A. (eds.) Geometry, Mechanics, and Dynamics, pp. 3–59. Springer, New York (2002)

    Chapter  Google Scholar 

  6. Bennett, C., Sharpley, R.: Interpolation of Operators. Academic Press Inc, Boston, MA (1988)

    MATH  Google Scholar 

  7. Benyamini, Y., Lindenstrauss, J.: Geometric Nonlinear Functional Analysis, vol. 1. American Mathematical Society, Providence, RI (2000)

    MATH  Google Scholar 

  8. Bevan, J.J.: Extending the Knops-Stuart-Taheri technique to \(C^1\) weak local minimizers in nonlinear elasticity. Proc. Am. Math. Soc. 139, 1667–1679 (2011)

    Article  MATH  Google Scholar 

  9. Brezis, H., Nirenberg, L.: Degree theory and BMO. I. Compact manifolds without boundaries. Selecta Math. (N.S.) 1, 197–263 1995

  10. Brezis, H., Nirenberg, L.: Degree theory and BMO. II. Compact manifolds with boundaries. With an appendix by the authors and Petru Mironescu. Selecta Math. (N.S.) 2, 309–368 1996

  11. Campos Cordero, J.: Boundary regularity and sufficient conditions for strong local minimizers. J. Funct. Anal. 272, 4513–4587 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  12. Carbonaro, A., Mauceri, G., Meda, S.: \(H^1\) and \({\rm BMO}\) for certain locally doubling metric measure spaces. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 8, 543–582 2009

  13. Carbonaro, A., Mauceri, G., Meda, S.: \(H^1\) and \({\rm BMO}\) for certain locally doubling metric measure spaces of finite measure. Colloq. Math. 118, 13–41 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  14. Carillo, S., Podio-Guidugli, P.: Vergara Caffarelli, G.: Second-order surface potentials in finite elasticity. In: Podio-Guidugli, P., Brocato, M. (eds.) Rational Continua. Classical and New, pp. 19–38. Springer Italia, Milan (2003)

    Chapter  Google Scholar 

  15. Ciarlet, P.G.: Mathematical Elasticity, Vol. I., Elsevier, Amsterdam 1988

  16. Ciarlet, P.G., Mardare, C.: Nonlinear Korn inequalities. J. Math. Pures Appl. 104, 1119–1134 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  17. Conti, S., Schweizer, B.: Rigidity and gamma convergence for solid-solid phase transitions with SO(\(2\)) invariance. Commun. Pure Appl. Math. 59, 830–868 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  18. Conti, S., Dolzmann, G., Müller, S.: Korn's second inequality and geometric rigidity with mixed growth conditions. Calc. Var. Partial Differential Equations 50, 437–454 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  19. Dal Maso, G., Negri, M., Percivale, D.: Linearized elasticity as \(\Gamma \)-limit of finite elasticity. Set-Valued Anal. 10, 165–183 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  20. de Figueiredo, D.G.: The coerciveness problem for forms over vector valued functions. Commun. Pure Appl. Math. 16, 63–94 (1963)

    Article  MathSciNet  MATH  Google Scholar 

  21. Diening, L., R\(\overset{_\circ }{\rm u\it }\)žička, M., Schumacher, K.: A decomposition technique for John domains. Ann. Acad. Sci. Fenn. Math. 35, 87–114 2010

  22. Evans, L.C., Gariepy, R.F.: Measure Theory and Fine Properties of Functions. CRC Press, Boca Raton (1992)

    MATH  Google Scholar 

  23. Fefferman, C., Stein, E.M.: \(H^p\) spaces of several variables. Acta Math. 129, 137–193 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  24. Fefferman, C., Damelin, S.B., Glover, W.: A \({\rm BMO}\) theorem for \(\varepsilon \)-distorted diffeomorphisms on \({\mathbb{R}^{D}}\) and an application to comparing manifolds of speech and sound. Involve 5, 159–172 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  25. Firoozye, N.B.: Positive Second Variation and Local Minimizers in BMO-Sobolev Spaces. Preprint no. 252, 1992, SFB 256, University of Bonn

  26. Fonseca, I., Gangbo, W.: Degree Theory in Analysis and Applications. The Clarendon Press, Oxford University Press, New York (1995)

    MATH  Google Scholar 

  27. Friesecke, G., James, R.D., Müller, S.: A theorem on geometric rigidity and the derivation of nonlinear plate theory from three-dimensional elasticity. Commun. Pure Appl. Math. 55, 1461–1506 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  28. Gao, D., Neff, P., Roventa, I., Thiel, C.: On the convexity of nonlinear elastic energies in the right Cauchy-Green tensor. J. Elast. 127, 303–308 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  29. Grafakos, L.: Classical Fourier analysis. \(3^{nd}\) edition. Springer, New York 2014

  30. Grafakos, L.: Modern Fourier Analysis. \(3^{nd}\) edition. Springer, New York 2014

  31. Grisvard, P.: Elliptic Problems in Nonsmooth Domains. Pitman, Boston, MA (1985)

    MATH  Google Scholar 

  32. Gurtin, M.E.: An Introduction to Continuum Mechanics. Academic Press, New York (1981)

    MATH  Google Scholar 

  33. Gurtin, M.E., Spector, S.J.: On stability and uniqueness in finite elasticity. Arch. Ration. Mech. Anal. 70, 153–165 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  34. Hlaváček, I., Nečas, J.: On inequalities of Korn's type. I. Boundary-value problems for elliptic system of partial differential equations. Arch. Rational Mech. Anal. 36, 305–311 1970

  35. Hofmann, S., Mitrea, M., Taylor, M.: Geometric and transformational properties of Lipschitz domains, Semmes-Kenig-Toro domains, and other classes of finite perimeter domains. J. Geom. Anal. 17, 593–647 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  36. Iwaniec, T.: On \(L^p\)-integrability in PDEs and quasiregular mappings for large exponents. Ann. Acad. Sci. Fenn. Ser. A I Math. 7, 301–322 1982

  37. John, F.: Rotation and strain. Commun. Pure Appl. Math. 14, 391–413 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  38. John, F.: Uniqueness of non-linear elastic equilibrium for prescribed boundary displacements and sufficiently small strains. Commun. Pure Appl. Math. 25, 617–634 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  39. John, F.: Bounds for deformations in terms of average strains. Inequalities, III Proceedings of the Third Symposium, University California, Los Angeles, California, 1969, pp. 129–144. Academic Press, New York, 1972

  40. John, F.: Collected Papers. Vol. 2. (Eds. Moser J.) Birkhäuser Boston, Boston, MA, 1985

  41. John, F., Nirenberg, L.: On functions of bounded mean oscillation. Commun. Pure Appl. Math. 14, 415–426 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  42. Jones, P.W.: Extension theorems for BMO. Indiana Univ. Math. J. 29, 41–66 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  43. Kohn, R.V.: New integral estimates for deformations in terms of their nonlinear strains. Arch. Ration. Mech. Anal. 78, 131–172 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  44. Knops, R.J., Stuart, C.A.: Quasiconvexity and uniqueness of equilibrium solutions in nonlinear elasticity. Arch. Ration. Mech. Anal. 86, 233–249 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  45. Kristensen, J., Taheri, A.: Partial regularity of strong local minimizers in the multi-dimensional calculus of variations. Arch. Ration. Mech. Anal. 170, 63–89 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  46. Leoni, G.: A First Course in Sobolev Spaces. American Mathematical Society, Providence, RI (2009)

    MATH  Google Scholar 

  47. Martio, O., Sarvas, J.: Injectivity theorems in plane and space. Ann. Acad. Sci. Fenn. Ser. A I Math. 4, 383–401 1979

  48. Morrey Jr., C.B.: Multiple Integrals in the Calculus of Variations. Springer, New York (1966)

    MATH  Google Scholar 

  49. Neff, P., Pompe, W.: Counterexamples in the theory of coerciveness for linear elliptic systems related to generalizations of Korn's second inequality. Z. Angew. Math. Mech. 94, 784–790 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  50. Neukamm, S., Schäffner, M.: Quantitative homogenization in nonlinear elasticity for small loads. Arch. Ration. Mech. Anal. 230, 343–396 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  51. Neukamm, S., Schäffner, M.: Lipschitz estimates and existence of correctors for nonlinearly elastic, periodic composites subject to small strains. arXiv:1807.09109

  52. Ogden, R.W.: Non-linear Elastic Deformations. Dover, Chicago (1984)

    MATH  Google Scholar 

  53. Podio-Guidugli, P., Vergara-Caffarelli, G.: Surface interaction potentials in elasticity. Arch. Ration. Mech. Anal. 109, 343–383 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  54. Pompe, W.: Korn's first inequality with variable coefficients and its generalization. Comment. Math. Univ. Carolin. 44, 57–70 (2003)

    MathSciNet  MATH  Google Scholar 

  55. Post, K.D.E., Sivaloganathan, J.: On homotopy conditions and the existence of multiple equilibria in finite elasticity. Proc. R. Soc. Edinburgh Sect. A 127, 595–614 1997 [Erratum: 127, 1111 (1997)]

  56. Rešetnjak, J.G.: Liouville's conformal mapping theorem under minimal regularity hypotheses. (Russian) Sibirsk. Mat. Ž. 8, 835–840 1967 [English Translation: Siberian Math. J. 8, 631–634 (1967)]

  57. Sewell, M.J.: On configuration-dependent loading. Arch. Ration. Mech. Anal. 23, 327–351 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  58. Šilhavý, M.: The Mechanics and Thermodynamics of Continuous Media. Springer, Berlin (1997)

    MATH  Google Scholar 

  59. Sivaloganathan, J., Spector, S.J.: On the uniqueness of energy minimizers in finite elasticity. J. Elast. 133, 73–103 (2018)

    Article  MathSciNet  Google Scholar 

  60. Spector, S.J.: On uniqueness in finite elasticity with general loading. J. Elast. 10, 145–161 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  61. Stein, E.M.: Singular Integrals and Differentiability Properties of Functions. Princeton University Press, Princeton, N.J. (1970)

    MATH  Google Scholar 

  62. Stein, E.M.: Harmonic Analysis: Real-variable Methods, Orthogonality, and Oscillatory Integrals. Princeton University Press, Princeton, NJ (1993)

    MATH  Google Scholar 

  63. Taheri, A.: Quasiconvexity and uniqueness of stationary points in the multi-dimensional calculus of variations. Proc. Am. Math. Soc. 131, 3101–3107 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  64. Truesdell, C., Noll, W.: The Non-linear Field Theories of Mechanics. Handbuch der Physik, Band III/3. Springer, Berlin 1965

  65. Valent, T.: Boundary Value Problems of Finite Elasticity. Springer, New York (1988)

    Book  MATH  Google Scholar 

  66. Verde, A., Zecca, G.: On the higher integrability for certain nonlinear problems. Differ. Integral Equ. 21, 247–263 (2008)

    MathSciNet  MATH  Google Scholar 

  67. Whitney, H.: Functions differentiable on the boundaries of regions. Ann. Math. 2(35), 482–485 (1934)

    Article  MathSciNet  MATH  Google Scholar 

  68. Zeidler, E.: Nonlinear Functional Analysis and its Applications. I. Fixed-Point Theorems. Translated from the German by P. R. Wadsack. Springer, New York 1986

  69. Zhang, K.: Energy minimizers in nonlinear elastostatics and the implicit function theorem. Arch. Ration. Mech. Anal. 114, 95–117 (1991)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors thank Mario Milman for interesting discussions regarding BMO and interpolation theory. The authors thank the referee for helpful comments and for providing a short proof of Lemma 4.6. The authors also thank one of the referees of [59] for their suggestion that the results in Kristensen and Taheri [45] might lead to an extension of John’s [38] uniqueness theorem to the mixed problem.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, National Chiao Tung University, Hsinchu, Taiwan

    Daniel E. Spector

  2. National Center for Theoretical Sciences, National Taiwan University, No. 1 Sec. 4 Roosevelt Rd., Taipei, 106, Taiwan

    Daniel E. Spector

  3. Department of Mathematics, Southern Illinois University, Carbondale, IL, 62901, USA

    Scott J. Spector

Authors
  1. Daniel E. Spector
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Scott J. Spector
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Daniel E. Spector.

Additional information

Communicated by S. Müller

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The first author (DS) is supported by the Taiwan Ministry of Science and Technology under research Grants 105-2115-M-009-004-MY2, 107-2918-I-009-003 and 107-2115-M-009-002-MY2

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Spector, D.E., Spector, S.J. Uniqueness of Equilibrium with Sufficiently Small Strains in Finite Elasticity. Arch Rational Mech Anal 233, 409–449 (2019). https://doi.org/10.1007/s00205-019-01360-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00205-019-01360-1

Search

Navigation