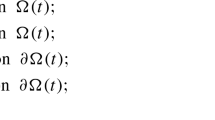Abstract
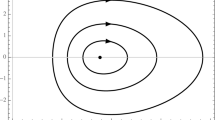
The 3D compressible and incompressible Euler equations with a physical vacuum free boundary condition and affine initial conditions reduce to a globally solvable Hamiltonian system of ordinary differential equations for the deformation gradient in \({{\rm GL}^+(3, \mathbb{R})}\). The evolution of the fluid domain is described by a family of ellipsoids whose diameter grows at a rate proportional to time. Upon rescaling to a fixed diameter, the asymptotic limit of the fluid ellipsoid is determined by a positive semi-definite quadratic form of rank r = 1, 2, or 3, corresponding to the asymptotic degeneration of the ellipsoid along 3−r of its principal axes. In the compressible case, the asymptotic limit has rank r = 3, and asymptotic completeness holds, when the adiabatic index \({\gamma}\) satisfies \({4/3 < \gamma < 2}\). The number of possible degeneracies, 3−r, increases with the value of the adiabatic index \({\gamma}\). In the incompressible case, affine motion reduces to geodesic flow in \({{\rm SL}(3, \mathbb{R})}\) with the Euclidean metric. For incompressible affine swirling flow, there is a structural instability. Generically, when the vorticity is nonzero, the domains degenerate along only one axis, but the physical vacuum boundary condition fails over a finite time interval. The rescaled fluid domains of irrotational motion can collapse along two axes.
Similar content being viewed by others
References
Arnold, V.: Sur la géométrie différentielle des groupes de Lie de dimension infinie et ses applications à l’hydrodynamique des fluides parfaits. Ann. Inst. Fourier 16(fasc. 1), 319–361 (1966)
Chemin J.Y.: Dynamique des gaz à masse totale finie. Asymptot. Anal. 3(3), 215–220 (1990)
Christodoulou, D.: The formation of black holes in general relativity. EMS Monographs in Mathematics. European Mathematical Society (EMS), Zürich (2009). doi:10.4171/068
Christodoulou, D., Lindblad, H.: On the motion of the free surface of a liquid. Comm. Pure Appl. Math. 53(12), 1536–1602 (2000). doi:10.1002/1097-0312(200012)53:12<1536::AID-CPA2>3.3.CO;2-H
Coutand D., Lindblad H., Shkoller S.: A priori estimates for the free-boundary 3D compressible Euler equations in physical vacuum. Comm. Math. Phys. 296(2), 559–587 (2010). doi:10.1007/s00220-010-1028-5
Coutand D., Shkoller S.: Well-posedness of the free-surface incompressible Euler equations with or without surface tension. J. Am. Math. Soc. 20(3), 829–930 (2007). doi:10.1090/S0894-0347-07-00556-5
Coutand D., Shkoller S.: A simple proof of well-posedness for the free-surface incompressible Euler equations. Discret. Contin. Dyn. Syst. Ser. S 3(3), 429–449 (2010). doi:10.3934/dcdss.2010.3.429
Coutand D., Shkoller S.: Well-posedness in smooth function spaces for the moving-boundary three-dimensional compressible Euler equations in physical vacuum. Arch. Ration. Mech. Anal. 206(2), 515–616 (2012). doi:10.1007/s00205-012-0536-1
Grassin M.: Global smooth solutions to Euler equations for a perfect gas. Indiana Univ. Math. J. 47(4), 1397–1432 (1998). doi:10.1512/iumj.1998.47.1608
Hadžić, M., Jang, J.: Expanding Large Global Solutions of the Equations of Compressible Fluid Mechanics. arXiv:1610.01666 (2016). http://arxiv.org/abs/1610.01666
Jang, J., Masmoudi, N.: Well and ill-posedness for compressible Euler equations with vacuum. J. Math. Phys. 53(11), 115,625 (2012). doi:10.1063/1.4767369
Jang J., Masmoudi N.: Well-posedness of compressible Euler equations in a physical vacuum. Comm. Pure Appl. Math. 68(1), 61–111 (2015). doi:10.1002/cpa.21517
Lax P.D.: Development of singularities of solutions of nonlinear hyperbolic partial differential equations. J. Math. Phys. 5, 611–613 (1964)
Lindblad H.: Well-posedness for the linearized motion of a compressible liquid with free surface boundary. Comm. Math. Phys. 236(2), 281–310 (2003). doi:10.1007/s00220-003-0812-x
Lindblad H.: Well-posedness for the linearized motion of an incompressible liquid with free surface boundary. Comm. Pure Appl. Math. 56(2), 153–197 (2003). doi:10.1002/cpa.10055
Lindblad H.: Well posedness for the motion of a compressible liquid with free surface boundary. Comm. Math. Phys. 260(2), 319–392 (2005). doi:10.1007/s00220-005-1406-6
Lindblad H.: Well-posedness for the motion of an incompressible liquid with free surface boundary. Ann. Math. 162(1), 109–194 (2005). doi:10.4007/annals.2005.162.109
Liu T.P.: Compressible flow with damping and vacuum. Jpn. J. Ind. Appl. Math. 13(1), 25–32 (1996). doi:10.1007/BF03167296
Majda, A.: Vorticity and the mathematical theory of incompressible fluid flow. Comm. Pure Appl. Math. 39(S, suppl.), S187–S220 (1986). doi:10.1002/cpa.3160390711. Frontiers of the Mathematical Sciences (New York, 1985)
Makino T., Ukai S., Kawashima S.: Sur la solution à support compact de l’équations d’Euler compressible. Jpn. J. Appl. Math. 3(2), 249–257 (1986). doi:10.1007/BF03167100
Makino, T., Ukai, S., Kawashima, S.: On compactly supported solutions of the compressible Euler equation. In: Recent Topics in Nonlinear PDE, III (Tokyo, 1986), North-Holland Mathematics Studies, vol. 148, pp. 173–183. North-Holland, Amsterdam (1987). doi:10.1016/S0304-0208(08)72332-6
Rouchon P.: The Jacobi equation, Riemannian curvature and the motion of a perfect incompressible fluid. Eur. J. Mech. B Fluids 11(3), 317–336 (1992)
Serre, D.: Solutions classiques globales des équations d’Euler pour un fluide parfait compressible. Ann. Inst. Fourier 47(1), 139–153 (1997). http://www.numdam.org/item?id=AIF_1997__47_1_139_0
Serre D.: Expansion of a compressible gas in vacuum. Bull. Inst. Math. Acad. Sin. (N.S.) 10(4), 695–716 (2015)
Sideris, T.C.: Formation of singularities in three-dimensional compressible fluids. Comm. Math. Phys. 101(4), 475–485 (1985). http://projecteuclid.org/getRecord?id=euclid.cmp/1104114244
Sideris T.C.: Spreading of the free boundary of an ideal fluid in a vacuum. J. Differ. Equ. 257(1), 1–14 (2014). doi:10.1016/j.jde.2014.03.006
Wu S.: Well-posedness in Sobolev spaces of the full water wave problem in 2-D. Invent. Math. 130(1), 39–72 (1997). doi:10.1007/s002220050177
Wu S.: Well-posedness in Sobolev spaces of the full water wave problem in 3-D. J. Am. Math. Soc. 12(2), 445–495 (1999). doi:10.1090/S0894-0347-99-00290-8
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by P. Constantin
Rights and permissions
About this article
Cite this article
Sideris, T.C. Global Existence and Asymptotic Behavior of Affine Motion of 3D Ideal Fluids Surrounded by Vacuum. Arch Rational Mech Anal 225, 141–176 (2017). https://doi.org/10.1007/s00205-017-1106-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00205-017-1106-3