The Elastic Trefoil is the Doubly Covered Circle

Abstract

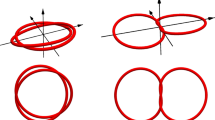
To describe the behavior of knotted loops of springy wire with an elementary mathematical model we minimize the integral of squared curvature, ${E = \int \varkappa^2}$, together with a small multiple of ropelength ${\mathcal{R}}$ = length/thickness in order to penalize selfintersection. Our main objective is to characterize all limit configurations of energy minimizers of the total energy ${E_{\vartheta} \equiv E + \vartheta \mathcal{R}}$ as ${\vartheta}$ tends to zero. For short, these limit configurations will be referred to as elastic knots. The elastic unknot turns out to be the once covered circle with squared curvature energy ${(2\pi)^2}$. For all (non-trivial) knot classes for which the natural lower bound ${(4\pi)^2}$ on E is sharp, the respective elastic knot is the doubly covered circle. We also derive a new characterization of two-bridge torus knots in terms of E, proving that the only knot classes for which the lower bound ${(4\pi)^2}$ on E is sharp are the ${(2,b)}$-torus knots for odd b with ${|b| \ge 3}$ (containing the trefoil knot class). In particular, the elastic trefoil knot is the doubly covered circle.