Political selection and the optimal concentration of political power

Abstract

We study how policy choice and political selection are affected by the concentration of political power. In a setting with inefficient policy gambles, variations in power concentration give rise to a trade-off. On the one hand, power-concentrating institutions allocate more power to the voters’ preferred candidate. On the other hand, they induce the adoption of more overly risky policies and decrease the voters’ capability to select well-suited politicians. We show that full concentration of power is optimal if and only if the conflict of interest between voters and politicians is small. Otherwise, an intermediate level of power concentration is optimal.

Appendices

Appendix

Proofs of Propositions and Lemmas

1.1 Proof of Proposition 1

Proof

Under perfect information, the voter can directly compare the expected payoffs from both candidates’ proposals. Hence, his optimal strategy is to choose $s(x_1,x_{2})=1$ if $x_1 \left( a_1 - c\right) > x_{2} \left( a_2 - c\right)$ and $s(x_1,x_{2})=0$ if $x_1 \left( a_1 - c\right) < x_{2} \left( a_2 - c\right)$. Denote by

$$\begin{aligned} E\left[ \pi _1(w,\rho )\right] = E_{a_{2}, \theta _2}\left[ \rho \ s\left( x_1,X_{2}\left( a_2, \theta _2\right) \right) +(1-\rho )\left( 1-s\left( x_1,X_{2}\left( a_2, \theta _2\right) \right) \right) \right] \; \end{aligned}$$

the expected power of candidate 1, given s and candidate 2’s strategy. $E\left[ \pi _1(\cdot )\right]$ is monotonically increasing in $x_1$ for $a_1>c$ and monotonically decreasing in $x_1$ for $a_1<c$. Candidate 1 chooses $x_1$ to maximize $E\left[ \pi _1(w,\rho )\right] \ \left[ x_1(a_1-c)+\theta _1\right]$. For $a_1>c$, a full reform $x_1=1$ maximizes both her expected power $E[\pi _1(\cdot )]$ and the expected policy payoff $x_1 \left( a_1-c\right)$. For $a_1<c$, in contrast, the status $x_1=0$ maximizes her winning probability as well as the expected policy payoff. Note that $x_1=0$ is the unique optimal action because it leads to a strictly positive winning probability against any opponent with $a_2<c$.

As shown above, changes in $\rho$ do influence neither candidate behavior nor the quality of political selection. However, the higher $\rho$, the higher is the expected power of the winning candidate, i.e., the one whose expected policy payoff is higher. Hence, voter welfare strictly increases with the level of power concentration. $\square$

1.2 Proof of Proposition 2 and Corollary 1

We prove Proposition 2 and Corollary 1 through a series of Lemmas. Lemma 2 shows that the candidates’ policy preferences satisfy the Gans and Smart (1996) single-crossing property. Lemma 3 characterizes the strategies in any PBE. Lemma 4 identifies equilibria that are robust to D1 and unprejudiced beliefs. Lemma 5 proves the existence of a unique D1 equilibrium, which is symmetric.

Lemma 2

Fix $\theta ^H> \theta ^L>0$, the voter’s strategy s and the strategy $X_{-i}$ of candidate $-i$. Denote by $\hat{\pi }_i(x)$ the expected power of candidate i for proposal x, given s and $X_{-i}$. Consider two actions $x_a$ and $x_b>x_a$ such that $\hat{\pi }_i(x_a)>0$, $\hat{\pi }_i(x_b)>0$.

• (i) If i weakly prefers $x_b$ to $x_a$ for some type $(a',\theta ^j)$ such that $\theta ^j + x_b(a'-c)>0$, then i strictly prefers $x_b$ to $x_a$ for any type $(a'',\theta ^j)$ with $a''>a'$.

• (ii) If i weakly prefers $x_a$ to $x_b$ for some type $(a',\theta ^j)$ such that $\theta ^j + x_a(a'-c)>0$, then i strictly prefers $x_a$ to $x_b$ for any type $(a'',\theta ^j)$ with $a''<a'$ and $\theta ^j + x_a(a''-c)>0$.

Proof

For any action x, we denote candidate i’s expected utility by $\hat{u}_i(x,a,\theta ^j):=\hat{\pi }_i(x)$$\left[ \theta ^j+x(a-c)\right]$. For part (i), she weakly prefers $x_b$ to $x_a$ for type $(a',\theta ^j)$ if $\hat{u}_i(x_b,a',\theta ^j) \ge \hat{u}_i(x_a,a',\theta ^j)$, which is equivalent to

$$\begin{aligned} \frac{\hat{\pi }_i(x_b)}{\hat{\pi }_i(x_a)} \ge \frac{\theta ^j + x_a(a'-c)}{\theta ^j + x_b(a'-c)}\; . \end{aligned}$$

Whenever $x_b> x_a$, the right-hand side of this inequality is strictly decreasing in $a'$. Hence, for any $a''>a'$, the strict inequality $\hat{u}_i(x_b,a'',\theta ^j) > \hat{u}_i(x_a,a'',\theta ^j)$ is satisfied, i.e., i strictly prefers $x_b$ to $x_a$.

For part (ii), let $\theta ^j + x_a(a'-c)>0$. Then, i weakly prefers $x_a$ to $x_b$ for type $(a',\theta ^j)$ if

$$\begin{aligned} \frac{\hat{\pi }_i(x_a)}{\hat{\pi }_i(x_b)} \ge \frac{\theta ^j + x_b(a'-c)}{\theta ^j + x_a(a'-c)}\; . \end{aligned}$$

Whenever $x_b>x_a$, the right-hand side of this inequality is strictly increasing in $a'$. Hence, for any $a''<a'$ such that $\theta ^j + x_a(a''-c)>0$, the strict inequality $\hat{u}_i(x_a,a'',\theta ^j) > \hat{u}_i(x_b,a'',\theta ^j)$ is satisfied, i.e., i strictly prefers $x_a$ to $x_b$. $\square$

Lemma 3

Assume that Assumption 1 holds and that $\theta ^H>\theta ^L>0$. In every PBE, the equilibrium strategy $X_i^*$ of candidate i satisfies the following properties:

• (i) $X_i^*(a,\theta )= 0$ for a subset of types with strictly positive probability mass.

• (ii) $X_i^*(a, \theta )$ is monotonically increasing in a.

• (iii) There is a policy ${\tilde{x}}_i \in [0,1]$ such that $X_i^*(a,\theta )= {\tilde{x}}_i$ for all $a\ge c$.

• (iv) There are two cutoffs $\alpha ^L_i \in (0,c)$ and $\alpha ^H_i \in (0,c)$ and a policy ${\tilde{x}}_i\in [0,1]$ such that

  $$\begin{aligned} X_i^*(a, \theta ^j) = {\left\{ \begin{array}{ll} 0 &{} \text {if} ~~~a < \alpha ^j_i \ \text {and} \ \theta = \theta ^j, \\ {\tilde{x}}_i &{} \text {if} ~~~a \ge \alpha ^j_i \ \text {and} \ \theta = \theta ^j\; . \end{array}\right. } \end{aligned}$$

  (11)

Proof

For part (i), denote by $\hat{a}_i(x)$ the expected ability and by $\hat{v}_i(x) =:x \left[ \hat{a}_i(x) -c\right]$ the expected policy payoff of agents proposing x. Assume there is a PBE with strategies $(X_1^*,X_2^*)$ such that candidate 1 does not play $x_1=0$ for any type. Recall that the expected ability E[a] is below c by Assumption 1. Then, there are a policy $x'>0$ and a type $(a_1,\theta _1)$ with $a_1<c$ such that $X_1^*(a_1,\theta _1) = x'>0$, $\hat{a}_1(x')<c$ and $\hat{v}_1(x')<0$. For this type, the expected utility is given by $\hat{u}(x',a_1,\theta _1) = \hat{\pi }_1(x') \left[ \theta _1 + x' (a_1-c)\right]$, where $\hat{\pi }_1(x')$ follows from the voter’s optimal strategy, given correct beliefs about $\hat{v}_1(x')<0$. Assume that type $(a_1,\theta _1)$ deviates from $x'$ to a status quo proposal $x_1=0$, thereby increasing the voter’s expected policy payoff to $\hat{v}_1(0)=0>\hat{v}_1(x')$. Given unprejudiced beliefs, the voter prefers candidate 1 weakly more often so that $\hat{\pi }_1(0) \ge \hat{\pi }_1(x')$. Note also that $\hat{\pi }_1(0)$ is strictly positive, because 1 wins with positive probability whenever $\hat{v}_2(x_2)<0$ or $x_2=0$ (by the tie-breaking rule $s(0,0)=1/2$). Hence, the deviation to $x_1=0$ is strictly profitable. But then, the initial strategy $X_1^*$ cannot be part of a PBE. We conclude that candidate 1 plays $x_1=0$ with strictly positive probability in every PBE.

For part (ii), assume that $X_i^*(a,\theta ^j)=x'$ for candidate i given some type $(a,\theta ^j)$. Her utility $\hat{u}_i(x',a,\theta ^j) = \hat{\pi }_i(x') \left[ \theta ^j+ x'(a-c)\right]$ must be weakly larger than $\hat{u}(0,a,\theta ^j)= \hat{\pi }_i (0) \theta ^j>0$, where $\theta ^j>0$ by assumption and $\hat{\pi }_i(0)>0$ by part (i). Hence, the conditions $\pi _i(x)>0$ and $\theta _j + x(a-c)>0$ are satisfied for $(x',a,\theta ^j)$ and the single-crossing condition established in Lemma 2 (i) holds for each pair of equilibrium actions. Thus, $X_i^*(a,\theta )$ is monotonically increasing in a in every PBE.

For part (iii), the expected utility of candidate i with type $(c,\theta )$ is given by $\hat{u}_i(x,c,\theta ) = \hat{\pi }_i(x) \theta$. Hence, $X_i^*(c,\theta )$ is equal to a maximizer ${\tilde{x}}_i$ of $\hat{\pi }_i(x)$ for each $\theta \in \left\{ \theta ^L,\theta ^H\right\}$. Assume for now that ${\tilde{x}}_i$ is the unique maximizer of $\hat{\pi }_i(x)$ and that there is a policy $x'\ne {\tilde{x}}_i$ such that $X_i^*(1,\theta ^j)= x'$ for at least one $j \in \left\{ L,H\right\}$. By part (ii), we must have $x'>{\tilde{x}}_i$. Moreover, there must exist a cutoff ${\tilde{\alpha }}^j_i \in (c,1]$ such that $X_i^*(a,\theta ^j)=x'$ for all $a>{\tilde{\alpha }}^j_i$. If $X_i^*(1,\theta ^{-j})= x'$ as well, there must be another cutoff ${\tilde{\alpha }}^{-j}_i \in (c,1)$ such that $X_i^*(a,\theta ^{-j})=x'$ for all $a>{\tilde{\alpha }}^{-j}_i$. In any case, we have $\hat{v}_i(x')>\hat{v}_i({\tilde{x}}_i)$, which implies that $\hat{\pi }_i(x')>\hat{\pi }_i({\tilde{x}}_i)$. Hence, we have a contradiction: ${\tilde{x}}_i$ does not maximize $\hat{\pi }_i(x)$.

Next, assume that both $\underline{x}$ and ${\bar{x}}> \underline{x}$ are maximizers of $\hat{\pi }_i(x)$. Then, candidate i is indifferent between $\underline{x}$ and ${\bar{x}}$ for type $(c,\theta )$. By Lemma 2, i strictly prefers ${\bar{x}}$ to $\underline{x}$ for each $a_i>c$, while she strictly prefer $\underline{x}$ for all $a_i < c$. Consequently, there must be a policy $x' \ge {\bar{x}}$ that is only proposed by candidates with expected ability above c and, correspondingly, gives a strictly positive expected payoff $\hat{v}_i(x')$. In contrast, policy $\underline{x}$ is only proposed by candidates with expected ability below c and, correspondingly, gives a weakly negative payoff. But then, $x'$ must give a strictly larger power than $\underline{x}$, i.e., the latter cannot be a maximizer of $\hat{\pi }_i(x)$. We conclude that there is a unique maximizer ${\tilde{x}}_i$, which is proposed by i given ability c as well as ability 1. By the monotonicity established in part (ii), $X_i^*(a_i,\theta ^j)= {\tilde{x}}_i$ for each $a_i\ge c$ and each $j \in \left\{ L,H\right\}$.

For part (iv), recall that candidate i proposes the actions 0 and ${\tilde{x}}_i = \arg \max \hat{\pi }(x)$ along the equilibrium path and that $\hat{\pi }_i(0)>0$. Assume that $X_i^*(a',\theta ^j) = x' \notin \left\{ 0,{\tilde{x}}_i\right\}$ for some ability $a'$ and some $j\in \left\{ L,H\right\}$. By part (iii), $x'$ can only be proposed by a subset of types with $a<c$. Hence, the expected policy payoff from $x'$ must be negative, $\hat{v}_i(x')<0$, which also implies that $\hat{\pi }_i(x') < \hat{\pi }_i(0)$ in every PBE. But then, as explained in part (i), a deviation from $x'$ to 0 is profitable for type $(a',\theta ^j)$. Hence, only the policies 0 and ${\tilde{x}}_i$ are proposed along the equilibrium path. By part (ii), there must be two cutoffs $\alpha ^L$ and $\alpha ^H$ such that $X_i^*(a,\theta ^j)$ is equal to 0 for all $(a,\theta ^j)$ with $a < \alpha ^j$, and equal to ${\tilde{x}}_i$ for all $(a,\theta ^j)$ with $a \ge \alpha ^j$. By parts (i) and (iii), both cutoffs are located in (0, c). $\square$

Lemma 4

Assume that Assumption 1 holds and that $\theta ^H>\theta ^L>0$. In every D1 equilibrium, $X_i^*(1,\theta ^j)= 1$ for each $j \in \left\{ L,H\right\}$ and each $i \in \left\{ 1,2\right\}$.

Proof

The following proof shows that, first, PBE equilibria with $X_i^*(1,\theta ^j)=1$ are robust to D1 and, second, PBE equilibria with $X_i^*(1,\theta ^j)= {\tilde{x}}_i < 1$ are not robust to D1. The D1 criterion restricts the set of viable beliefs off the equilibrium path (Cho and Kreps 1987). Essentially, D1 says that a deviation of agent i to some action $\varepsilon$ cannot be assigned to type $(a,\theta )$ if there is another type $(a',\theta ')$ with the following properties: For every potential best response s by the voter such that a deviation to $\varepsilon$ is weakly profitable for $(a,\theta )$, the deviation is strictly profitable for $(a', \theta ')$. In our setting, the strategy of the voter affects the candidates only through its impact on their expected political power $\hat{\pi }_i(\varepsilon )$. To apply D1, it therefore suffices to consider the set of viable levels of $\hat{\pi }_i(\varepsilon ) \in [1-\rho ,\rho ]$.

First, we show that each PBE with $X_i^*(1,\theta ^j)=1$ is robust to D1. Consider a deviation from $X_i^*(a,\theta ^j) \in \left\{ 0,1\right\}$ to $\varepsilon \in (0,1)$. The deviation gives utility $\hat{u}_i(\varepsilon ,a,\theta ^j) = \hat{\pi }_i(\varepsilon ) \left[ \theta ^j + \varepsilon (a-c)\right]$, while $\hat{u}_i(X_i^*(a,\theta ^j),a,\theta ^j)\ge \hat{u}_i(0,a,\theta ^j) = \hat{\pi }_i(0) \ \theta ^j >0$. Hence, the deviation can only be profitable for $(a,\theta ^j)$ if $\hat{\pi }_i(\varepsilon )>0$ and $\theta ^j + \varepsilon (a-c)>0$. Thus, we can exploit the single-crossing property established in Lemma 2, using $0< \varepsilon <1$. Consider a type $(a,\theta ^j)$ such that a is below the cutoff $\alpha _i^j$ and $X_i^*(a,\theta )=0$ (see Lemma 3). By Lemma 2 (i), for any $\hat{\pi }_i(\varepsilon )$ such that $(a,\theta ^j)$ weakly prefers $\varepsilon$ to 0, any type $(a', \theta ^j)$ with $a'\in (a,\alpha ^j_i)$ strictly prefers $\varepsilon$ to 0. Hence, we cannot assign the deviation to a type with $a< \alpha _i^j$. Consider a type $(a,\theta ^j)$ such that $a>\alpha ^j_i$ and $X_i^*(a,\theta )=1$. For any such type, we have $\theta ^j + 1 (a - c) >0$, which also ensures that $\theta ^j + \varepsilon (a - c) >0$. We can hence apply Lemma 2 (ii): For any $\hat{\pi }_i(\varepsilon )$ such that $(a,\theta ^j)$ weakly prefers $\varepsilon$ to 1, any type $(a', \theta ^j)$ with $a' \in (\alpha ^j_i,a)$ strictly prefers $\varepsilon$ to 1. Hence, we cannot assign the deviation to a type with $a> \alpha ^j$. As a result, D1 requires that any deviation from the equilibrium actions 0 or 1 to some $\varepsilon \in (0,1)$ is associated with one of the cutoff types $(\alpha ^L_i,\theta ^L)$ or $(\alpha ^H_i,\theta ^H)$. For these cutoff types, a deviation is profitable whenever $\hat{\pi }_i(\varepsilon ) \ge \hat{\pi }_i(1) \in [1-\rho ,\rho ]$. Hence, the set of voter responses such that the deviation is profitable is non-empty. In both cases, because $\alpha ^L_i<c$ and $\alpha ^H_i<c$, the D1 beliefs imply an expected payoff $\hat{v}_i(\varepsilon ) <0$ and, hence, $\hat{\pi }_i(\varepsilon )< \hat{\pi }_i(0) < \hat{\pi }_i(1)$. Given these beliefs, the deviation is not profitable for any type because $\hat{u}(\varepsilon ,\alpha ^j_i,\theta ^j) < \hat{u}(0,\alpha ^j_i, \theta ^j) = \hat{u}(1,\alpha ^j_i, \theta ^j)$ for $j \in \left\{ L,H\right\}$.

Second, we show that PBE with $X_i^*(1,\theta ^j)= {\tilde{x}}_i < 1$ are not robust to D1. Consider a PBE with ${\tilde{x}}_i \in [0,1)$ and a deviation to $x_i=1$, giving utility $\hat{u}_i(1,a,\theta ^j) = \hat{\pi }_i(1) \left[ \theta ^j + a-c\right]$. Again, the deviation can only be profitable for $(a,\theta ^j)$ if $\hat{\pi }_i(1)>0$ and $\theta ^j + a-c>0$. Thus, we can exploit the single-crossing property, using $1>{\tilde{x}}_i \ge 0$. By Lemma 2 (i), for any $\hat{\pi }_i(1)$ such that $(a,\theta ^j)$ weakly prefers 1 to 0, any type $(a', \theta ^j)$ with $a'>a$ strictly prefers 1 to 0. Moreover, for any $\hat{\pi }_i(1)$ such that $(a,\theta ^j)$ weakly prefers 1 to ${\tilde{x}}_i$, any type $(a', \theta ^j)$ with $a'>a$ strictly prefers 1 to ${\tilde{x}}_i$. Hence, D1 requires that the voter assigns the deviation to 1 to a type $(1,\theta ^j)$ with $j \in \left\{ L,H\right\}$. For such a type, the expected policy payoff $\hat{v}_i(1) = 1-c$ is strictly larger than $\hat{v}_i({\tilde{x}}_i) = {\tilde{x}}_i [\hat{a}_i({\tilde{x}}_i)-c]$ and, hence, gives higher expected power $\hat{\pi }_i(1)> \hat{\pi }_i({\tilde{x}}_i)$. Hence, the deviation from ${\tilde{x}}$ to 1 increases i’s office rents as well as her legacy payoff and is thus strictly profitable for type $(1,\theta ^j)$ and $j\in \left\{ L,H\right\}$. As a result, the PBE with ${\tilde{x}}_i \in [0,1)$ is not robust to D1. $\square$

Lemma 5

Assume that Assumptions 1 and 2 hold and that $\theta ^L \rightarrow 0$.

• (i) In every D1 equilibrium, $\alpha _1^L=\alpha _2^L = c$.

• (ii) There is a unique symmetric D1 equilibrium with either $\alpha _1^H=\alpha _2^H \in (\underline{a}, c)$ and $s_{10}=1$, or $\alpha _1^H=\alpha _2^H=\underline{a}$ and $s_{10} \in (1/2,1]$.

• (iii) If $s(0,0)=1/2$, there exists no asymmetric equilibrium with $\alpha _1^H \ne \alpha _2^H$.

Proof

Assume that there is a D1 equilibrium. By Lemmas 3 and 4, there is a unique cutoff $\alpha ^j_i \in (0,c)$ such that a candidate with type $(a,\theta ^j)$ prefers $x_i=1$ to $x_i=0$ if and only if a is above a threshold $\alpha _i^j$. For each $j \in \left\{ L,H\right\}$, the threshold $\alpha ^j_i$ is defined by the indifference condition

$$\begin{aligned} \hat{u}_i(1,\alpha ^j,\theta ^j) - \hat{u}_i(0,\alpha ^j,\theta ^j) = \left[ \hat{\pi }_i(1)- \hat{\pi }_i(0)\right] \theta ^j + \hat{\pi }_i(1) (\alpha ^j-c) =0\; . \end{aligned}$$

(12)

For part (i), consider the limit case $\theta ^L \rightarrow 0$ and recall that $\hat{\pi }_i(1) \ge \hat{\pi }_i(0)>0$. For $j = L$, indifference condition (12) is satisfied if and only if $\alpha ^L=c$. This implies that candidate i proposes the status quo with probability $K(\alpha _i^H) = \mu \varPhi (\alpha _i^H) + (1-\mu ) \varPhi (c)$, and a reform with probability $1-K(\alpha _i^H)$.

Part (ii) proves the existence and uniqueness of a symmetric D1 equilibrium and, at the same time, Corollary 1. In any symmetric equilibrium, we have $\alpha _1^L=\alpha _2^L=c$, $\alpha _1^H=\alpha _2^H=\alpha$, $s(1,1)=s(0,0)=1/2$ and $s(1,0)=1-s(0,1)=s_{10}$. Ex ante, candidate i proposes the reform with probability $K(\alpha ) = \mu \varPhi (\alpha ) + (1-\mu ) \varPhi (c)$. The expected power shares for both possible actions follow as

$$\begin{aligned} \hat{\pi }_i(1)= & {} K(\alpha ) \left\{ s_{10} \rho + (1-s_{10}) (1-\rho )\right\} + \left[ 1-K(\alpha )\right] \frac{1}{2} = \frac{1}{2} + K(\alpha ) g(\rho ,s_{10})\; , \\ \hat{\pi }_i(0)= & {} K(\alpha ) \frac{1}{2} + \left[ 1-K(\alpha )\right] \left\{ (1-s_{10}) \rho + s_{10} (1-\rho )\right\} \\= & {} \frac{1}{2} -\left[ 1- K(\alpha )\right] g(\rho ,s_{10}) \; , \end{aligned}$$

with the difference $\hat{\pi }_i(1)-\hat{\pi }_i(0)$ being equal to $g(\rho ,s_{10}) = 2(\rho -\frac{1}{2})(s_{10}-\frac{1}{2})$. In symmetric equilibria, the indifference condition (12) hence boils down to (8), i.e.,

$$\begin{aligned} R(\alpha ,s_{10},\rho )= & {} \hat{u}_i(1,\alpha ,\theta ^H) - \hat{u}_i(0,\alpha ,\theta ^H)= g(\rho ,s_{10}) \theta ^H \\&+\left[ \frac{1}{2} + K(\alpha ) g(\rho ,s_{10})\right] (\alpha -c) =0\; . \end{aligned}$$

Next, identify the viable combinations of $\alpha$ and $s_{10}$. The equilibrium value of $s_{10}$ depends on the expected ability $\hat{a}_i(1)$ for a reform proposal, which is determined by the cutoff $\alpha$. For $\alpha >\underline{a}$, $\hat{a}_i(1)$ is larger than c, so that $s_{10}$ must equal 1. For $\alpha = \underline{a}$, $\hat{a}_i(1)$ is equal to c, so that any $s_{10} \in [0,1]$ is viable. For $\alpha < \underline{a}$, $s_{10}$ is equal to 0.

We are now able to show that there is a unique symmetric equilibrium, which is either of type I or type II. This also proves Corollary 1. We exploit that R is continuous and monotonically increasing in $s_{10}$ and, by Assumption 2, in $\alpha$. For any $\rho \in (1/2,1]$, $R(\underline{a},1/2,\rho ) = (\underline{a} -c)/2 <0$ and $R(c,1,\rho )= g(\rho ,s_{10}) \theta ^H>0$. For any $\rho \in (1/2,1]$, there is hence a unique equilibrium $(\alpha ,s_{10})$ that satisfies $R(\alpha ,s_{10},\rho )=0$. This equilibrium is of type I with $\alpha \in (\underline{a},c)$ and $s_{10}=1$ if $R(\underline{a},1,\rho )<0$. It is of type II with $\alpha =\underline{a}$ and $s_{10}\in (1/2,1]$ if $R(\underline{a},1,\rho )\ge 0$. For $\rho =1/2$, we have $g(1/2,s_{10})=0$ and equilibrium behavior is given by $\alpha =c$ and $s_{10}=1$. Note also that there cannot be equilibria with $\alpha < \underline{a}$. In this case, $s_{10}$ would be equal to 0, so that a reform proposal would give a smaller expected power than the status quo. But then, $\alpha$ must be located above c, which contradicts the assumption that $\alpha < \underline{a}$.

For part (iii), assume without loss of generality that there is an equilibrium with $\alpha _1^H>\alpha _2^H$. This requires that there is an ability level $a' \in (\alpha _2^H,\alpha _1^H)$ such that candidate 1 with type $(a',\theta ^H)$ prefers to propose the status quo, while candidate 2 with the same type prefers to propose the reform. At the same time, a reform proposal by candidate 1 must be associated with a higher expected payoff and, hence, a higher conditional winning probability than a reform proposal by candidate 2. We show by contradiction that such a situation is impossible.

Rearranging (12), $\alpha _1^H>\alpha _2^H$ implies that

$$\begin{aligned} \frac{\hat{\pi }_2(1)- \hat{\pi }_2(0)}{\hat{\pi }_2(1)} =\frac{c-\alpha _2^H}{\theta ^H}> & {} \frac{c-\alpha _1^H}{\theta ^H} = \frac{\hat{\pi }_1(1)- \hat{\pi }_1(0)}{\hat{\pi }_1(1)} \nonumber \\ \Leftrightarrow \hat{\pi }_2(1) \hat{\pi }_1(0)> & {} \hat{\pi }_1(1) \hat{\pi }_2(0)\;. \end{aligned}$$

(13)

We compute $\pi _i(1)$ and $\pi _i(0)$ for $i \in \left\{ 1,2\right\}$. Given $\alpha _1^H>\alpha _2^H\ge \underline{a}$, a reform by candidate 1 has a larger expected payoff than a reform by candidate 2, giving rise to the winning probabilities $s(1,1)=s(1,0)=1$. If both candidates propose the status quo, each wins with $s(0,0)=1/2$ by the tie-breaking rule imposed in Sect. 4.3. For $(x_1,x_2)=(0,1)$, candidate 2 wins with probability $\tilde{s} = 1-s(0,1) \in (0,1]$, where $\tilde{s}=1$ if $\alpha _2^H> \underline{a}$. Hence, the expected power share $\hat{\pi }_i(x_i)$ of candidate i is given by

$$\begin{aligned} \hat{\pi }_1(1)= & {} \rho \; , \\ \hat{\pi }_1(0)= & {} \frac{1}{2}- [1-K(\alpha _2^H)] {\tilde{g}} \; , \\ \hat{\pi }_2(1)= & {} K(\alpha _1^H) \left( \frac{1}{2}+ {\tilde{g}}\right) + \left[ 1-K(\alpha _1^H)\right] (1-\rho ) \; , \\ \hat{\pi }_2(0)= & {} K(\alpha _1^H) \frac{1}{2} + \left[ 1-K(\alpha _1^H)\right] (1-\rho )\; , \end{aligned}$$

where ${\tilde{g}} = 2\left( \rho -\frac{1}{2}\right) \left( {\tilde{s}} - \frac{1}{2}\right) \in \left( 0,\rho -\frac{1}{2}\right]$. Collecting terms, we find that

$$\begin{aligned} \hat{\pi }_2(1) \hat{\pi }_1(0) - \hat{\pi }_1(1) \hat{\pi }_2(0)= & {} \frac{1}{2}K(\alpha _1^H) \left( \frac{1}{2} + {\tilde{g}} - \rho \right) + (1-\rho ) \left[ 1-K(\alpha _1^H)\right] \left( \frac{1}{2}- \rho \right) \\&- {\tilde{g}} \left[ 1-K(\alpha _2^H)\right] \hat{\pi }_2(1) \; , \end{aligned}$$

which is weakly negative because ${\tilde{g}} \in [0,\rho -1/2]$ and $\rho \in [1/2,1]$. This contradicts (13), ruling out asymmetric equilibria with $\alpha _1^H > \alpha _2^H$.

Moreover, there is no asymmetric equilibrium with $\alpha _1^H=\alpha _2^H> \underline{a}$ and $s(1,1) \ne 1/2$. In this case, we would have $\pi _1(1) \ne \pi _2(1)$ and $\pi _1(0) = \pi _2(0)$ at the same time. Then, indifference condition (12) cannot be satisfied at the same cutoff $\alpha _1^H=\alpha _2^H$ for both candidates. $\square$

1.3 Proof of Lemma 1

By Proposition 2, the behavior of the public-spirited candidates does not depend on $\rho$. In contrast, changes in $\rho$ affect the cutoff $\alpha ^H$ of egoistic types, which is implicitly defined by Eq. (8) in the main text. To simplify notation, we denote by $g_\rho$ the derivative of $g(\rho ,s_{10})$ with respect to $\rho$ and suppress the arguments of $g(\rho ,s_{10})$ in the following. Implicit differentiation of (8) gives

$$\begin{aligned}&\frac{\mathrm{d} \alpha ^H}{\mathrm{d} \rho }=-\frac{\frac{\partial R}{\partial \rho }}{\frac{\partial R}{\partial \alpha ^H}}= -\frac{\left[ \theta ^H+(\alpha ^H-c)K(\alpha ^H)\right] g_\rho }{\frac{1}{2}+K(\alpha ^H) g +(\alpha ^H-c) k(\alpha ^H)) g}<0. \end{aligned}$$

In type I equilibria, $g_\rho (\rho ,1)=1$ and $g(\rho ,1) = \rho -1/2$. By equilibrium condition (8), the numerator equals $\frac{c-\alpha ^H}{2 g} g_\rho$, which is strictly positive for all $\rho > \frac{1}{2}$. Under Assumption 2, the denominator is strictly positive as well. Consequently, the overall effect is negative. $\square$

1.4 Proof of Proposition 3

First, the unique equilibrium is always of type I for $\rho =\frac{1}{2}$. In this case, winning and losing the election promises the same amount of power ($g=0$), so that even egoistic candidates care only about their legacy payoff. As a consequence, equilibrium condition (8) is satisfied for $\alpha ^H=c$.

By Lemma 1, the cutoff $\alpha ^H$ strictly decreases with $\rho$. Moreover, implicit differentiation of (8) gives $\frac{\mathrm{d} \alpha ^H}{\mathrm{d} \theta ^H}<0$ and $\frac{\mathrm{d} \alpha ^H}{\mathrm{d} \mu }<0$ for any type I equilibrium with $\rho > \frac{1}{2}$. Two possible cases arise.

Case a: If $\theta ^H < \bar{\theta }(\mu ) = \left[ 1+K\left( \underline{a}\right) \right] \left( c - \underline{a}\right)$, then $R\left( \underline{a}, 1, 1\right) = \frac{1}{4} \theta ^H + \frac{1}{4}\left[ 1 + K\left( \underline{a}\right) \right] \left( \underline{a} - c\right)$$< 0$ is true. Hence, there is a type I equilibrium for all $\rho \in \left[ \frac{1}{2}, 1\right]$. (Note that K depends on $\underline{a}$ as well as $\mu$, the probability to draw an egoistic candidate.)

Case b: If $\theta ^H \ge \bar{\theta }(\mu )$, we get $R\left( \underline{a}, 1, 1\right) \ge 0$ and $R\left( \underline{a}, 1, 1/2\right) = (\underline{a}-c)/2 < 0$. Hence, $R\left( \underline{a}, 1, \rho \right)$ attains negative values if and only if power concentration $\rho$ is sufficiently small. Formally, there is a unique threshold ${\bar{\rho }}(\theta ^H, \mu )$ such that, if and only if $\rho < {\bar{\rho }}(\theta ^H, \mu )$, $R\left( \underline{a}, 1, \rho \right) <0$ is true and a type I equilibrium exists.

Finally, the derivative of $\bar{\theta }(\mu )$ is given by $\bar{\theta }'(\mu )= \left[ \varPhi \left( \underline{a}\right) - \varPhi (c) \right] \left( c - \underline{a}\right) <0$. $\square$

1.5 Proof of Proposition 4

1.5.1 Unique maximum

We measure welfare by the voter’s expected utility from ex ante perspective. As explained in the main text, this is given by

$$\begin{aligned} W(\rho ) = 2 \left[ s^R \rho + (1-s^R) (1-\rho )\right] B(\alpha ^H). \end{aligned}$$

A reforming candidate wins the election with probability $s_{10}$ if her opponent proposes the status quo and with probability $s(1,1)=1/2$ if her opponent proposes a reform as well. As a consequence, the winning probability $s^R$ is given by

$$\begin{aligned} s^R= K(\alpha ^H)s_{10}+\frac{1-K(\alpha ^H)}{2}. \end{aligned}$$

Inserting this probability and our definition of $g\left( \rho , s_{10}\right)$, voter welfare is given by

$$\begin{aligned} W(\rho )&= 2 \left[ \frac{1}{2}+K(\alpha ^H) ~g\left( \rho , s_{10}\right) \right] \\&\quad \times \left[ \underbrace{(1-\mu ) \int _{c}^1 \phi (a)(a-c) \mathrm{d}a+ \mu \int _{\alpha ^H}^1 \phi (a)(a-c) \mathrm{d}a}_{z(\alpha ^H)} \right] . \end{aligned}$$

First, note that $z\left( \underline{a}\right) = 0$, and $z\left( \alpha \right) > 0$ for all $\alpha > \underline{a}$ by the construction of $\underline{a}$. Hence, welfare is strictly positive in all type I equilibria and equals zero in all type II equilibria. We conclude that the welfare-maximizing level of power concentration satisfies $\rho ^*< {\bar{\rho }}(\theta ^H, \mu )$, where the latter is strictly larger than $\frac{1}{2}$. Hence, the welfare maximizing $\rho ^*$ always gives rise to a type I equilibrium.

Second, we show that the welfare function is strictly quasi-concave in $\rho$ for type I equilibria, $\rho < {\bar{\rho }}(\theta ^H, \mu )$, where $s_{10}= 1$ and $\alpha ^H$ is implicitly defined by (8). In a type I equilibrium, the derivative of W with respect to $\rho$ is given by

$$\begin{aligned} \frac{\mathrm{d} W}{\mathrm{d} \rho }&= \frac{\partial W}{\partial \rho } + \frac{\partial W}{\partial \alpha ^H} \frac{\mathrm{d} \alpha ^H}{\mathrm{d} \rho } = K(\alpha ^H) z(\alpha ^H) \\&\quad + \left\{ (c-\alpha ^H)k(\alpha ^H) \left( \frac{1}{2}+ K(\alpha ^H) g\right) + z(\alpha ^H) k(\alpha ^H) g \right\} \frac{\mathrm{d} \alpha ^H}{\mathrm{d} g} \\&= \frac{1}{D} \left\{ K(\alpha ^H) z(\alpha ^H) \left[ \frac{1}{2}+ (K(\alpha ^H)+(\alpha ^H-c) k(\alpha ^H))g\right] \right. \\&\quad - \left[ (c-\alpha ^H)k(\alpha ^H) \left( \frac{1}{2}+ K(\alpha ^H) g\right) + z(\alpha ^H) k(\alpha ^H) g \right] \\&\quad \left. \left[ \theta ^H + K(\alpha ^H) (\alpha ^H-c)\right] \right\} \\&= \frac{1}{D} \left\{ K(\alpha ^H) z(\alpha ^H) \left[ \frac{1}{2}+ K(\alpha ^H)g\right] -(c-\alpha ^H)k(\alpha ^H) \left( \frac{1}{2}+ K(\alpha ^H) g\right) \right. \\&\quad \left. \left[ \theta ^H + K(\alpha ^H) (\alpha ^H-c)\right] - z(\alpha ^H) k(\alpha ^H) g \theta ^H \right\} \\&= \frac{1}{D} \left\{ \left[ K(\alpha ^H)- k(\alpha ^H) (c-\alpha ^H)\right] \frac{W(\rho )}{2} -k(\alpha ^H)(c-\alpha ^H) \frac{\theta ^H}{2}\right\} , \end{aligned}$$

where $D>0$ denotes the denominator of $\frac{\mathrm{d} \alpha ^H}{\mathrm{d} \rho }$ and $k(\alpha ^H) :=\mu \phi (\alpha ^H)$. Hence, the term in brackets has to equal zero in every extremum of W in the interval $\left( \frac{1}{2}, 1\right)$. Recall that $W(\rho )>0$ in all type I equilibria, and note that $\frac{\mathrm{d} W}{\mathrm{d} \rho }$ is strictly positive for $\rho =\frac{1}{2}$ (where $\alpha ^H=c$). The necessary condition for an extremum can be rearranged to read

$$\begin{aligned} h(\rho ) :=\frac{K(\alpha ^H)}{k(\alpha ^H)(c-\alpha ^H)} -\left( 1 + \frac{\theta ^H}{ W(\rho )}\right) = 0. \end{aligned}$$

Note that h is continuous in $\rho$ and that the sign of $\frac{\mathrm{d} W}{\mathrm{d} \rho }$ is identical to the sign of h. Under Assumption 3, its first term is strictly increasing in $\alpha ^H$ and, consequently, decreasing in $\rho$. The second term is constant in every extreme value of W (root of h). Hence, h is strictly decreasing in $\rho$ in each root. We conclude that h has at most one root and that the welfare function has at most one maximum and no minimum in $\left[ \frac{1}{2}, {\bar{\rho }}(\theta ^H, \mu \right) )$. Recalling that $W(\rho )=0$ in all type II equilibria, this implies that W is globally quasi-concave and has a unique maximum in $\left[ \frac{1}{2}, 1\right]$.

1.5.2 Optimality of power dispersion

Proposition 4 states that some power dispersion is optimal if and only if $\theta ^H$ exceeds a unique threshold ${\tilde{\theta }}(\mu ) < \bar{\theta }(\mu )$.

First, full concentration of power is optimal if and only if $h(1) \ge 0$. This is true for $\theta ^H \rightarrow 0$, where $\alpha ^H=c$. The derivative of h in $\theta ^H$ is given by

$$\begin{aligned} \frac{\mathrm{d} h}{\mathrm{d} \theta ^H}=\frac{\partial h}{\partial \theta ^H} + \frac{\partial h}{\partial \alpha ^H} \frac{ \mathrm{d}\alpha ^H}{\mathrm{d}\theta ^H}. \end{aligned}$$

The first term is strictly negative, and the same is true for $\frac{\mathrm{d} \alpha ^H}{\mathrm{d} \theta ^H}$ in every type I equilibrium. Under Assumption 3, h is strictly increasing in $\alpha ^H$. Hence, the derivative $\frac{\mathrm{d} h}{\mathrm{d} \theta ^H}$ is strictly negative in every type I equilibrium. We conclude that there is at most one threshold $\tilde{\theta }(\mu )>0$ such that $h(1) = 0$ if $\theta ^H = {\tilde{\theta }} (\mu )$ and $h(1) < 0$ if and only if $\theta ^H < {\tilde{\theta }} (\mu )$.

Second, note that $W(\frac{1}{2})>0$ while $W(\rho )=0$ for all $\rho \ge {\bar{\rho }}(\theta ^H,\mu )$. For values of $\theta ^H$ such that full power concentration induces a type II equilibrium, full concentration of power can hence not be optimal. By continuity, the same holds for levels of $\theta ^H$ slightly smaller than $\bar{\theta }(\mu )$. Hence, the threshold ${\tilde{\theta }}(\mu )$ for the optimality of power dispersion is strictly below $\bar{\theta }(\mu )$.

Third, implicit differentiation of the threshold ${\tilde{\theta }}$ with respect to $\mu$ gives

$$\begin{aligned} {\tilde{\theta }}'(\mu )= -\left. \frac{\frac{\mathrm{d} h(1)}{\mathrm{d} \mu }}{\frac{\mathrm{d} h(1)}{\mathrm{d} \theta }}\right| _{\theta ={\tilde{\theta }}(\mu )}. \end{aligned}$$

We have shown that full power concentration can only be optimal if it induces a type I equilibrium. Hence, $\left. \frac{\mathrm{d} h(1)}{\mathrm{d} \theta }\right| _{\theta ={\tilde{\theta }}(\mu )}$ is strictly negative as argued above. Moreover, $\frac{\mathrm{d} h(\rho )}{\mathrm{d} \mu }$ is given by

$$\begin{aligned} \frac{\mathrm{d} h(\rho )}{\mathrm{d} \mu }= \frac{\partial h(\rho )}{\partial \mu } + \frac{\partial h(\rho )}{\partial \alpha ^H} \frac{\mathrm{d} \alpha ^H}{\mathrm{d} \mu }. \end{aligned}$$

This comprises a direct effect and an indirect effect of $\mu$ on the level of $h(\rho )$. The direct effect is given by

$$\begin{aligned} \frac{\partial h(\rho )}{\partial \mu }&= - \frac{\varPhi (c)}{ k\left( \alpha ^H\right) \left( c- \alpha ^H\right) } + \frac{\theta ^H}{W(\rho )^2} \frac{\partial W(\rho )}{\partial \mu } \\&<\frac{\theta ^H}{W(\rho )^2}\left[ 2 K_\mu \left( \alpha ^H\right) g z\left( \alpha ^H\right) + 2 \left[ \frac{1}{2}+K(\alpha ^H)g\right] z_\mu \left( \alpha ^H\right) \right] < 0 \end{aligned}$$

The negative sign follows since $K_{\mu }(\alpha ^H)= \varPhi \left( \alpha ^H\right) - \varPhi \left( c\right) <0$ and $z_{\mu }(\alpha ^H)= \int _{\alpha ^H}^c \phi (a) (a-c) \mathrm{d}a <0$.

With respect to the indirect effect, implicit differentiation of (8) gives

$$\begin{aligned} \frac{\mathrm{d} \alpha ^H}{\mathrm{d} \mu } = - \frac{K_{\mu }(\alpha ^H)g(\alpha ^H- c)}{\frac{1}{2} + K\left( \alpha ^H\right) g + k\left( \alpha ^H\right) \left( \alpha ^H-c\right) g} < 0. \end{aligned}$$

As h is strictly increasing in $\alpha ^H$ as argued before, the indirect effect of $\mu$ on h is negative as well. Hence, the same is true for the derivative of h with respect to $\mu$ in every type I equilibrium.

Altogether, we find that ${\tilde{\theta }}'(\mu )<0$. Hence, if the share of egoistic candidates is increased, this decreases the level of egoism ${\tilde{\theta }}(\mu )$ up to which full power concentration is optimal.

1.5.3 Comparative statics of $\rho ^*$

Finally, we show that the optimal level $\rho ^*$ is strictly decreasing in $\theta ^H$ and $\mu$ whenever some power dispersion is optimal, i.e., when $\theta ^H > {\tilde{\theta }}(\mu )$. In this case, the optimal level of power concentration is implicitly defined by $h(\rho ^*)=0$.

With respect to $\theta ^H$, implicit differentiation of h gives

$$\begin{aligned} \frac{\mathrm{d} \rho ^*}{\mathrm{d} \theta ^H }= -\left. \frac{\frac{\mathrm{d} h(\rho )}{\mathrm{d} \theta ^H}}{\frac{\mathrm{d} h(\rho )}{\mathrm{d} \rho }}\right| _{\rho =\rho ^*}. \end{aligned}$$

Above, we have shown that the numerator $\frac{\mathrm{d} h(\rho )}{\mathrm{d} \theta ^H}$ is strictly negative. The denominator is strictly negative as well, as h is strictly decreasing in $\rho$ in every root. Thus, the optimal level $\rho ^*$ is strictly decreasing in $\theta ^H$.

With respect to $\mu$, implicit differentiation of h gives

$$\begin{aligned} \frac{\mathrm{d} \rho ^*}{\mathrm{d} \mu }= -\left. \frac{\frac{\mathrm{d} h(\rho )}{\mathrm{d} \mu } }{\frac{\mathrm{d} h(\rho )}{\mathrm{d} \rho }}\right| _{\rho =\rho ^*}. \end{aligned}$$

As shown above, both numerator and denominator of this expression are strictly negative. Hence, the same is true for the entire derivative $\frac{\mathrm{d} \rho ^*}{\mathrm{d} \mu }$. $\square$

Graphical illustration of welfare effects

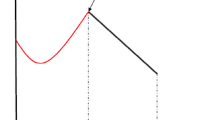
The following figure illustrates for a numerical example how the sizes of the positive empowerment effect and the negative effects (disciplining, selection) on voter welfare change with the concentration of political power $\rho$ (Fig. 3).

Fig. 3

The welfare effects of a change in $\rho$. The solid line represents the (positive) empowerment effect; the dashed line represents the sum of the (negative) disciplining and selection effects. The optimal level of $\rho$ is attained at the intersection of both lines. Parameters: uniform ability distribution, $c=0.6$, $\mu =0.8$, $\theta ^H=0.6$

Empirical illustration

Our model makes novel statements about the welfare effect of power concentration and its dependence on the conflict of interest between voters and politicians. These results give rise to empirically testable predictions under the assumption that real-world institutions have not been chosen optimally (according to our model). This assumption seems plausible if institutional settings and the implied levels of power concentration in the real world differ mainly for historical reasons such as the predominant views at the time of constitutional drafting, the settings in neighboring countries or their colonial heritage.²⁵ Under this assumption, Corollary 2 predicts an interaction between power concentration and the conflict of interest between voters and politicians summarized by the following hypothesis.

Hypothesis

The effect of power concentration on welfare depends on the conflict of interest between voters and politicians. Power concentration has positive effects on welfare if the conflict of interest is low. In contrast, if the conflict of interest is high, the welfare effect of power concentration is significantly smaller or negative.

Ideally, we would like to test this hypothesis empirically for a large set of countries. Unfortunately, we face a restriction to data availability. A focus on meaningful variations in institutional settings and in politicians’ motivation requires a cross-country analysis, but measures for our key variables are only available for some established democracies. We nevertheless propose an empirical strategy to illustrate the consistency of our model predictions with the data.

1.1 Operationalization

The empirical analysis is based on three key variables. The dependent variable is a measure of efficient policies. The two major independent variables are the degree of power concentration within the political system and the conflict of interest between voters and politicians. In this subsection, we present the operationalization of our main empirical model. The analysis is followed by several robustness checks in which we show that our results survive the use of alternative operationalizations.

As a measure for efficient policies, we use growth in real GDP per capita in constant 2005 US $ as provided by the World Bank (2014). It provides a concise and objective measure of developments that bear the potential of welfare improvements. We measure the concentration of power within a political system by Lijphart’s index of the executive-parties dimension (Lijphart 1999). This well-established measure quantifies how easily a single party can take complete control of the government. The index is based on the period 1945–1996 and is available for 36 countries. The conflict of interest between voters and politicians cannot be measured objectively. However, indication for it may come from voter surveys. The International Social Survey Programme includes questions on voters’ opinions about politicians. In its 2004 survey, conducted in 38 countries, it included the item “Most politicians are in politics only for what they can get out of it personally” (ISSP Research Group 2012). Agreement with this statement was coded on a five-point scale. We use mean agreement in a country as our measure for the conflict of interest between voters and politicians.

We normalize the indices for both power concentration and the conflict of interest to range between zero and one. High values indicate a strong concentration of political power or a strong conflict of interest of politicians, respectively.

1.2 Design

Data on both indices are available for 20 countries. Of these countries, New Zealand underwent major constitutional changes after 1996. As these changes are not captured by the Lijphart index, we have to exclude New Zealand from the analysis. As our model focuses on established democracies, we require that countries have a Polity IV Constitutional Democracy index (Marshall and Jaggers 2010) of at least 95 in the year 2002. This excludes Venezuela from the sample. The remaining 18 countries are similar with respect to their economic characteristics.²⁶ They are economically highly developed (World Bank) and feature a Human Development Index (HDI) of at least 0.9. None of the exclusions changes the qualitative results of the analysis.

We find no correlation between power concentration and the conflict of interest (Pearson’s correlation coefficient $\rho =0.199$, $p=0.428$). Technically, this means that the analysis will not suffer from multicollinearity and that the hypothesis can be tested by a linear regression model even though the welfare function of our model is nonlinear in power concentration.²⁷

The time-invariant dependent variables require a cross-section analysis. All explanatory variables correspond to 2004 or earlier years. To address potential problems of reversed causality, our explained variable captures growth after 2004. To test whether the welfare effect of power concentration varies with the conflict of interest, we include an interaction term between power concentration and the conflict of interest in the regression. We control for variables that may be correlated with both our explanatory variables and our explained variable. Most notably, past economic performance affects growth (see, e.g., Sala-i-Martin 1994) and may alter voters’ perception of politicians. We hence control for GDP per capita in 2004. Growth is also affected by other variables, such as capital accumulation, school enrollment rates, life expectancy or openness of the economy (see, e.g., Sala-i-Martin 1997). To capture these influences and to keep the number of explanatory variables low, we add past growth in real GDP per capita (from 1991 to 2004) to the regression.

1.3 Results

For a first glimpse of the data, we split the country set at the median value of the conflict of interest between voters and politicians. Figure 4 shows how growth is related to the concentration of power for the two sets of countries. The left panel contains countries with a small conflict of interest, while the right panel contains countries with a large conflict of interest. The figure shows that power concentration is only weakly related to growth if the conflict of interest is small, whereas power concentration is negatively related to growth if the conflict of interest is large.

Fig. 4

Relationship between power concentration and growth

For the analysis of the relationship between power concentration and economic growth, we use the conflict of interest as a continuous explanatory variable in an OLS regression and control for relevant covariates. Table 1 presents the regression results.

Column (a) displays the results of a regression model without interaction term. In this regression, the coefficient of power concentration estimates the effect on economic growth under the assumption that this effect does not depend on the conflict of interest between voters and politicians. We find that this coefficient is insignificant.

This picture changes if the interplay between power concentration and the conflict of interest is taken into account. Column (b) displays the results of a regression model with an interaction term between power concentration and the conflict of interest. Most importantly, the coefficient of the interaction term is negative and significant. Thus, power concentration is more negatively related to growth if the conflict of interest between voters and politicians is large. The inclusion of the interaction term in the regression also strongly increases the explanatory power of the econometric model. The adjusted $R^2$ increases from 0.13 to 0.42.

These results suggest that the welfare effect of power concentration depends strongly on the conflict of interest. The conditional effect of power concentration at the smallest and the largest level of conflict of interest in our country set are reported in Table 2. At the smallest level of conflict, power concentration is positively related to growth. At the largest level of conflict, in contrast, power concentration is negatively related to growth. Our analysis thus leads to the following result.

Result

The higher the conflict of interest between voters and politicians is, the more negative the relation between power concentration and growth is. Furthermore, power concentration is negatively related to growth if the conflict of interest is high and positively related to growth if the conflict of interest is low.

We conclude that the data are in line with our model. While the evidence is only suggestive, it indicates that the effect of power-concentrating institutions depends on the specific conditions of a country. The direction and the size of the effect seem to depend on the conflict of interest between voters and politicians, as predicted by our model. Our model delivers a plausible explanation for the pattern in the data: Countries with a small conflict of interest benefit from power concentration as it helps them empower better candidates; countries with a large conflict of interest suffer from power concentration as it induces more inefficient reforms and worse selection of candidates. While the data support our theoretically derived hypothesis, we are unable to test the explanation provided by our model against alternative explanations.

Table 1 OLS regression results

Table 2 Effect of power concentration

1.4 Robustness

The specification used above is parsimonious and may give raise to a concern of omitted variable bias. However, for an omitted variable to bias the coefficient of the interaction effect, it would have to be correlated with the interaction term between power concentration and the conflict of interest, and with growth potential. The most obvious candidate for an omitted variable is general trust among the population. Trust may be correlated with the perception of politicians’ motivation and may reduce opposition toward power concentration. If trust had a nonlinear effect on growth, in addition, the absence of a quadratic trust term would bias the coefficient of the interaction effect between power concentration and the conflict of interest. We control for this possibility and add general trust, as measured by the 2004 ISSP survey,²⁸ as linear and quadratic term to our regression. This does not change our result.

To further confirm robustness of the result, we check whether the negative and significant interaction term between power concentration and the conflict of interest is robust to the use of different measures for our key variables. For any alternative model specification, we provide the p-value of the interaction term and the F-statistic of the regression model in parenthesis. As alternative measures for power concentration, we use a more recent index by Armingeon et al. (2011) ($p=0.013$, $F=5.75$, $N=17$) as well as its modified version that focuses on institutional factors only ($p=0.011$, $F=6.87$, $N=17$). We get similar results if we use a modified index for the executive-parties dimension suggested by Ganghof and Eppner (2019) that focuses on the clarity of responsibility and accountability ($p=0.005$, $F=4.10$, $N=19$), the index for checks and balances (Keefer and Stasavage 2003, $p=0.019, F=16.12, N=18$) and the political constraint (POLCON) index (Henisz 2006, $p=0.060, F=15.60, N=18$). For the nine-categorical type of electoral system (IDEA 2004), the coefficient of the interaction term has the same sign, but becomes insignificant ($p=0.216, F=5.32, N=18$).

The interaction term is also of the expected sign and remains significantly different from zero, if we measure the conflict of interest between voters and politicians by the belief that politicians are more interested in votes than peoples’ opinions, elicited in the ESS (2002, $p=0.020$, $F=7.12$, $N=17$) or the Corruption Perception Index (Transparency International 2004, $p=0.005$, $F=7.86$, $N=18$). Using trust in political parties from the Eurobarometer, however, yields an insignificant interaction term (European Commission 2012, $p=0.173$, $F=1.38$, $N=16$).

Finally, one might fear that our result is influenced by the financial crisis, which affected output beginning in 2008. To test whether this is the case, we may exclude from the sample the countries that were hit hardest by the financial crisis. The result is robust to the exclusion of any subset of the countries Ireland, Spain and Portugal (all p-values $<0.085$, $F>2.53$).