Abstract
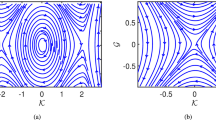
In this paper, elastic wave propagation in a one-dimensional micromorphic medium characterized by two internal variables is investigated. The evolution equations are deduced following two different approaches, namely using: (i) the balance of linear momentum and the Clausius–Duhem inequality, and (ii) an assumed Lagrangian functional (including a gyroscopic coupling) together with a variational principle. The dispersion relation is obtained and the possibility of the emerging band gaps is shown in such microstructured materials. Some numerical simulations are also performed in order to highlight the dispersive nature of the material under study.
Similar content being viewed by others
References
Alessandroni, S., dell’Isola, F., Porfiri, M.: A revival of electric analogs for vibrating mechanical systems aimed to their efficient control by PZT actuators. Int. J. Solids Struct. 39(20), 5295–5324 (2002)
Alibert, J.J., Seppecher, P., dell’Isola, F.: Truss modular beams with deformation energy depending on higher displacement gradients. Math. Mech. Solids 8(1), 51–73 (2003)
Altenbach, H., Eremeyev, V.A.: On the constitutive equations of viscoelastic micropolar plates and shells of differential type. Math. Mech. Complex Syst. 3(3), 273–283 (2015)
Andrianov, I.V., Bolshakov, V.I., Danishevs’kyy, V.V., Weichert, D.: Higher order asymptotic homogenization and wave propagation in periodic composite materials. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 464(2093), 1181–1201 (2008)
Arnol’d, V.I.: Mathematical Methods of Classical Mechanics. Springer, Berlin (2013)
Askes, H., Metrikine, A.V., Pichugin, A.V., Bennett, T.: Four simplified gradient elasticity models for the simulation of dispersive wave propagation. Philos. Mag. 88(28–29), 3415–3443 (2008)
Auffray, N., dell’Isola, F., Eremeyev, V.A., Madeo, A., Rosi, G.: Analytical continuum mechanics à la Hamilton–Piola least action principle for second gradient continua and capillary fluids. Math. Mech. Solids 20(4), 375–417 (2015)
Berezovski, A., Engelbrecht, J., Berezovski, M.: Waves in microstructured solids: a unified viewpoint of modeling. Acta Mech. 220(1–4), 349–363 (2011)
Berezovski, A., Engelbrecht, J., Maugin, G.A.: Generalized thermomechanics with dual internal variables. Arch. Appl. Mech. 81(2), 229–240 (2011)
Bertram, A., Glüge, R.: Gradient materials with internal constraints. Math. Mech. Complex Syst. 4(1), 1–15 (2016)
Biswas, R., Poh, L.H.: A micromorphic computational homogenization framework for heterogeneous materials. J. Mech. Phys. Solids 102, 187–208 (2017)
Bloch, A.: XXXVIII: A new approach to the dynamics of systems with gyroscopic coupling terms. Lond. Edinb. Dublin Philos. Mag. J. Sci. 35(244), 315–334 (1944)
Born, M., Huang, K.: Dynamical Theory of Crystal Lattices. Oxford University Press, Oxford (1954)
Boutin, C., dell’Isola, F., Giorgio, I., Placidi, L.: Linear pantographic sheets: asymptotic micro-macro models identification. Math. Mech. Complex Syst. 5(2), 127–162 (2017)
Brillouin, L.: Wave Propagation in Periodic Structures: Electric Filters and Crystal Lattices. Dover Publications, Mineola (1946)
Capriz, G.: Continua with Microstructure. Springer, Berlin (1989)
Chen, W., Fish, J.: A dispersive model for wave propagation in periodic heterogeneous media based on homogenization with multiple spatial and temporal scales. Trans. ASME J. Appl. Mech. 68(2), 153–161 (2001)
Crandall, S.H.: Dynamics of Mechanical and Electromechanical Systems. McGraw-Hill, New York (1968)
De Masi, A., Merola, I., Presutti, E., Vignaud, Y.: Potts models in the continuum. Uniqueness and exponential decay in the restricted ensembles. J. Stat. Phys. 133(2), 281–345 (2008)
dell’Isola, F., Andreaus, U., Placidi, L.: At the origins and in the vanguard of peridynamics, non-local and higher-gradient continuum mechanics: an underestimated and still topical contribution of Gabrio Piola. Math. Mech. Solids 20(8), 887–928 (2015)
dell’Isola, F., Corte, A.D., Giorgio, I.: Higher-gradient continua: the legacy of Piola, Mindlin, Sedov and Toupin and some future research perspectives. Math. Mech. Solids 22(4), 852–872 (2017)
dell’Isola, F., Cuomo, M., Greco, L., Della Corte, A.: Bias extension test for pantographic sheets: numerical simulations based on second gradient shear energies. J. Eng. Math. 103(1), 127–157 (2017)
dell’Isola, F., Della Corte, A., Esposito, R., Russo, L.: Some cases of unrecognized transmission of scientific knowledge: from antiquity to Gabrio Piola’s peridynamics and generalized continuum theories. In: Generalized Continua as Models for Classical and Advanced Materials, pp. 77–128. Springer (2016)
dell’Isola, F., Seppecher, P., Della Corte, A.: The postulations á la D’Alembert and á la Cauchy for higher gradient continuum theories are equivalent: a review of existing results. Proc. R. Soc. A 471(2183), 20150,415 (2015)
dell’Isola, F., Steigmann, D., Della Corte, A.: Synthesis of fibrous complex structures: designing microstructure to deliver targeted macroscale response. Appl. Mech. Rev. 67(6), 060,804 (2015)
Duhem, P.: Sauver les phénomènes. Essai sur la notion de théorie physique de Platon à Galilée. Sozein ta phainomena (2005)
Eremeyev, V.A., Pietraszkiewicz, W.: Material symmetry group and constitutive equations of micropolar anisotropic elastic solids. Math. Mech. Solids 21(2), 210–221 (2016)
Eringen, A.C., Suhubi, E.S.: Nonlinear theory of simple micro-elastic solids I. Int. J. Eng. Sci. 2(2), 189–203 (1964)
Eugster, S.R., dell’Isola, F.: An ignored source in the foundations of continuum physics “Die Allgemeinen Ansätze der Mechanik der Kontinua” by E. Hellinger. In: Proceedings in Applied Mathematics and Mechanics (2017)
Eugster, S.R., et al.: Exegesis of the introduction and sect. I from “Fundamentals of the mechanics of continua” by E. Hellinger. ZAMM J. Appl. Math. Mech. (Z. Angew. Math. Mech.) 97(4), 477–506 (2017)
Eugster, S.R., et al.: Exegesis of sect. II and III. A from “Fundamentals of the mechanics of continua” by E. Hellinger. ZAMM J. Appl. Math. Mech. (Z. Angew. Math. Mech.) 98(1), 31–68 (2018)
Eugster, S.R., et al.: Exegesis of sect. III. B from “Fundamentals of the mechanics of continua” by E. Hellinger. ZAMM J. Appl. Math. Mech. (Z. Angew. Math. Mech.) 98(1), 69–105 (2018)
Fish, J., Chen, W.: Higher-order homogenization of initial/boundary-value problem. J. Eng. Mech. 127(12), 1223–1230 (2001)
Fish, J., Kuznetsov, S.: From homogenization to generalized continua. Int. J. Comput. Methods Eng. Sci. Mech. 13(2), 77–87 (2012)
Forest, S., Sab, K.: Cosserat overall modeling of heterogeneous materials. Mech. Res. Commun. 25(4), 449–454 (1998)
Geers, M.G., Kouznetsova, V.G., Brekelmans, W.: Multi-scale computational homogenization: trends and challenges. J. Comput. Appl. Math. 234(7), 2175–2182 (2010)
Giorgio, I., Culla, A., Del Vescovo, D.: Multimode vibration control using several piezoelectric transducers shunted with a multiterminal network. Arch. Appl. Mech. 79(9), 859 (2009)
Giorgio, I., Della Corte, A., dell’Isola, F.: Dynamics of 1D nonlinear pantographic continua. Nonlinear Dyn. 88(1), 21–31 (2017)
Goldstein, H.: Classical Mechanics. Addison-Wesley, Boston, United States (1965)
Grimmett, G.R.: Correlation inequalities for the potts model. Math. Mech. Complex Syst. 4(3–4), 327–334 (2016)
Harrison, P.: Modelling the forming mechanics of engineering fabrics using a mutually constrained pantographic beam and membrane mesh. Compos. A Appl. Sci. Manuf. 81, 145–157 (2016)
Lagrange, J.L.: Mécanique Analytique, vol. 1. Mallet-Bachelier, Paris (1853)
Mariano, P.M.: Multifield theories in mechanics of solids. Adv. Appl. Mech. 38, 1–93 (2001)
Maugin, G.A.: Infernal variables and dissipative structures. J. Non-equilib. Thermodyn. 15(2), 173–192 (1990)
Maugin, G.A.: Material Inhomogeneities in Elasticity. CRC Press, Boca Roton (1993)
Maugin, G.A.: On the thermomechanics of continuous media with diffusion and/or weak nonlocality. Arch. Appl. Mech. 75(10–12), 723–738 (2006)
Maugin, G.A.: The saga of internal variables of state in continuum thermo-mechanics (1893–2013). Mech. Res. Commun. 69, 79–86 (2015)
Maugin, G.A., Muschik, W.: Thermodynamics with internal variables. Part I. General concepts. J. Non-equilib. Thermodyn. 19, 217–249 (1994)
Millet, O., Hamdouni, A., Cimetière, A.: A classification of thin plate models by asymptotic expansion of non-linear three-dimensional equilibrium equations. Int. J. Non-linear Mech. 36(1), 165–186 (2001)
Milton, G.W., Briane, M., Harutyunyan, D.: On the possible effective elasticity tensors of 2-dimensional and 3-dimensional printed materials. Math. Mech. Complex Syst. 5(1), 41–94 (2017)
Mindlin, R.D.: Micro-structure in linear elasticity. Arch. Ration. Mech. Anal. 16(1), 51–78 (1964)
Misra, A., Lekszycki, T., Giorgio, I., Ganzosch, G., Müller, W.H., dell’Isola, F.: Pantographic metamaterials show atypical Poynting effect reversal. Mech. Res. Commun. 89, 6–10 (2018)
Misra, A., Poorsolhjouy, P.: Identification of higher-order elastic constants for grain assemblies based upon granular micromechanics. Math. Mech. Complex Syst. 3(3), 285–308 (2015)
Mura, T.: Micromechanics of Defects in Solids. Springer, Berlin (1987)
Neff, P., Ghiba, I.D., Madeo, A., Placidi, L., Rosi, G.: A unifying perspective: the relaxed linear micromorphic continuum. Continuum Mech. Thermodyn. 26(5), 639–681 (2014)
Nemat-Nasser, S., Hori, M.: Micromechanics: Overall Properties of Heterogeneous Materials. Elsevier, New York (1993)
Pfeuty, P.: The one-dimensional Ising model with a transverse field. Ann. Phys. 57(1), 79–90 (1970)
Porfiri, M., dell’Isola, F., Santini, E.: Modeling and design of passive electric networks interconnecting piezoelectric transducers for distributed vibration control. Int. J. Appl. Electromagn. Mech. 21(2), 69–87 (2005)
Turco, E., dell’Isola, F., Cazzani, A., Rizzi, N.L.: Hencky-type discrete model for pantographic structures: numerical comparison with second gradient continuum models. Z. Angew. Math. Phys. 67(4), 85 (2016)
Turco, E., Giorgio, I., Misra, A., dell’Isola, F.: King post truss as a motif for internal structure of (meta) material with controlled elastic properties. R. Soc. Open Sci. 4(10), 171,153 (2017)
Ván, P., Berezovski, A., Engelbrecht, J.: Internal variables and dynamic degrees of freedom. J. Non-equilib. Thermodyn. 33(3), 235–254 (2008)
Vinogradov, A.M., Kupershmidt, B.A.: The structures of Hamiltonian mechanics. Russ. Math. Surv. 32(4), 177 (1977)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Francesco dell’Isola.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Berezovski, A., Yildizdag, M.E. & Scerrato, D. On the wave dispersion in microstructured solids. Continuum Mech. Thermodyn. 32, 569–588 (2020). https://doi.org/10.1007/s00161-018-0683-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00161-018-0683-1