Abstract
This paper develops a unified model of dual and unitary job holding based on a Stone-Geary utility function. The model incorporates both constrained and unconstrained labor supply. Panel data methods are adapted to accommodate unobserved heterogeneity and multinomial selection into six mutually exclusive labor supply regimes. We estimate the wage and income elasticities arising from selection and unobserved heterogeneity as well as from the Stone-Geary Slutsky equations. The labor supply model is estimated with data from the British Household Panel Survey 1991–2008. Among dual job holders, our study finds that the Stone-Geary income and wage elasticities are much larger for labor supply to the second job compared with the main job. When the effects of selection and unobserved heterogeneity are taken account of, the magnitudes of these elasticities on the second job tend to be significantly reduced.



Similar content being viewed by others
Notes
Typically, the Stone-Geary utility function is used to estimate expenditure functions for multiple commodity groups. See Chung (1994) for a review of the main studies based on a Stone-Geary utility function.
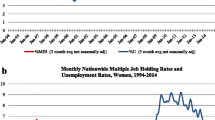
In fact, the male participation rate in our sample is 90% while the same rate for women is only 74%.
This result is consistent with Panos et al. (2011) which finds that workers who have a lower degree of transferability on the main job are more likely to choose the same occupation in their second job
In the present context, the available price and quantity information is disaggregated for jobs but not for commodities.
In the empirical section, we introduce a multinomial selection process that accounts for the fact that the potential wage on the second job for unitary job holders is unobservable to us.
This assumption is supported by the data. Typically, the hours constraint is generated by institutional labor laws which force firms to pay overtime above a certain number of hours worked per week. For the UK, this cut off is set at 41 gross hours (36 net hours) per week. Figure 3 reveals that the mode of the distribution of the hours worked on the first job is around the institutional standard hours while the distribution of the hours on the second job is always below that cut off. As such, the institutional standard hours seem to be binding for the first job but not for the second job.
We also ran our estimation for the sample of men aged 25 to 65 to avoid the standard problems associated with selection into college. However, we obtain results virtually identical to those reported in this paper. Results are available from the authors upon request.
8 O u r a d j u s t m e n t f o r t a x e s i s a s i m p l e a p p r o x i m a t i o n t h a t t r e a t s m a r g i n a l r a t e s a s p r o p o r t i o n a l i n o r d e r t o o b t a i n l o c a l a f t e r t a x w a g e r a t e s a n d i n c o m e.H e n c e , o u r a n a l y s i s d o e s n o t a t t e m p t t o i n c o r p o r a t e t h e k i n k e d b u d g e t a r i s i n g f r o m a g r a d u a t e d i n c o m e t a x s y s t e m.
This conclusion is in line with the results in Wu et al. (2009), although their argument is based on the fact that the wage on job 2 is larger than the wage on job 1.
“A” level education represents 13 years of education/upper secondary school.
An alternative modeling strategy to sample selection would be nested logit. Conditional on whether or not one is constrained on their first job, an individual chooses between holding one job and holding two jobs. One challenge is that with our data it would be difficult to find plausible variables reflecting choice specific characteristics.
Estimated standard errors reported in the paper are bootstrap estimates from 200 replications that account for all estimation steps, including the estimation of multinomial logit regression and boundary parameters.
For the sample mean values used to correct for unobserved heterogeneity in the labor supply equations, we average only over the time-series for which the individual was in the particular labor supply regime.
As a robustness check, we trimmed the top 1% of the sample hours distribution in order to change our hours boundary parameters. Overall, the changes in the boundary values are small to nonexistent. Although the sign changes on the total own wage elasticity on the second job for underemployed dual job holders, this does not alter the conclusion that labor supply to the second job is very inelastic for this class of workers
Our predicted weekly labor supply responses reflect the direct effects of changes in the wage rate for job 1 and non-labor income on the probability of switching between regimes. In this application, however, we can consider only their effects on expected labor supply within a labor supply regime.
References
Abdukadir G (1992) Liquidity constraints as a cause of moonlighting. Appl Econ 24(12):1307–1310
Allen DW (1998) The moonlighting decision of unmarried men and momen: family and labor market influences. Atl Econ J 26(2):190–205
Altonji J, Paxson C (1988) Labor supply preferences, hours constraints, and hours-wage trade-offs. J Labor Econ 6(2):254–276
Averett S (2001) Moonlighting: Multiple motives and gender differences. Appl Econ 33(11):1391–1410
Bell D, Hart R, Wright R (1997) Multiple job holding as a’hedge’against unemployment. CEPR Discussion Papers
Böheim R, Taylor MP (2004) And in the Evening She’s a Singer with the Band - Second Jobs, Plight or Pleasure? IZA Discussion Papers 1081, Institute for the Study of Labor (IZA)
Chung J (1994) Utility and production punctions: theory and applications. Blackwell Oxford, Oxford
Conway KS, Kimmel J (1998) Male labor supply estimates and the decision to moonlight. Labour Econ 5(2):135–166
Dustmann C, Rochina-Barrachina M (2007) Selection correction in panel data models: an application to the estimation of females’ wage equations. Econ J 10(2):263–293
Foley M (1997) Multiple job holding in Russia during economic transition. Economic Growth Center Working Papers No 781
Frederiksen A, Graversen E, Smith N (2008) Overtime work, dual job holding, and taxation. Res Labor Econ 28:25–55
Kimmel J, Conway KS (2001) Who moonlights and why? Evidence from the SIPP. Ind Relat J Econ Soc 40(1):89–120
Krishnan P (1990) The economics of moonlighting: a double self-selection model. Rev Econ Stat 72(2):361–367
Lee L-F (1983) Generalized econometric models with selectivity. Econometrica 51(2):507–12
Lundborg P (1995) Job amenity and the incidence of double work. J Econ Behav Organ 26(2):273–287
Office for National Statistics (2002) Labour market trends. Tech Rep 110(10):S18
Panos GA, Pouliakas K, Zangelidis A (2011) Multiple job holding as a strategy for skills diversification and labour market mobility. Essex Business School, University of Essex, Essex
Paxson C, Sicherman N (1998) The dynamics of dual-job holding and job mobility. J Labor Econ 14(3):357–393
Perlman R (1966) Observations on overtime and moonlighting. South Econ J 33(2):237–244
Renna F, Oaxaca RL, Choe C (2013) Constrained vs Unconstrained Labor Supply: The Economics of Dual Job Holding. LISER Working Paper Series 2013-03, LISER
Shishko R, Rostker B (1976) The economics of multiple job holding. Amer Econ Rev 66(3):298–308
Theisen T (2009) Multiple-job-holding in tanzania. In: Kanbur R, Svejnar J (eds) Labor Markets and Economic Development. Routledge, pp 176–196
Wooldridge J (1995) Selection corrections for panel data models under conditional mean independence assumptions. J Econ 68(1):115–132
Wooldridge J (2010) Econometric analysis of cross section and panel data. The MIT Press, Cambridge
Wu Z, Baimbridge M, Zhu Y (2009) Multiple job holding in the united kingdom: Evidence from the british household panel survey. Appl Econ 41(21):2751–2766
Acknowledgments
We thank three anonymous referees for comments that greatly improved the paper. We also gratefully acknowledge the helpful comments of Changhui Kang, Tiemen Woutersen and participants at the 14th IZA/SOLE Transatlantic Meeting of Labor Economists, as well as seminar participants at CEPS/INSTEAD, University of Bari, University of Lecce, and University of Rome. This work was supported by the Ministry of Education of the Republic of Korea and the National Research Foundation of Korea (NRF-2015S1A5A8014290).
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible editor: Klaus F. Zimmermann
A Technical Appendix
A Technical Appendix
1.1 A.1 Theoretical Stone-Geary elasticities
Unconstrained dual job holder
The own wage Slutsky equation in elasticity form may be expressed by
where
is the uncompensated own wage elasticity for job m,
is the compensated own substitution effect elasticity for job m, S m m is the compensated own substitution effect, and
is the own wage income effect elasticity.
The pure income effect elasticity for job m is given by
The Slutsky equation for cross wage effects in elasticity form is given by
where
is the uncompensated cross wage effect elasticity of labor supply to job m from a change in the wage for job k,
is the compensated cross substitution effect elasticity, S m k is the compensated cross substitution effect of a change in the wage on job k on labor supply to job m, and
is cross-wage income effect elasticity. Observe that both uncompensated and compensated increases in the wage for job k lead to reductions in labor supply to job m.
Unconstrained unitary job holders
The own wage Slutsky equation in elasticity form for job 1 when not working a second job is expressed as
where
is the uncompensated own wage effect elasticity for job 1,
is the compensated own substitution effect elasticity for job 1,\(S_{11}\vert _{h_{2}= 0}\). is the own compensated substitution effect, and
is the income effect elasticity from the own wage.
The pure income effect elasticity for h1∗is determined by
Constrained dual job holder
The own wage Slutsky equation in elasticity form for job 2 when constrained on job 1 may be expressed as
where
is the uncompensated wage uncompensated own wage elasticity for job 2,
is the compensated own substitution elasticity, \(S_{22}{\vert }_{h_{1}={\ddot {h}}_{1}}\) is the compensated own substitution effect, and
is the income effect elasticity from the own wage.
The pure income effect elasticity for h2∗is determined by
Note that the compensated cross-substitution effect of wages on job 1 on labor supply to job 2 is necessarily zero when hours are constrained in job 1 because wages on job 1 can only have income effects. Hence, the uncompensated cross-wage elasticity of w 1 on h2∗ is the same as the cross-wage income effect elasticity:
Constrained unitary job holder
For a constrained unitary job holder, the hours worked \((\ddot {h}_{1})\) are treated as exogenous so there is no corresponding labor supply equation.
1.2 A.2 Empirical elasticities based on the effects of key economic variables on the effects of selection and unobserved heterogeneity.
Unconstrained dual job holder
We express the total labor supply effect of w 1i t as
where \(\left (\frac {\partial \hat {h}_{1it}}{\partial w_{1it}}\right )^{SG}\) is the slope of the uncompensated labor supply curve obtained from the Stone-Geary utility function and \(\frac {\partial \hat {\lambda }_{1it}}{\partial w_{1it}}\) \(=\left [ \dfrac {-\hat {\lambda }_{1it}\left (z_{1it}+\hat {\lambda }_{1it}\right )} {\phi \left (z_{1it}\right )} \right ] \left [ \frac {\partial \hat {P}_{1it}}{\partial w_{1it}}\right ] ,\) \(z_{1it}={\Phi }^{-1}\left (\hat {P}_{1it}\right )\), and \(\hat {P}_{1it}={\Lambda }\left (x_{it},\bar {\omega }_{i},\tilde {\beta }_{1}\right ) \). Accordingly, the total labor supply elasticity with respect to w 1i t is obtained from
where \(\hat {\eta }_{_{11it}}=\hat {\eta }{{~}_{_{11it}}^{c}}+\hat {\epsilon }_{11\text {I}}\) is the estimated Stone-Geary uncompensated labor supply elasticity with respect to w 1i t , \(\hat {\eta }{_{_{11it}}^{c}}\) is the compensated labor supply elasticity with respect to w 1i t (evaluated at \(\hat {h}_{1it}\)) , and \(\hat {\epsilon }_{11\text {I} }\) is the own wage income effect elasticity. The term \(\hat {\theta }_{11}\left [ \frac {1}{\hat {h}_{1it}}\frac {\partial \hat {\lambda }_{1it}}{\partial w_{1it}}-\frac {\hat {\lambda }_{1it}}{w_{1it} \hat {h}_{1it}}\right ] \) captures the job 1 labor supply elasticity effects of w 1i t on the probability of being an unconstrained dual job holder. Although w 1i t does not directly affect the controls for unobserved heterogeneity, it does impact the effect of unobserved heterogeneity on job 1 labor supply via the term \(-\frac {\bar {Z}_{1i}\hat {\pi }_{11}}{w_{1it}\hat {h}_{1it}}\).
The total cross labor supply effect of w 2i t is identical to the uncompensated cross wage effect of w 2i t on labor supply to job 1 from the Stone-Geary utility function:
Therefore, the total labor supply elasticity for job 1 with respect to w 2i t is the same as the estimated Stone-Geary uncompensated cross wage elasticity:
where \(\hat {\eta }_{_{12it}}=\hat {\eta }{~}_{_{12it}}^{c}+\hat {\epsilon }_{12\text {I} }\), \(\hat {\eta }_{_{12it}}^{c}\) is the compensated cross substitution effect elasticity, and \(\hat {\epsilon }_{12\text {I} }\) is the cross wage income effect elasticity.
The total pure income effect of non-labor income on labor supply to job 1 is obtained as
where \(\left (\frac {\partial \hat {h}_{1it}}{\partial I_{it}}\right )^{SG}\) is the Stone-Geary pure income effect for labor supply to job 1 and \(\frac {\partial \hat {\lambda }_{1it}}{\partial I_{it}}=\left [ \frac {-\hat {\lambda }_{1it}\left (z_{1it}+\hat {\lambda }_{1it}\right )} {\phi \left (z_{1it}\right )} \right ] \left [ \frac {\partial \hat {P}_{1it}}{\partial I_{it}}\right ] \). Accordingly, the total labor supply pure income effect elasticity is obtained from
where \(\hat {\eta }_{_{1Iit}}\) is the Stone-Geary pure income effect elasticity for job 1. The term \(\left (\frac {\hat {\theta }_{11}I_{it}}{w_{1it}\hat {h}_{1it}}\right ) \left (\frac {\partial \hat {\lambda }_{1it}}{\partial I_{it}}\right ) \) captures the job 1 labor supply elasticity effects of I i t on the probability of being an unconstrained dual job holder.
The implied hours equation for job 2 obtained from the estimated earnings (12) is given by
Similarly as in job 1, we express the total labor supply effect of w 2i t on job 2 as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial w_{2it}}\right )^{SG}\) is the slope of the uncompensated labor supply curve for job 2 obtained from the Stone-Geary utility function. Accordingly, the total job 2 labor supply elasticity with respect to w 2i t is obtained from
where \(\hat {\eta }_{_{22it}}=\hat {\eta }{~}_{_{22it}}^{c}+\hat {\epsilon }_{22\text {I} }\) is the estimated Stone-Geary uncompensated job 2 labor supply elasticity with respect to w 2i t , \(\hat {\eta }{_{_{22it}}^{c}}\) is the compensated labor supply elasticity with respect to w 2i t (evaluated at \(\hat {h}_{2it}\)) , and \(\hat {\epsilon }_{22\text {I} }\) is the own wage income effect elasticity. Although w 2i t does not affect the probability of a worker being an unconstrained dual job holder, it does affect the impact of selection on job 2 labor supply via the term \(-\frac {\hat {\theta }_{21}\hat {\lambda }_{1it}}{w_{2it}\hat {h}_{2it}}\). Similarly, w 2i t impacts the effect of unobserved heterogeneity on job 2 labor supply via the term \(\frac {\bar {Z}_{1i}\hat {\pi }_{21}}{w_{2it}\hat {h}_{2it}}\).
The total cross-labor supply effect of w 1i t on job 2 labor supply is obtained as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial w_{1it}}\right )^{SG}\) is the cross-wage effect for the uncompensated job 2 labor supply curve obtained from the Stone-Geary utility function. Therefore, the total cross-labor supply elasticity of w 1i t on job 2 labor supply is obtained as
where \(\hat {\eta }_{_{21it}}=\hat {\eta }{{~}_{_{21it}}^{c}}+\hat {\epsilon }_{21\text {I} }\), \(\hat {\eta }{_{_{21it}}^{c}}\) is the compensated cross substitution effect elasticity, and \(\hat {\epsilon }_{21\text {I} }\) is the cross wage income effect elasticity. The term \(\left (\frac {\hat {\theta }_{21}w_{1it}}{w_{2it}\hat {h}_{2it}}\right ) \left (\frac {\partial \hat {\lambda }_{1it}}{\partial w_{1it}}\right ) \) captures the job 2 labor supply elasticity effects of w 1i t on the probability of being an unconstrained dual job holder.
The total pure income effect of non-labor income on labor supply to job 2 is obtained as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial I_{it}}\right )^{SG}\) is the Stone-Geary pure income effect for labor supply to job 2. Accordingly, the total labor supply pure income effect elasticity is obtained from
where \(\hat {\eta }_{_{2Iit}}\) is the Stone-Geary pure income effect elasticity for job 2. The term \(\left (\frac {\hat {\theta }_{21}I_{it}}{w_{2it}\hat {h}_{2it}}\right ) \left (\frac {\partial \hat {\lambda }_{1it}}{\partial I_{it}}\right ) \) captures the job 2 labor supply elasticity effects of I i t on the probability of being an unconstrained dual job holder.
Unconstrained unitary job holders
We express the total labor supply effect of w 1i t as
where \(\left (\frac {\partial \hat {h}_{1it}}{\partial w_{1it}}\vert _{h_{2}= 0}\right )^{SG}\) is the slope of the uncompensated labor supply curve obtained from the Stone-Geary utility function and \(\frac {\partial \hat {\lambda }_{2it}} {\partial w_{1it}}=\left [ \frac {-\hat {\lambda }_{2it}\left (z_{2it}+\hat {\lambda }_{2it}\right )} {\phi \left (z_{2it}\right )} \right ] \left [\frac {\partial \hat {P}_{2it}}{\partial w_{1it}}\right ]\), \(z_{2it}={\Phi }^{-1}\left (\hat {P}_{2it}\right )\), and \(\hat {P}_{2it}={\Lambda }\left (x_{it},\bar {\omega }_{i},\tilde {\beta }_{2}\right )\). Accordingly, the total labor supply elasticity with respect to w 1i t is obtained from
where \(\hat {\eta }_{_{11it}}\vert _{h_{2}= 0}=\hat {\eta }{{~}_{_{11it}}^{c}}\vert _{h_{2}= 0} +\hat {\epsilon }_{11\text {I}} \vert _{h_{2}= 0}\) is the estimated Stone-Geary uncompensated labor supply elasticity with respect to w 1i t , \(\hat {\eta }{{~}_{_{11it}}^{c}}\vert _{h_{2}= 0} \) is the compensated labor supply elasticity with respect to w 1i t (evaluated at \(\hat {h}_{1it}\)) , \(\hat {\epsilon }_{11\text {I} }\vert _{h_{2}= 0}\) is the own wage income effect elasticity. The term \(\hat {\theta }_{12}\left [ \frac {1}{\hat {h}_{1it}}\frac {\partial \hat {\lambda }_{2it}}{\partial w_{1it}}-\frac {\hat {\lambda }_{2it}}{w_{1it}\hat {h}_{1it}}\right ] \) captures the job 1 labor supply elasticity effects of w 1i t on the probability of being an unconstrained dual job holder. w 1i t impacts the effect of unobserved heterogeneity on job 1 labor supply via the term \(-\frac {\bar {Z}_{2i}\hat {\pi }_{12}}{w_{1it}\hat {h}_{1it}}\).
The total pure income effect of non-labor income on labor supply to job 1 is obtained as
where \(\left (\frac {\partial \hat {h}_{1it}}{\partial I_{it}}\vert _{h_{2}= 0} \right )^{SG}\) is the Stone-Geary pure income effect for labor supply and \(\frac {\partial \hat {\lambda }_{2it}} {\partial I_{it}}=\left [ \frac {-\hat {\lambda }_{2it}\left (z_{2it}+\hat {\lambda }_{2it}\right )} {\phi \left (z_{2it}\right )} \right ] \left [ \frac {\partial \hat {P}_{2it}}{\partial I_{it}}\right ] \). Accordingly, the total labor supply pure income effect elasticity is obtained from
where \(\hat{\eta}_{_{1Iit}}\left\vert _{h_{2}=0}\right. \) is the Stone-Geary pure income effect elasticity. The term \(\left (\frac {\hat {\theta }_{12}I_{it}}{w_{1it}\hat {h}_{1it}}\right ) \left (\frac {\partial \hat {\lambda }_{2it}}{\partial I_{it}}\right ) \) captures the labor supply elasticity effects of I i t on the probability of being an unconstrained unitary job holder.
Constrained dual job holders
For over-employed dual job holders:
We express the total labor supply effect of w 2i t on job 2 as
where \(\left( \dfrac{\partial \hat{h}_{2it}}{\partial w_{2it}}\left\vert _{h_{1}=\ddot{h}_{1}>h_{1}^{\ast} }\right. \right) ^{SG}\) is the slope of the uncompensated labor supply curve for job 2 obtained from the Stone-Geary utility function and \(\frac {\partial \hat {\lambda }_{23it}} {\partial w_{1it}}=\left [\frac {-\hat {\lambda }_{23it}\left (z_{3it}+\hat {\lambda }_{3it}\right )}{\phi \left (z_{3it}\right )} \right ] \left [ \frac {\partial \hat {P}_{3it}}{\partial w_{1it}}\right ] ,z_{3it}={\Phi }^{-1}\left (\hat {P}_{3it}\right )\), and \(\hat {P}_{3it}={\Lambda }\left (x_{it},\bar {\omega }_{i},\tilde {\beta }_{3}\right ) \). Accordingly, the total job 2 labor supply elasticity with respect to w 2i t is obtained from
where \(\hat {\eta }_{_{22it}}\left |{~}_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast }}\right . =\hat {\eta }_{_{22it}}^{c}\left |{~}_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } }\right . +\hat {\epsilon }_{22\text {I} }\left |{~}_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } }\right . \) is the estimated Stone-Geary uncompensated job 2 labor supply elasticity with respect to w 2i t , \(\hat {\eta }{{~}_{_{22it}}^{c}}_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast }}\) is the compensated labor supply elasticity with respect to w 2i t (evaluated at \(\hat {h}_{2it}\left |{~}_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast }}\right . \)), and \(\hat {\epsilon }_{22\mathrm {I} }\left |_{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } }\right . \) is the own wage income effect elasticity. The effects of selection and unobserved heterogeneity on job 2 labor supply are affected by changes in w 2i t because the selection and unobserved heterogeneity terms in the job 2 labor supply function include w 2i t in the denominator. After differentiating job 2 labor supply with respect to w 2i t and converting to elasticities, the selection and unobserved heterogeneity effects are captured by \(-\frac {\hat {\theta }_{23}\hat {\lambda }_{23it}}{w_{2it}\hat {h}_{2it}}\ \)and \(-\frac {\bar {Z}_{3i}\hat {\pi }_{23}}{w_{2it}\hat {h}_{2it}}\), respectively.
Because hours are constrained for job 1, the wage rate for job 1 can only have income and selection effects on labor supply to job 2 but not substitution effects. The total cross-labor supply effect of w 1i t on job 2 labor supply is obtained as
where \(\left( \dfrac{\partial \hat{h}_{2it}}{\partial w_{1it}}\left\vert _{h_{1}=\ddot{h}_{1}>h_{1}^{\ast} }\right. \right) ^{SG}\) is the cross-wage effect for the uncompensated job 2 labor supply curve obtained from the Stone-Geary utility function. Therefore, the total cross-labor supply elasticity of w 1i t on job 2 labor supply is obtained as
where \(\hat {\eta }_{_{21it}}\vert _{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } } =\hat {\epsilon }_{21\text {I} }\vert _{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } }\) is the estimated Stone-Geary uncompensated job 2 cross-labor supply elasticity, and \(\left (\frac {\hat {\theta }_{23}\ w_{1it}}{w_{2it}\hat {h}_{2it}}\right ) \left (\frac {\partial \hat {\lambda }_{23it}}{\partial w_{1it}}\right ) \) is the job 2 labor supply elasticity effects of w 1i t on the probability of being a constrained over-employed dual job holder.
The total pure income effect of non-labor income on labor supply to job 2 is obtained as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial I_{it}}\vert _{h_{1}=\ddot {h}_{1}>h_{1}^{\ast } }\right )^{SG}\) is the Stone-Geary pure income effect for labor supply to job 2 and \(\frac {\partial \hat {\lambda }_{23it}} {\partial I_{it}}=\left [ \dfrac {-\hat {\lambda }_{23it}\left (z_{3it}+\hat {\lambda }_{3it}\right )} {\phi \left (z_{3it}\right )} \right ] \left [ \frac {\partial \hat {P}_{3it}} {\partial I_{it}}\right ] \). Accordingly, the total labor supply pure income effect elasticity is obtained from
where \(\hat {\eta }_{_{2Iit}}\vert _{h_{1}=\ddot {h}_{1}>h_{1}^{\ast }}\) is the Stone-Geary pure income effect elasticity for job 2. The term \(\left (\frac {\hat {\theta }_{23}I_{it}}{w_{2it}\hat {h}_{2it}}\right )\left (\frac {\partial \hat {\lambda }_{23it}}{\partial I_{it}}\right ) \) captures the job 2 labor supply elasticity effects of I i t on the probability of being a constrained over-employed dual job holder.
For under-employed dual job holders:
We express the total labor supply effect of w 2i t on job 2 as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial w_{2it}} \vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } } \right )^{SG}\) is the slope of the uncompensated labor supply curve for job 2 obtained from the Stone-Geary utility function and \(\frac {\partial \hat {\lambda }_{24it}} {\partial w_{1it}}=\left [ \dfrac {-\hat {\lambda }_{24it}\left (z_{4it}+\hat {\lambda }_{4it}\right )} {\phi \left (z_{4it}\right )} \right ] \left [ \frac {\partial \hat {P}_{4it}} {\partial w_{1it}}\right ] ,z_{4it}={\Phi }^{-1}\left (\hat {P}_{4it}\right )\), and \(\hat {P}_{4it}={\Lambda }\left (x_{it},\bar {\omega }_{i},\tilde {\beta }_{4}\right ) \). Accordingly, the total job 2 labor supply elasticity with respect to w 2i t is obtained from
where \(\hat {\eta }_{_{22it}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } } =\hat {\eta }{_{_{22it}}^{c}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } } +\hat {\epsilon }_{22\text {I} }\vert _{h_{1}= \ddot {h}_{1}<h_{1}^{\ast } } \) is the estimated Stone-Geary uncompensated job 2 labor supply elasticity with respect to w 2i t , \(\hat {\eta }{_{_{22it}}^{c}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } } \) is the compensated labor supply elasticity with respect to w 2i t (evaluated at \(\hat {h}_{2it}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast }} \)), and \(\hat {\epsilon }_{22\text {I} }\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } }\) is the own wage income effect elasticity. The effects of selection and unobserved heterogeneity on job 2 labor supply are affected by changes in w 2i t because the selection and unobserved heterogeneity terms in the job 2 labor supply function include w 2i t in the denominator. After differentiating job 2 labor supply with respect to w 2i t and converting to elasticities, the selection and unobserved heterogeneity effects are captured by \(-\frac {\hat {\theta }_{24}\hat {\lambda }_{24it}}{w_{2it}\hat {h}_{2it}}\) and \(-\frac {\bar {Z}_{4i}\hat {\pi }_{24}}{w_{2it}\hat {h}_{2it}}\), respectively.
Because hours are constrained for job 1, the wage rate for job 1 can only have income and selection effects on labor supply to job 2 but not substitution effects. The total cross-labor supply effect of w 1i t on job 2 labor supply is obtained as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial w_{1it}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } }\right )^{SG}\) is the cross-wage effect for the uncompensated job 2 labor supply curve obtained from the Stone-Geary utility function. Therefore, the total cross-labor supply elasticity of w 1i t on job 2 labor supply is obtained as
where \(\hat {\eta }_{_{21it}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast }} =\hat {\epsilon }_{21\text {I} }\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } }\) is the estimated Stone-Geary uncompensated job 2 cross-labor supply elasticity, and \(\left (\frac {\hat {\theta }_{24}\ w_{1it}}{w_{2it}\hat {h}_{2it}}\right ) \left (\frac {\partial \hat {\lambda }_{24it}}{\partial w_{1it}}\right ) \) is the job 2 labor supply elasticity effects of w 1i t on the probability of being a constrained under-employed dual job holder.
The total pure income effect of non-labor income on labor supply to job 2 is obtained as
where \(\left (\frac {\partial \hat {h}_{2it}}{\partial I_{it}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } }\right )^{SG}\) is the Stone-Geary pure income effect for labor supply to job 2and \(\frac {\partial \hat {\lambda }_{24it}} {\partial I_{it}}=\left [ \dfrac {-\hat {\lambda }_{24it}\left (z_{4it}+\hat {\lambda }_{4it}\right )} {\phi \left (z_{4it}\right )} \right ] \left [ \frac {\partial \hat {P}_{4it}} {\partial I_{it}}\right ] \). Accordingly, the total labor supply pure income effect elasticity is obtained from
where \(\hat {\eta }_{_{2Iit}}\vert _{h_{1}=\ddot {h}_{1}<h_{1}^{\ast } }\) is the Stone-Geary pure income effect elasticity for job 2. The term \(\left (\frac {\hat {\theta }_{24}I_{it}}{w_{2it}\hat {h}_{2it}}\right ) \left (\frac {\partial \hat {\lambda }_{24it}}{\partial I_{it}}\right ) \) captures the job 2 labor supply elasticity effects of I i t on the probability of being a constrained under-employed dual job holder.
Rights and permissions
About this article
Cite this article
Choe, C., Oaxaca, R.L. & Renna, F. Constrained vs unconstrained labor supply: the economics of dual job holding. J Popul Econ 31, 1279–1319 (2018). https://doi.org/10.1007/s00148-017-0678-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00148-017-0678-4