Skip to main content
SpringerLink
Log in

A Novel Quantum Audio Steganography–Steganalysis Approach Using LSFQ-Based Embedding and QKNN-Based Classifier

  • Published:
Circuits, Systems, and Signal Processing Aims and scope Submit manuscript

Abstract

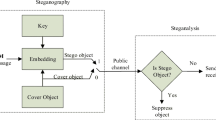
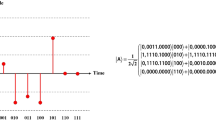
In this paper, a steganography–steganalysis model for quantum audio signals is presented. This model consists of two separate parts of steganography and steganalysis. In the steganography part, to increase the imperceptibility and enhance the undetectability of the least significant bit method, first, the quantum host audio signal is divided into quantum frames of the specified length, and then, using the triangular number sequence, some samples of each frame are selected for the embedding operation. Finally, the embedding process is carried out within the least significant fractional qubit of the amplitude information of the selected samples. The steganalysis part also includes a quantum universal steganalyzer for detecting the embedding operations of the steganography part and a feature extraction module for calculating the power feature of quantum audio signal frames. The recognition operation is based on the quantum K-nearest neighbor algorithm and the Hamming distance criterion. All quantum circuits of the steganography and steganalysis parts of the proposed model were simulated and then tested and evaluated using different audio files. Detection accuracy of over 90% indicates the accuracy and efficiency of the proposed method in quantum audio steganography detection in the context of quantum communication networks.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

(figures and descriptions adapted from [20, 49], respectively)

Fig. 3

(figures and descriptions adapted from [20])

Fig. 4

(figures adapted from [20])

Fig. 5
Fig. 6

(figure adapted from [44])

Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. E. Aïmeur, G. Brassard, S. Gambs, Quantum speed-up for unsupervised learning. Mach. Learn. 90(2), 261–287 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  2. A.H. Ali, L.E. George, A. Zaidan, M.R. Mokhtar, High capacity, transparent and secure audio steganography model based on fractal coding and chaotic map in temporal domain. Multimed. Tools Appl. 77(23), 31487–31516 (2018)

    Article  Google Scholar 

  3. G.E. Andrews, Number Theory (Courier Corporation, North Chelmsford, 1994)

    Google Scholar 

  4. K. Bailey, K. Curran, J. Condell, Evaluation of pixel-based steganography and stegodetection methods. Imaging Sci. J. 52(3), 131–150 (2004)

    Article  Google Scholar 

  5. J. Biamonte, P. Wittek, N. Pancotti, P. Rebentrost, N. Wiebe, S. Lloyd, Quantum machine learning. Nature 549(7671), 195 (2017)

    Article  Google Scholar 

  6. R. Böhme, Advanced Statistical Steganalysis (Springer, Cham, 2010)

    Book  MATH  Google Scholar 

  7. R. Chandramouli, M. Kharrazi, N. Memon, Image steganography and steganalysis: concepts and practice. in International Workshop on Digital Watermarking (Springer, 2003), pp. 35–49

  8. K. Chen, F. Yan, A.M. Iliyasu, J. Zhao, Exploring the implementation of steganography protocols on quantum audio signals. Int. J. Theor. Phys. 57(2), 476–494 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  9. K. Chen, F. Yan, A.M. Iliyasu, J. Zhao, Dual quantum audio watermarking schemes based on quantum discrete cosine transform. Int. J. Theor. Phys. 58(2), 502–521 (2019)

    Article  MATH  Google Scholar 

  10. D. Deutsch, Uncertainty in quantum measurements. Phys. Rev. Lett. 50(9), 631 (1983)

    Article  MathSciNet  Google Scholar 

  11. V. Dunjko, H.J. Briegel, Machine learning & artificial intelligence in the quantum domain: a review of recent progress. Rep. Prog. Phys. 81(7), 074001 (2018)

    Article  MathSciNet  Google Scholar 

  12. V. Dunjko, J.M. Taylor, H.J. Briegel, Quantum-enhanced machine learning. Phys. Rev. Lett. 117(13), 130501 (2016)

    Article  MathSciNet  Google Scholar 

  13. S.E. El-Khamy, N.O. Korany, M.H. El-Sherif, A security enhanced robust audio steganography algorithm for image hiding using sample comparison in discrete wavelet transform domain and RSA encryption. Multimed. Tools Appl. 76(22), 24091–24106 (2017)

    Article  Google Scholar 

  14. A.A.A. EL-Latif, B. Abd-El-Atty, S.E. Venegas-Andraca, A novel image steganography technique based on quantum substitution boxes. Opt. Laser Technol. 116, 92–102 (2019)

    Article  Google Scholar 

  15. S. Heidari, M.R. Pourarian, R. Gheibi, M. Naseri, M. Houshmand, Quantum red–green–blue image steganography. Int. J. Quantum Inf. 15(05), 1750039 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  16. N. Jiang, L. Wang, A quantum image information hiding algorithm based on Moiré pattern. Int. J. Theor. Phys. 54, 1021–1032 (2014)

    Article  Google Scholar 

  17. N. Jiang, N. Zhao, L. Wang, LSB based quantum image steganography algorithm. Int. J. Theor. Phys. 55(1), 107–123 (2016)

    Article  MATH  Google Scholar 

  18. J.I. Latorre, Image compression and entanglement (2005). arXiv:quant-ph/0510031

  19. P.Q. Le, F. Dong, K. Hirota, A flexible representation of quantum images for polynomial preparation, image compression, and processing operations. Quantum Inf. Process. 10(1), 63–84 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  20. P. Li, B. Wang, H. Xiao, X. Liu, Quantum representation and basic operations of digital signals. Int. J. Theor. Phys. 57(10), 3242–3270 (2018)

    Article  MATH  Google Scholar 

  21. S. Lloyd, M. Mohseni, P. Rebentrost, Quantum algorithms for supervised and unsupervised machine learning (2013). arXiv:1307.0411

  22. R.G. Lyons, Understanding Digital Signal Processing, 3rd edn. (Pearson Education India, Delhi, 2011)

    Google Scholar 

  23. M. Naseri, S. Heidari, M. Baghfalaki, R. Gheibi, J. Batle, A. Farouk, A. Habibi, A new secure quantum watermarking scheme. Optik 139, 77–86 (2017)

    Article  Google Scholar 

  24. M.Y. Nejad, M. Mosleh, S.R. Heikalabad, An LSB-based quantum audio watermarking using MSB as arbiter. Int. J. Theor. Phys. 58, 3828–3851 (2019)

    Article  Google Scholar 

  25. A.V. Oppenheim, Discrete-Time Signal Processing (Pearson Education India, Delhi, 1999)

    Google Scholar 

  26. Z.-G. Qu, H.-X. He, T. Li, Novel quantum watermarking algorithm based on improved least significant qubit modification for quantum audio. Chin. Phys. B 27(1), 010306 (2018)

    Article  Google Scholar 

  27. Z. Qu, Z. Cheng, W. Liu, X. Wang, A novel quantum image steganography algorithm based on exploiting modification direction. Multimed. Tools Appl. 78, 7981–8001 (2018)

    Article  Google Scholar 

  28. Z. Qu, Z. Cheng, W. Liu, X. Wang, A novel quantum image steganography algorithm based on exploiting modification direction. Multimed. Tools Appl. 78(7), 7981–8001 (2019)

    Article  Google Scholar 

  29. P. Rebentrost, M. Mohseni, S. Lloyd, Quantum support vector machine for big data classification. Phys. Rev. Lett. 113(13), 130503 (2014)

    Article  Google Scholar 

  30. Y. Ruan, H. Chen, J. Tan, X. Li, Quantum computation for large-scale image classification. Quantum Inf. Process. 15(10), 4049–4069 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  31. Y. Ruan, X. Xue, H. Liu, J. Tan, X. Li, Quantum algorithm for k-nearest neighbors classification based on the metric of hamming distance. Int. J. Theor. Phys. 56(11), 3496–3507 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  32. E. Şahin, İ. Yilmaz, A novel quantum steganography algorithm based on LSBq for multi-wavelength quantum images. Quantum Inf. Process. 17(11), 319 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  33. E. Şahin, İ. Yilmaz, QRMA: quantum representation of multichannel audio. Quantum Inf. Process. 18(7), 209 (2019)

    Article  Google Scholar 

  34. J. Sang, S. Wang, Q. Li, A novel quantum representation of color digital images. Quantum Inf. Process. 16(2), 42 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  35. M. Schuld, I. Sinayskiy, F. Petruccione, Quantum computing for pattern classification. in Pacific Rim International Conference on Artificial Intelligence 2014 (Springer, 2014), pp. 208–220

  36. G.J. Simmons, The prisoners’ problem and the subliminal channel, in Advances in Cryptology, ed. by D. Chaum (Springer, Boston, 1984), pp. 51–67

    Chapter  Google Scholar 

  37. B. Sun, A.M. Iliyasu, F. Yan, F. Dong, K. Hirota, An RGB multi-channel representation for images on quantum computers. J. Adv. Comput. Intell. Intell. Inf. 17(3), 404–417 (2013)

    Article  Google Scholar 

  38. A.Z. Tirkel, G. Rankin, R. Van Schyndel, W. Ho, N. Mee, C.F. Osborne, Electronic watermark. in Digital Image Computing, Technology Applications (1993), pp. 666–673

  39. C.A. Trugenberger, Probabilistic quantum memories. Phys. Rev. Lett. 87(6), 067901 (2001)

    Article  Google Scholar 

  40. V. Vedral, A. Barenco, A. Ekert, Quantum networks for elementary arithmetic operations. Phys. Rev. A 54(1), 147 (1996)

    Article  MathSciNet  Google Scholar 

  41. S.E. Venegas-Andraca, S. Bose, Storing, processing, and retrieving an image using quantum mechanics. in Quantum Information and Computation 2003 (International Society for Optics and Photonics, 2003), pp. 137–148

  42. S.E. Venegas-Andraca, J. Ball, Processing images in entangled quantum systems. Quantum Inf. Process. 9(1), 1–11 (2010)

    Article  MathSciNet  Google Scholar 

  43. J. Wang, QRDA: quantum representation of digital audio. Int. J. Theor. Phys. 55(3), 1622–1641 (2016)

    Article  MATH  Google Scholar 

  44. D. Wang, Z.-H. Liu, W.-N. Zhu, S.-Z. Li, Design of quantum comparator based on extended general Toffoli gates with multiple targets. Comput. Sci. 39(9), 302–306 (2012)

    Google Scholar 

  45. S. Wang, J. Sang, X. Song, X. Niu, Least significant qubit (LSQb) information hiding algorithm for quantum image. Measurement 73, 352–359 (2015)

    Article  Google Scholar 

  46. J. Wiśniewska, M. Sawerwain, Recognizing the pattern of binary Hermitian matrices by quantum kNN and SVM methods. Vietnam J. Comput. Sci. 5, 197–204 (2018)

    Article  Google Scholar 

  47. W.K. Wootters, W.H. Zurek, A single quantum cannot be cloned. Nature 299(5886), 802 (1982)

    Article  MATH  Google Scholar 

  48. F. Yan, A.M. Iliyasu, Y. Guo, H. Yang, Flexible representation and manipulation of audio signals on quantum computers. Theor. Comput. Sci. 752, 71–85 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  49. Y. Zhang, K. Lu, Y. Gao, M. Wang, NEQR: a novel enhanced quantum representation of digital images. Quantum Inf. Process. 12(8), 2833–2860 (2013)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Computer Engineering, Dezful Branch, Islamic Azad University, Dezful, Iran

    Javad Chaharlang & Mohammad Mosleh

  2. Department of Computer Engineering, Tabriz Branch, Islamic Azad University, Tabriz, Iran

    Saeed Rasouli Heikalabad

Authors
  1. Javad Chaharlang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohammad Mosleh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Saeed Rasouli Heikalabad
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mohammad Mosleh.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chaharlang, J., Mosleh, M. & Rasouli Heikalabad, S. A Novel Quantum Audio Steganography–Steganalysis Approach Using LSFQ-Based Embedding and QKNN-Based Classifier. Circuits Syst Signal Process 39, 3925–3957 (2020). https://doi.org/10.1007/s00034-020-01345-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00034-020-01345-6

Keywords

Search

Navigation