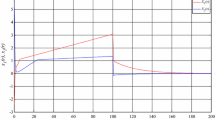
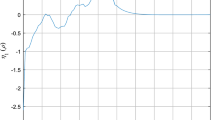
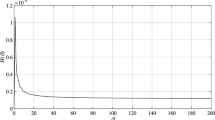
Abstract
This paper investigates the problem of the input-to-state stability (ISS) of fixed-point state-space digital filters in the presence of saturation overflow arithmetic and external interference. By utilizing an augmented Lyapunov function and the passivity property associated with multiple saturation nonlinearities, a new criterion for the ISS of interfered digital filters with saturation is proposed. When the external interference is present, the criterion can be used to determine ISS of digital filters. Without the external interference, the criterion is capable of detecting the asymptotic stability of digital filters. The proposed ISS criterion is in linear matrix inequality framework and, hence, is computationally tractable. The obtained criterion turns out to be less conservative than a recently reported ISS criterion. The superiority of the presented approach over the existing techniques is confirmed by numerical examples.


Similar content being viewed by others
References
C.K. Ahn, Two new criteria for the realization of interfered digital filters utilizing saturation overflow nonlinearity. Signal Process. 95, 171–176 (2014)
C.K. Ahn, P. Shi, Dissipativity analysis for fixed-point interfered digital filters. Signal Process. 109, 148–153 (2015)
H. Gao, C. Wang, New approaches to robust l 2 − l ∞ and H ∞ filtering for uncertain discrete-time systems. Sci. China Ser. F 46(5), 355–370 (2003)
S. Huang, M.R. James, D. Nešić, P.M. Dower, A unified approach to controller design for achieving ISS and related properties. IEEE Trans. Autom. Control 50(11), 1681–1697 (2005)
Z.-P. Jiang, Y. Wang, Input-to-state stability for discrete-time nonlinear systems. Automatica 37(6), 857–869 (2001)
H. Kar, An LMI based criterion for the nonexistence of overflow oscillations in fixed-point state-space digital filters using saturation arithmetic. Digit. Signal Process. 17(3), 685–689 (2007)
H. Kar, A new sufficient condition for the global asymptotic stability of 2-D state-space digital filters with saturation arithmetic. Signal Process. 88(1), 86–98 (2008)
M.K. Kumar, P. Kokil, H. Kar, A new realizability condition for fixed-point state-space interfered digital filters using any combination of overflow and quantization nonlinearities. Circuits Syst. Signal Process. 36(8), 3289–3302 (2017)
D. Liu, A.N. Michel, Asymptotic stability of discrete-time systems with saturation nonlinearities with applications to digital filters. IEEE Trans. Circuits Syst. I 39(10), 798–807 (1992)
Y. Liu, Y. Kao, H.R. Karimi, Z. Gao, Input-to-state stability for discrete-time nonlinear switched singular systems. Inf. Sci. 358–359, 18–28 (2016)
J. Löfberg, YALMIP: a toolbox for modeling and optimization in MATLAB, in Proceedings of the 2004 IEEE International Symposium on CACSD, Taipei, Taiwan, 2004, pp. 284–289
S.K. Mitra, R.J. Sherwood, Digital ladder networks. IEEE Trans. Audio Electroacoust. AU-21(1), 30–36 (1973)
J. Monteiro, R.V. Leuken (eds.), Integrated Circuit and System Design: Power and Timing Modeling, Optimization and Simulation (Springer, Berlin, 2010)
N. Noroozi, A. Khayatian, S. Ahmadizadeh, H.R. Karimi, On integral input-to-state stability for a feedback interconnection of parameterised discrete-time systems. Int. J. Syst. Sci. 47(7), 1598–1614 (2016)
J.G. Proakis, D.G. Manolakis, Digital Signal Processing: Principles, Algorithms, and Applications (Pearson Prentice Hall, New Jersey, 1996)
L.R. Rabiner, B. Gold, Theory and Application of Digital Signal Processing (Prentice-Hall, New Jersey, 1975)
P. Rani, P. Kokil, H. Kar, l 2 − l ∞ suppression of limit cycles in interfered digital filters with generalized overflow nonlinearities. Circuits Syst. Signal Process. 36(7), 2727–2741 (2017)
M. Rehan, M. Tufail, M.T. Akhtar, On elimination of overflow oscillations in linear time-varying 2-D digital filters represented by a Roesser model. Signal Process. 127, 247–252 (2016)
D. Schlichthärle, Digital Filters: Basics and Design (Springer, Berlin, 2000)
T. Shen, Z. Yuan, X. Wang, A new stability criterion for fixed-point state–space digital filters using two’s complement arithmetic. Automatica 47(7), 1538–1541 (2011)
T. Shen, Z. Yuan, X. Wang, An improved stability criterion for fixed-point state-space digital filters using two’s complement arithmetic. Automatica 48(1), 243–245 (2012)
H. Shen, J. Wang, J.H. Park, Z.-G. Wu, Condition of the elimination of overflow oscillations in two-dimensional digital filters with external interference. IET Signal Process. 8(8), 885–890 (2014)
V. Singh, Elimination of overflow oscillations in fixed-point state-space digital filters using saturation arithmetic. IEEE Trans. Circuits Syst. 37(6), 814–818 (1990)
E.D. Sontag, Smooth stabilization implies coprime factorization. IEEE Trans. Autom. Control 34(4), 435–443 (1989)
J.F. Sturm, Using SeDuMi 1.02, a MATLAB toolbox for optimization over symmetric cones. Optim. Methods Softw. 11(1–4), 625–653 (1999)
A.R. Teel, J.P. Hespanha, A. Subbaraman, Equivalent characterizations of input-to-state stability for stochastic discrete-time systems. IEEE Trans. Autom. Control 59(2), 516–522 (2014)
Y. Tsividis, Mixed Analog–Digital VLSI Devices and Technology (World Scientific Publishing, Singapore, 2002)
D. Zhang, S.K. Nguang, D. Srinivasan, L. Yu, Distributed filtering for discrete-time T–S fuzzy systems with incomplete measurements. IEEE Trans. Fuzzy Syst. (2017). https://doi.org/10.1109/TFUZZ.2017.2725228
D. Zhang, Z. Xu, H.R. Karimi, Q.-G. Wang, Distributed filtering for switched linear systems with sensor networks in presence of packet dropouts and quantization. IEEE Trans. Circuits Syst. I 64(10), 2783–2796 (2017)
D. Zhang, Q.-G. Wang, D. Srinivasan, H. Li, L. Yu, Asynchronous state estimation for discrete-time switched complex networks with communication constraints. IEEE Trans. Neural Netw. Learn. Syst. 29(5), 1732–1746 (2018)
Acknowledgements
The authors thank the Editors and the anonymous reviewers for their constructive comments and suggestions to improve the manuscript.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kumar, M.K., Kar, H. ISS Criterion for the Realization of Fixed-Point State-Space Digital Filters with Saturation Arithmetic and External Interference. Circuits Syst Signal Process 37, 5664–5679 (2018). https://doi.org/10.1007/s00034-018-0836-2
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00034-018-0836-2