Abstract.
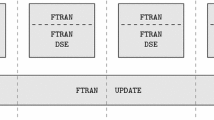
We describe a coarse-grain parallel approach for the homogeneous solution of linear systems. Our solutions are symbolic, i.e., exact rather than numerical approximations. We have performed an outer loop parallelization that works well in conjunction with a black box abstraction for the coefficient matrix. Our implementation can be run on a network cluster of UNIX workstations as well as on an SP-2 multiprocessor. Task distribution and management are effected through MPI and other packages. Fault tolerance, checkpointing, and recovery are incorporated. Detailed timings are presented for experiments with systems that arise in RSA challenge integer factoring efforts. For example, we can solve a 252,222 × 252,222 system with about 11.04 million nonzero entries over the Galois field with two elements using four processors of an SP-2 multiprocessor, in about 26.5 hours CPU time.
Similar content being viewed by others
Author information
Authors and Affiliations
Additional information
Received June 1, 1997; revised March 10, 1998.
Rights and permissions
About this article
Cite this article
Kaltofen, E., Lobo, A. Distributed Matrix-Free Solution of Large Sparse Linear Systems over Finite Fields . Algorithmica 24, 331–348 (1999). https://doi.org/10.1007/PL00008266
Issue Date:
DOI: https://doi.org/10.1007/PL00008266