Skip to main content
SpringerLink
Log in

Decoupled estimation of angular parameters for coherently distributed sources

Estimation découplée des paramètres angulaires pour des sources distribuées avec cohérence

  • Published:
Annales Des Télécommunications Aims and scope Submit manuscript

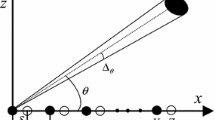
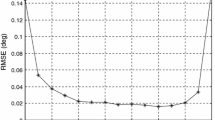
Abstract

In this paper, we consider the problem of estimating the direction-of-arrivals (doas) and angular spreads of uncorrelated coherently distributed sources. The proposed methods enable a decoupled estimation of the doas from that of the angular spreads of sources. Compared with existing algorithms, the proposed algorithms improve the robustness to the mismodeling of the spatial distribution of the scatterers. They allow an unambiguous doas estimation and don’t need the a priori knowledge of the azimutal power distribution. Furthermore, the proposed techniques work even in the case where the different sources have different angular distribution shape.

Résumé

Dans cet article on considère le problème d’estimation des directions d’arrivée (dda) et des étalements angulaires de sources décorrélées distribuées avec cohérence. Les techniques proposées permettent d’estimer ces paramètres angulaires de façon séparée. Par comparaison avec des techniques existantes, ces nouvelles méthodes n’exigent pas la connaissance des distributions angulaires des réflecteurs, en particulier pour l’estimation des dda. Notons que ces méthodes sont capables de fonctionner lorsque les différentes sources ont des distributions azimutales différentes, contrairement aux méthodes existantes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Zetterberg P., “Mobile cellular communications with base station antenna arrays: Spectrum, algorithms and propagation models”, PhD thesis, Royal Institute of technology, Sweden, 1997.

    Google Scholar 

  2. Pedersen K.I., Mogensen P.E., Fleury B. H., “A stochastic model of the temporal and azimuthal dispersion seen at the base station in outdoor propagation environments”, IEEE Transaction Veh. Technology, vol. 49, pp. 437–447, Mar. 2000.

    Google Scholar 

  3. Valaee S., Champagne B., Kabal P., “Parametric localization of distributed sources”, IEEE Trans. on Signal Processing, vol. 43, pp. 2144–2153, Sept. 1995.

    Google Scholar 

  4. Bengtsson M., “Antenna array signal processing for high rank models”, PhD thesis, Royal Institue of technology, Sweden, 1999.

    Google Scholar 

  5. Hassanien A., Shahbazpanahi S., Gershman A.B., “A generalized Capon estimator for localization of multiple spread sources”, IEEE Transaction on signal processing, vol. 52(1), pp. 280–283, January 2004.

    MathSciNet  Google Scholar 

  6. Capon J., “High resolution frequency-wavenumber spectrum analysis”, Proc. IEEE, volume 57, pp. 1408–1418, August 1969.

    Google Scholar 

  7. Besson O., Stoica P., “Decoupled estimation of doa and angular spread for a spatially distributed source”, IEEE Trans. on signal processing, vol. 48(7), pp. 1872–1882, July 2000.

    MATH  Google Scholar 

  8. Zoubir A., Wang Y., Chargé P., “On the ambiguity of comet-exip algorithm for estimating a scattered source”, Proc. of ICASSP, March 2005.

    Google Scholar 

  9. Lee J., Song I., Kwon H., Lee S., “Low-complexity estimation of 2D doa for coherently distributed sources”, Elsevier Signal Processing, 83, pp. 1789–1802, 2003.

    MATH  Google Scholar 

  10. Wan Q., Liu S. J., Ge F.X., Yuan J., Peng Y. N., “doa estimator for multiple coherently distributed sources with symmetric angular distribution”, In Proc. of Vehicular Technology Conference VTC 2003, vol. 1, pp. 213–216, April 2003.

    Google Scholar 

  11. Zoubir A., Wang Y, Chargé P., “The generalized beamforming techniques for estimating the coherently distributed sources”, In Proc. of 8th European Conference on Wireless Technology, pp. 157–160, Paris, October 2005.

    Google Scholar 

  12. Ghogho M., Besson O., Swami A., “Estimation of directions of arrival of multiple scattered sources”, IEEE Transaction on Signal Processing, vol. 49, pp. 2467–2479, November 2001.

    Google Scholar 

  13. Fletcher R., Powell M.J.D., “A Rapidly Convergent Descent Method for Minimization”, Computer Journal, vol. 6, pp. 163–168, 1963.

    MathSciNet  MATH  Google Scholar 

  14. Goldfarb D., “A Family of Variable Metric Updates Derived by Variational Means”, Mathematics of Computing, vol. 24, pp. 23–26, 1970.

    MathSciNet  MATH  Google Scholar 

  15. Han S.P., “A Globally Convergent Method for Nonlinear Programming”, Journal of Optimization Theory and Applications, vol. 22, pp. 297, 1977.

    MATH  Google Scholar 

  16. Powell M.J.D., “A Fast Algorithm for Nonlinearly Constrained Optimization Calculations”, Numerical Analysis, ed. G.A. Watson, Lecture Notes in Mathematics, Springer Verlag, vol. 630, 1978.

    Google Scholar 

  17. Powell M. J.D., “The Convergence of Variable Metric Methods For Nonlinearly Constrained Optimization Calculations”, Nonlinear Programming 3 (O.L. Mangasarian, R.R. Meyer, and S.M. Robinson, eds.), Academic Press, 1978.

    Google Scholar 

  18. Gill P.E., Murray W., Wright M.H., “Practical Optimization”, London, Academic Press, 1981.

Download references

Author information

Authors and Affiliations

  1. IREENA École polytechnique, University of Nantes, BP 50609, 44306, Nantes, France

    Ahmed Zoubir & Mohamed M. Ould Haiballa

  2. SSC, FST, University of Sidi Mohamed Ben Abdellah, Route Immouzer, BP 2202, Fez, Morocco

    Yide Wang & Mohamed M. Ould Haiballa

  3. LATTIS, INSA, 135 avenue de Rangueil, 31077, Toulouse Cedex 4, France

    Pascal Chargé

Authors
  1. Ahmed Zoubir
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yide Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mohamed M. Ould Haiballa
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Pascal Chargé
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ahmed Zoubir.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zoubir, A., Wang, Y., Ould Haiballa, M.M. et al. Decoupled estimation of angular parameters for coherently distributed sources. Ann. Telecommun. 62, 1171–1184 (2007). https://doi.org/10.1007/BF03253311

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF03253311

Key words

Mots clés

Search

Navigation