Summary
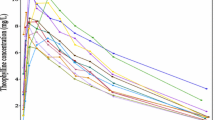
The gamma variate, C=Ata exp(−bt), was tested, as a fitting function, with various real and error-free simulated intravascular and extravascular pharmacokinetic data sets and the results compared with polyexponential fits. For extravascular data, the gamma variate is only suitable to globally fit data which might otherwise be described biexponentially. For intravascular data, the gamma variate could only fit a limited range of the possible concentration-time profiles. Gamma variate fitting algorithms must minimize relative deviations; fits using unweighted sums of squared deviations gave excellent results at higher concentration values but consistently underestimated terminal descending portions of the data.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Norris W.P., Kisielski W. (1948): Comparative metabolism of radium, strontium and calcium. Cold Spring Harbor Symp. Quant Biol., 13, 164–172.
Way K., Wigner E.P. (1948): Rate of decay of fission products. Physical Rev., 73, 1318–1330.
Weiss M. (1983): Use of gamma distributed residence times in pharmacokinetics. Eur. J. Clin. Pharmacol., 25, 695–702.
Sainsbury E.J., Ashley J.J. (1986): Curve-fitting in pharmacokinetics — a comparison between gamma and biexponential fits. Eur. J. Clin. Pharmacol., 30, 243–244.
Norris W.P., Tyler S.A., Brues A.M. (1958): Retention of radioactive bone-seekers. Science, 128, 456–462.
Hartley H.O. (1961): The modified Gauss-Newton method for the fitting of non-linear regression functions by least squares. Technometrics, 3, 269–280.
Brown R.D., Manno J.E. (1978): ESTRIP, a BASIC computer program for obtaining initial polyexponential parameter estimates. J. Pharm. Sci., 67, 1687–1691.
Worsley B.H., Lax L.C. (1962): Selection of a numerical technique for analyzing experimental data of the decay type with special reference to the use of tracers in biological systems. Biochim. Biophys. Acta., 59, 1–24.
Ottaway J.H. (1973): Normalization in the fitting of data by iterative methods. Biochem. J., 134, 729–736.
Berman M., Weiss M.F. (1967): Users Manual for SAAM, U.S. Public Health Service 1703, U.S. Government Printing Office, Washington.
Gibaldi M., Perrier D. (1982): Pharmacokinetics, 2nd ed., New York: Marcel Detter, pp. 433–444.
Wagner J.G. (1975): Fundamentals of clinical pharmacokinetics. Drug Intelligence Publications, Hamilton, Illinois, pp. 60–62.
Kahlmeter G., Jonsson S., Kamme C. (1978): Multiple-compartment pharmacokinetics of tobramycin. J. Antimicrob. Chemother., 4(suppl A), 5–11.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Pfeffer, M. Gamma variate fits to pharmacokinetic data. European Journal of Drug Metabolism and Pharmacokinetics 13, 155–159 (1988). https://doi.org/10.1007/BF03189934
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF03189934