Skip to main content
SpringerLink
Log in

Governing equations of envelope surface created by nearly bichromatic waves propagating on an elastic plate and their stability

  • Published:
Japan Journal of Industrial and Applied Mathematics Aims and scope Submit manuscript

Abstract

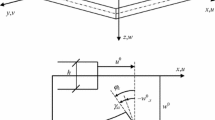
Plates are common structural elements of most engineering structures, including aerospace, automotive, and civil engineering structures. The study of plates from theoretical perspective as well as experimental viewpoint is fundamental to understanding of the behavior of such structures. The dynamic characteristics of plates, such as natural vibrations, transient responses for the external forces and so on, are especially of importance in actual environments. In this paper, we consider natural vibrations of an elastic plate and the propagation of a wavepacket on it. We derive the two-dimensional equations that govern the spatial and temporal evolution of the amplitude of a wavepacket and discuss its features. We especially consider wavenumber-based nearly bichromatic waves and direction-based nearly bichromatic waves on an elastic plate. The former waves are defined by the waves that almost concentrate the energy in two wavenumbers, which are very closely approached each other. The latter waves are defined by the waves that almost concentrate the energy in two propagation directions and two propagation directions are very close each other. The fact that the solution of the governing equation for wavenumber-based nearly bichromatic waves is stable is also shown.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

A:

slowly varying amplitude in space and/or time of a wavepacket

Cl :

the function space consisting of functionsf(x) which is lth differentiable at any point ofx and the lth derivative off is continuous

D:

plate flexural rigidity

E:

elastic modulus

G:

direction-based spectrum of a wavepacket or Green’s function

H:

value used in boundary conditions

L:

linear operator defined by Equations (A.19) and (A.20) or value used in boundary conditions

M:

positive integer

N:

positive integer

O:

function of order

Q:

function defined by Equation (A.18) or Fourier coefficient

R:

real space

S:

function used by the method of separation of variables in Equation (A.3)

U:

function used by the method of separation of variables in Equation (A.3)

a:

cosθ0

b:

sinθ0

c:

Fourier coefficient

cc:

complex conjugate

f:

function of initial condition

h:

plate thickness

i:

imaginary unit or unit vector alongx axis

j:

unit vector alongy axis

k:

wave number

m:

integer

n:

integer

t:

time

u:

function

v:

function

v:

velocity of envelope surface

w:

plane traveling waves

x, y:

rectangular coordinate system

Ψ:

function used by the method of separation of variables in Equation (A.9)

Φ:

function used by the method of separation of variables in Equation (A.9)

α:

coefficient in Equation (A.34)

β:

coefficient in Equation (A.34)

γ:

coefficient in Equations (A.10) and (A.11)

δ:

Delta function

ε:

order of time and spacial scales

θ:

propagation direction

λ:

separation constant

μ:

separation constant

ν:

Poisson’s ratio

ρ:

mass density

ω:

angular frequency

δD :

boundary of rectangle

∇4 :

biharmonic operator

0:

dominant value

1,2:

number of function or value

m:

number of coefficient

n:

number of function or coefficient

’:

differentiation with respect to wave numberk

∼:

modified value

∧:

modified value

*:

adjoint operator

References

  1. S. P. Timoshenko, Theory of Plates and Shells. McGraw-Hill, New York, 1940.

    MATH  Google Scholar 

  2. S.P. Timoshenko and S. Woinowsky-Krieger, Theory of Plates and Shells. McGraw-Hill, Singapore, 1970.

    Google Scholar 

  3. A.C. Ugural, Stresses in Plates and Shells. McGraw-Hill, New York, 1981.

    Google Scholar 

  4. A.W. Leissa, Vibration of Plates. NASA-Sp-160, 1969.

  5. H.N. Chu and G. Herrmann, Influence of large amplitudes on free flexural vibrations of rectangular elastic plates. J. Appl. Mech.,23, No. 2 (1956), 532–540.

    MATH  MathSciNet  Google Scholar 

  6. M.M. Hrabok and T.M. Hrudey, A review and catalog of plate bending finite elements. Computers and Structures,19, No. 3 (1984), 479–495.

    Article  Google Scholar 

  7. R.C. Averill and J.N. Reddy, Behavior of plate elements based on the first-order shear deformation theory. Engineering Computations,7 (1990), 57–74.

    Article  Google Scholar 

  8. J.N. Reddy, An Introduction to the Finite Element Method (2nd ed.). McGraw-Hill, New York, 1993.

    Google Scholar 

  9. R. Haberman, Elementary Applied Partial Differential Equations. Prentice Hall, Englewood Cliff, NJ, 1983.

    MATH  Google Scholar 

  10. Y. Goda, Numerical experiments on wave statistics with spectral simulation. Report Port Harbour Research Institute,9, No. 3 (1970), 3–57.

    Google Scholar 

  11. S.K. Chakrabarti, R.H. Snider and P.H. Feldhausen, Mean length of runs of ocean waves. J. Geophys. Res.,79, No. 36 (1974), 5665–5667.

    Article  Google Scholar 

  12. M.S. Longuet-Higgins, Statistical properties of wave groups in a random sea-state. Phil. Trans. Roy. Soc. London, Series A,312 (1984), 219–250.

    Article  MATH  MathSciNet  Google Scholar 

  13. H. Washimi and T. Taniuti, Propagation of ion-acoustic solitary waves of small amplitude. Phys. Rev. Lett.,17 (1966), 996–998.

    Article  Google Scholar 

  14. G.P. Agrawal, Fiber-Optic Communication System (2nd ed.). Wiley, New York, 1997.

    Google Scholar 

  15. A.H. Nayfeh and D.T. Mook, Nonlinear Oscillations. Wiley, New York, 1979.

    MATH  Google Scholar 

  16. B.T. Nohara, Consideration of higher order governing equations of envelope surface and governing equations of envelope surface created by directional, nearly monochromatic waves. Internat. J. Differential Equations and Applications,6, No. 2 (2002), 209–225.

    MATH  MathSciNet  Google Scholar 

  17. B.T. Nohara, Derivation and consideration of governing equations of the envelope surface created by directional, nearly monochromatic waves. Internat. J. Nonlinear Dynamics and Chaos in Engineering Systems,31, No. 4 (2003), 375–392.

    MATH  MathSciNet  Google Scholar 

  18. B.T. Nohara, A note on the derivation of governing equations of envelopes created by nearly bichromatic waves — the modified Schrödinger equation and the modified Schrödinger-Nohara equation -. J. Differential Equations and Control Processes,2, No. 2 (2003), 23–34.

    MathSciNet  Google Scholar 

  19. B.T. Nohara, A Comment on governing equations of envelope surface created by a two-dimensional directional wavepacket centered around a propagation direction on an elastic plate. Internat. J. Nonlinear Dynamics and Chaos in Engineering Systems,35, No. 2 (2004), 175–186.

    MATH  Google Scholar 

  20. H. Reismann, Elastic Plates: Theory and Application. Wiley, New Jersey, 1988.

    MATH  Google Scholar 

  21. A.H. Nayfeh, Perturbation Methods. Wiley, New York, 2002.

    Google Scholar 

  22. A. Zygmund, Trigonometric Series (2nd ed.), Vol.11. Cambridge University Press, 1988, 301.

Download references

Author information

Authors and Affiliations

  1. Department of Electronic and Computer Engineering, Musashi Institute of Technology, Tamazutsumi, Setagaya, 158-8557, Tokyo, Japan

    Ben T. Nohara

Authors
  1. Ben T. Nohara
    View author publications

    You can also search for this author in PubMed Google Scholar

About this article

Cite this article

Nohara, B.T. Governing equations of envelope surface created by nearly bichromatic waves propagating on an elastic plate and their stability. Japan J. Indust. Appl. Math. 22, 87 (2005). https://doi.org/10.1007/BF03167478

Download citation

  • Received:

  • Revised:

  • DOI: https://doi.org/10.1007/BF03167478

Key words

Search

Navigation