Skip to main content
SpringerLink
Log in

Reanalysis of the 3D quasi-stationary grain size distribution based on Hillert's grain growth rate equation

  • Published:
Science in China Series E: Technological Sciences Aims and scope Submit manuscript

Abstract

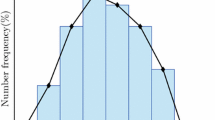
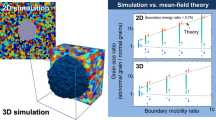
Based on Hillert's 3D grain growth rate equation, the grain growth continuity equation was solved. The results show that there are an infinite number of 3D quasi-stationary grain size distributions. This conclusion has gained strong supports from results of different computer simulations reported in the literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Feppon, J. M., Hutchinson, W. B., On the growth of grains, Acta Mater., 2002, 50: 3293–3300.

    Article  Google Scholar 

  2. Liu Guoquan, Yu Haibo, Song Xiaoyan et al., A new model of three-dimensional grain growth: Theory and computer simulation of topology-dependency of individual grain growth rate, Materials and Design, 2001, 22:33–38.

    Article  Google Scholar 

  3. Liu Guoquan, Yu Haibo, Qin Xiangge, Three-dimensional grain topology—size relationships in a real metallic polycrystal compared with theoretical models, Materials Science and Engineering A, 2002, 326 276–281.

    Article  Google Scholar 

  4. Hillert, M., On the theory of normal and abnormal grain growth, Acta Metall., 1965, 13(3): 227–238.

    Article  Google Scholar 

  5. Hillert, M., Analytical treatments of normal grain growth, Materials Science Forum, 1996, 202–206: 3–18.

    Article  Google Scholar 

  6. Wagner, C., Thoerie der altering von neiderschlagen durch umlosen, Z. Elektrochem, 1961, 65: 581–591.

    Google Scholar 

  7. Brown, L. C., A new reexamination of classical coarsening theory, Acta Metall., 1989, 37(1): 71–77.

    Article  Google Scholar 

  8. Coughlan, S. D., Fortes, M. A., Self-similar size distribution in particle coarsening, Scripta Metall. Mater., 1993, 28: 1471–1476.

    Article  Google Scholar 

  9. Rios, P. R., Comparison between a computer simulated and an analytical grain size distribution, Scripta Mater., 1999, 40(6): 665–668.

    Article  Google Scholar 

  10. Atkinson, H. V., Theories of normal grain growth in pure single phase system, Acta Metal., 1988, 36(3), 469–491.

    Article  Google Scholar 

  11. Weygand, D., Brechet, Y., Three-dimensional grain growth A vertex dynamics simulation, Phil. Mag. B, 1999, 79(5): 703–716.

    Google Scholar 

  12. Wakai, F., Enomoto, N., Ogawa, H., Three-dimensional microstructural evolution in ideal grain growth—general statistics, Acta Mater., 2000, 48: 1297–1311.

    Article  Google Scholar 

  13. Krill, III C. E., Chen, L. Q., Computer simulation of 3D grain growth using a phase-field model, Acta Mater., 2002, 50: 3057–3073.

    Google Scholar 

  14. Song Xiaoyan, Liu Guoquan, Gu Nanju, Re-analysis on grain size distribution during normal grain growth based on Monte Carlo simulation, Scripta Mater., 2000, 43: 355–359.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Materials Science and Engineering, University of Science and Technology Beijing, 100083, Beijing, China

    Wang Chao & Liu Guoquan

Authors
  1. Wang Chao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Liu Guoquan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Liu Guoquan.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wang, C., Liu, G. Reanalysis of the 3D quasi-stationary grain size distribution based on Hillert's grain growth rate equation. Sci. China Ser. E-Technol. Sci. 47, 112–120 (2004). https://doi.org/10.1007/BF03037688

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF03037688

Keywords

Search

Navigation