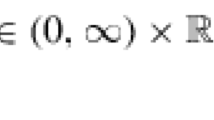Abstract
Let μ be any probability measure onR with λ |x|dμ(x)<∞, and let μ* denote its associated Hardy and Littlewood maximal p.m. It is shown that for any p.m.v for which μ<ν<μ* in the usual stochastic order, there is a martingale (X t)0≦t≦1 for which sup0≦t≦1 X t andX 1 have respective p.m. 'sv and μ. The proof uses induction and weak convergence arguments; in special cases, explicit martingale constructions are given. These results provide a converse to results of Dubins and Gilat [6]; applications are made to give sharp martingale and ‘prophet’ inequalities.
Similar content being viewed by others
References
J. Azema and M. Yor,Une solution simple au probleme de Skorokhod, inSem Probab. XIII, Lecture Notes in Math. 721, Springer-Verlag, New York, 1979, pp. 90–115.
J. Azema and M. Yor,Le probleme de Skorokhod: complements a l'expose precedent, inSem. Probab. XIII, Lecture Notes in Math. 721, Springer-Verlag, New York, 1979, pp. 625–633.
D. P. Bertsekas and S. E. Shreve,Stochastic Optimal Control: The Discrete Time Case, Academic Press, New York, 1978.
P. Billingsley,Convergence of Probability Measures, Wiley, New York, 1968.
D. Blackwell and L. E. Dubins,A converse to the dominated convergence theorem, Illinois J. Math.7 (1963), 508–514.
L. E. Dubins and D. Gilat,On the distribution of maxima of martingales, Proc. Am. Math. Soc.68 (1978), 337–338.
L. E. Dubins and J. Pitman,A maximal inequality for skew fields, Z. Wahrscheinlichkeitstheor. Verw. Geb.52 (1980) 219–227.
S. N. Ethier and T. G. Kurtz,Markov Processes Characterization and Convergence, Wiley, New York, 1986.
D. Gilat,On the ratio of the expected maximum of a martingale and the L p-norm of its last term, Isr. J. Math.63 (1988), 270–280.
G. H. Hardy and J. E. Littlewood,A maximal theorem with function theoretic applications, Acta. Math.54 (1930), 81–116.
T. P. Hill and R. P. Kertz,Stop rule inequalities for uniformly bounded sequences of random variables, Trans. Am. Math. Soc.278 (1983), 197–207.
S. D. Jacka,Doob's inequality revisited: a maximal H 1-embedding, Stoch. Processes Appl,29 (1988), 281–290.
I. Karatzas and S. E. Shreve,Brownian Motion and Stochastic Calculus, Springer-Verlag, New York, 1988.
U. Krengel and L. Sucheston,Semiamarts and finite values, Bull. Am. Math. Soc.83 (1977), 745–747.
U. Krengel and L. Sucheston,On semiamarts, amarts, and processes with finite value, inAdvances in Probability, Vol. 4, Marcel Dekkar, New York, 1978, pp. 197–266.
P. A. Meyer,Probability and Potentials, Blaisdell Publ. Co., Waltham, Mass., 1966.
E. Perkins,The Cereteli—Davis solution to the H 1-embedding problem and an optimal embedding in Brownian motion, inSeminar on Stochastic Processes, Birkhäuser, Boston, 1985.
V. Pestien,An extended Fatou equation and continuous-time gambling, Adv. Appl. Prob.14 (1982), 309–323.
S. I. Resnick,Extreme Values, Regular Variation, and Point Processes, Springer-Verlag, New York, 1987.
G. R. Shorack and J. A. Wellner,Empirical Processes with Applications to Statistics, Wiley, New York, 1986.
D. Stoyan,Comparison Methods for Queues and Other Stochastic Models (D. J. Daley, ed.), Wiley, New York, 1983.
V. Strassen,The existence of probability measures with given marginals, Ann. Math. Statist.36 (1965), 423–439.
D. P. van der Vecht,Inequalities for Stopped Brownian Motion, C. W. I. Tract 21, Mathematisch Centrum, Amsterdam, 1986.
Author information
Authors and Affiliations
Additional information
Supported in part by NSF grants DMS-86-01153 and DMS-88-01818.
Rights and permissions
About this article
Cite this article
Kertz, R.P., Rösler, U. Martingales with given maxima and terminal distributions. Israel J. Math. 69, 173–192 (1990). https://doi.org/10.1007/BF02937303
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02937303