Skip to main content
SpringerLink
Log in

The influence of gas-liquid properties on gas driven waves in a film

  • Two-Phase Flows
  • Published:
Microgravity Science and Technology Aims and scope Submit manuscript

Abstract

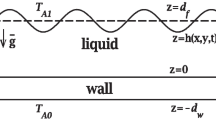
The paper deals with a two-layer flow in microgravity, when the upper light fluid (gas) drives the lower layer and forces the wave motion at the interface. The flow is simulated numerically by solving the coupled 2D problem for the Navier-Stokes equations. Gas phases with different viscosities and densities are considered and the influence of these properties on wave characteristics is investigated. It has been shown that film Reynolds number depends on density ratio when the viscosity ratio is fixed. Another result is that the wave amplitude and phase speed at a given film Reynolds number depend on gas viscosity and density. The flow with heavy gas can result in waves with equal amplitudes but different phase speed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. R. Miesen andB.J. Boersma: “Hydrodynamic stability of a sheared liquid film”. J. Fluid Mech. vol. 301, 175 (1995).

    Article  MATH  MathSciNet  Google Scholar 

  2. T.M. Segin, B.S. Tilley andL. Kondic: “On undercompressive shocks and flooding in countercurrent two-layer flows”. J. Fluid Mech. vol. 532, 217 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  3. L.S. Cohen andT.J. Hanratty: “Generation of waves in the concurrent flow of air and a liquid”. AIChEJ vol. 11, 138 (1965).

    Article  Google Scholar 

  4. A.D.D. Craik: “Wind-generated waves in thin liquid films”. J. Fluid Mech. vol. 26, 369 (1966).

    Article  MATH  Google Scholar 

  5. L.A. Jurman andM.J. McCready: “Study of waves on thin liquid films sheared by turbulent gas flows”. Phys. Fluids A vol. 1, 522 (1989).

    Article  Google Scholar 

  6. A.M. Frank: “Shear driven solitary waves on a liquid film”. Phys. Review E vol. 74, 065301 (2006).

    Article  Google Scholar 

  7. A.M. Frank: “Method of particles for incompressible flows with free surface”. Notes on Numerical Fluid Mechanics and Multidisciplinary Design vol. 88, p. 189 (Springer, Berlin, 2005)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. ICM SB RAS, 660036, Akademgorodok, Krasynoarsk, Russia

    Alexander M. Frank

Authors
  1. Alexander M. Frank
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexander M. Frank.

Additional information

Paper was presented on the Second International Topical Team Workshop on TWO-PHASE SYSTEMS FOR GROUND AND SPACE APPLICATIONS October 26–28, 2007, Kyoto, Japan.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Frank, A.M. The influence of gas-liquid properties on gas driven waves in a film. Microgravity Sci. Technol 19, 38–40 (2007). https://doi.org/10.1007/BF02915745

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02915745

Keywords

Search

Navigation