Wave recursive interpolation

Abstract

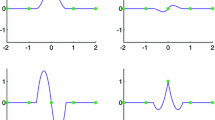
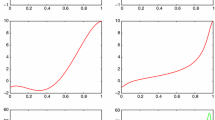
In this paper it is studied that the generated theory of wave recursive inter polation of uniform T-subdivision scheme include wave parameter.

The paper analyses the convergence of sequences of control polygons produced by wave recursive inter polation T-subdivision scheme of the form

$$\left\{ {\begin{array}{*{20}c} {P_{Tm}^{k + 1} = P_m^h ,} \\ {P_{Tm + j}^{k + 1} \left( \beta \right) = \sum\limits_{i = 1}^k {\left( {P_{m - i + 1}^k a_{i,j} \left( \beta \right) + P_{m + i}^k a_{i,T - j} \left( \beta \right)} \right)} } \\ \end{array} } \right.$$

, j=1,2,...,T−1; m=0,1,...,nT^k; k=0,1,2,.... and differentiability of the limit curve.