Non perturbative effective potentials of quantum oscillators

Abstract

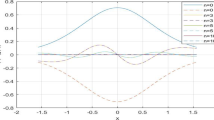
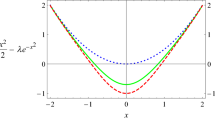
Non perturbative analogues of the Gaussian effective potential (GEP) are defined for quantum oscillators obeyingq—or (q,p)—deformed commutation relations. These are called the non perturbativeq-effective potential (NP _q EP) and the non perturbativeqp effective potential (NP _qp EP), in the respective cases. A system-specific effective potential (SSEP) is also introduced by means of an additional minimization with respect to theq orq andp parameters. The method is applied toq and (q,p) oscillators of the quartic and sextic types. The SSEP in the case of ground states of theq-oscillators corresponds toq=1, which is the ordinary bosonic limit. A potential shape transition that involves the conversion of a double well to a single well or vice versa, is seen to exist in the case of quantum oscillators sitting in a double well potential.