Abstract
A method is described for inverting the Mellin transform which uses an expansion in Laguerre polynomials and converts the Mellin transform to the Laplace transform, then the Laplace transform is converted to the first kind convolution integral equation by a suitable substitution.
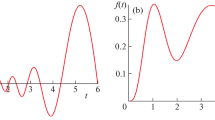
The integral equation so obtained is an ill-posed problem and we use the spline regularization to solve it. The performance of the method is illustrated by the inversion of the test functions available in the literature [J. Inst. Math. & Appl. 20 (1977), p. 73], [J. Math. Comp. 53 (1989), p. 589], [J. Sci. Stat. Comp. 4 (1983), p. 164]. The effectiveness of the method is shown by results obtained demonstrated by means of tables and diagrams.
Similar content being viewed by others
References
Aki, K. and Richards, G., Quantitative Seismology: Theory and Methds', Freeman, San Francisco, (1980).
Ang, D. D. et al, Complex Variable and Regularization Methods of Inversion of the Laplace Transform, J. Math. Comp. 53(1989), 589–608.
Brianzi, P., A Criterion for the Choice of a Sampling Parameter in the Problem of Laplace Transform Inversion, J. Inv. Probls. 10(1994), 55–61.
Budinger, T. F., Physical Attributes of Single-Photon Tomography, J. Nucl. Med., 21 (1980), 6.
Davies, B. and Martin, B., Numerical Inversion of the Laplace Transform, J. Comp. Physics, 33(1979) No. 2, 1–32.
De Hoog, F. R., Review of Fredholm Equations of the First Kind, In the Application and Numerical Solutions of Interal Equations, Editors, R. S. Anderssen, F. R. de Hoog and M. A. Lucas, Published by Sijthoff and Noordhoff (1980).
Essah, W. A. and Delves, L. M., On the Numerical Inversion of the Laplace Transform, J. Inv. Problems, 4 (1988), 705–724.
Franzone, P. C. et al., An Approach to Inverse Calculation of Epi-cardiol Potentials from Body Surface Mpas, J. Adv. Cardiol 21(1977), 167–170.
Gautschi, W., Attenuation factor in practical Fourier Analysis, Numer. Math., 18 (1972), 373–400.
Gelfat, V. I., Kosarev, E. L. and Podolyak, E. R., Programs for Signal Recovery from Noisy data Using the Maximum Likelihood Principle, Computer Physics Communications 74 (1993), 335–348.
Grunbaum, F. A., Remark on the Phase Problem in Crystallography, Proc. Nat. Acad. Sci. U.S.A., 72(1975), 1699–1701.
Jaynes, E. T., Papers on Problability, Statistics and Statistical Physics, Synthese Library (1983).
Karavaris, C. and Seinfeld, J. H., Identification of Parameters in Distributed Parameter Systems by Regularization, SIAM. J. Control. Optim., 23 (1985), 217–241.
McWhirter, J. G. and Pike, E. R., On the Numerical Inversion of the Laplace Transform and Similar FI Equations of the First Kind, J. Phys. A, 11(1978), 1729–1745.
Mendelsohn, J. and Rice, J., Deconvolution of Micro-Fluorometric Histograms with B-Splines, J. Amer. Statist. Assoc., 77(1982), 748–753.
Papoulis, A., A New Method of Inversion of Laplace Transform, Quarterly Appl. Math., 14 (1956), 405–414.
Pennisi, L.L., Elements of Complex Variables, McGraw-Hill, New York (1976).
Smith, W., The Retrieval Atmospheric Profiles From VAS Geostationary Radiance Observation, J. Atmospheruc Sci., 40(1983), 2025–2035.
Snedon, J.N., The Use of Integral Transforms, McGraw-Hill, New York, (1972).
Stoer, J. and Bulirsch, R., Introduction to Numerical Analysis, Springer-Verlag, (1978).
Szego, G., Orthogonal Polynomials, American Math. Society Colloq. Publications, 23 (AMS Providence, RI 3rd edition) (1967).
Talbot, A., The Accurate Numerical Inversion of Laplace Transform. J. Inst. Maths. Applics, 23(1979), 97–120.
Theocaris, P. and Chrysakis, A. C., Numerical Inversion of the Mellin Transform. J. Math. and Appl., 20(1977), 73–83.
Tikhonov, A. N., Solutions of Incorrectly Formulated Problems and Regularization Method, Soviet Math. Dokl., 4(1963), 1035–1038.
Tikhonov, A. N and Arsenin, V. Y., Solutions of Ill-Posed Problems, (Translated From Russian) Wiley Publishing Co. New York (1977).
Varah, J. M., Pitfalls in the Numerical Solution of Linear Ill-Posed Problems, SIAM J. Sci. Stat. Comp., 4(1983), 164–176.
Wahba, G., Practical Approximation Solutions to Linear Operator Equations when the Data are Noisy, SIAM J. Numer. Anal., 14(1977), 651–677.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Iqbal, M. Spline regularization of numerical inversion of Mellin transform. Approx. Theory & its Appl. 16, 1–16 (2000). https://doi.org/10.1007/BF02845223
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02845223