Semiconductor quantum dots: Theory and phenomenology

Abstract

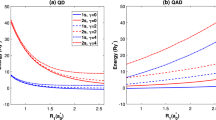
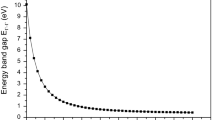
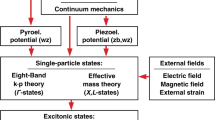
Research in semiconductor quantum dots (q-dots) has burgeoned in the past decade. The size (R) of these q-dots ranges from 1 to 100 nm. Based on the theoretical calculations, we propose energy and length scales which help in clarifying the physics of this mesoscopic system. Some of these length scales are: the Bohr exciton radius (α_B*), the carrier de Broglie and diffusion length (λ_D andl _D), the polaron radius (α_p), and the reduction factor modulating the optical matrix element (M _x).R<α_B is an individual particle confinement regime, whereas the larger ones are exciton confinement regime wherein Coulomb interaction play an important role. Similarly a size-dependent dielectric constantε(R) should be used forR<α_p<α_B. An examination ofM _x reveals that an indirect gap material q-dot behaves as a direct gap material in the limit of very small dot size. We have carried out effective mass theory (EMT) calculations to estimate the charge density on the surface of the quantum dot. We present tight binding (TB) calculation to show that the energy upshift scales as 1/R ^x, wherex is less than 2 and the exponent depends on the orientation of the crystallite.