Skip to main content
SpringerLink
Log in

Bulk viscosity and the inflationary universe

  • Published:
Lettere al Nuovo Cimento (1971-1985)

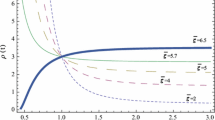
Summary

Phase transitions from a universe with nonnegligible bulk viscosity to an inflationary one are possible only if viscosity virtually disappears in the process. This suggests the phase change involved is of the type normal fluid to superfluid. Some exact solutions of Einstein equations for a viscous fluid are also given. They show the subtle interplay of viscosity and equations of state.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. S. W. Hawking:Astrophys. J.,145, 544 (1966);C. W. Misner:Astrophys. J.,151, 431 (1968);S. Weinberg:Gravitation and Cosmology (J. Wiley and Sons, New York, N. Y., 1972), p. 469 ff.

    Article  ADS  Google Scholar 

  2. W. Israel andJ. N. Vardalas:Lett. Nuovo Cimento,4, 887 (1970).

    Article  Google Scholar 

  3. S. Weinberg:Astrophys. J.,168, 175 (1971). See also N. Straumann:Helv. Phys. Acta,49, 269 (1976).

    Article  ADS  Google Scholar 

  4. M. Heller andL. Susztcki:Acta Phys. Pol. B,5, 345 (1974);M. Heller andZ. Klimek:Astrophys. Space Sci.,33, L37 (1975).

    ADS  Google Scholar 

  5. M. Heller, Z. Klimek andL. Susztcki:Astrophys. Space Sci.,20, 205 (1973);M. Szydlowski andM. Heller:Acta Phys. Pol. B,14, 303 (1983);S. R. Rot andO. P. Tiwari:Indian J. Pure Appl. Math.,14, 233 (1983);A. A. Coley andB. O. J. Tupper:Phys. Rev. D,29, 2701 (1984);Astrophys. J.,288, 418 (1985);N. O. Santos,R. S. Dias andA. Banerjee:J. Math. Phys. (N.Y.),26, 878 (1985).

    Article  ADS  Google Scholar 

  6. G. E. Tauber:Astrophys. Space Sci.,57, 163 (1978).

    Article  MathSciNet  ADS  Google Scholar 

  7. G. Neugebaubr andH. Strobel: Wissen. Zeitschr. der Friedrich-Schiller-Universität, Jena Jahrg. 18 (1969).

  8. R. Treciokas andG. F. R. Ellis:Commun. Math. Phys.,23, 1 (1971).

    Article  MathSciNet  ADS  Google Scholar 

  9. J. D. Nightingale:Astrophys. J.,185, 105 (1973).

    Article  MathSciNet  ADS  Google Scholar 

  10. G. Neugebauer andH. Strobel, ref. (7), assume (1) as equation of state. The same assumption is made throughout this paper.

  11. A. H. Guth:Phys. Rev. D,23, 347 (1981).

    Article  ADS  Google Scholar 

  12. D. Kramer,H. Stephani,M. MacCallum andE. Herlt:Exact solutions of Einstein field equations, VEB Deutscher Verlag der Wissenschatten (Berlin, 1980), p. 166.

  13. S. Weinberg:Gravitation and Cosmology (J. Wiley and Sons, New York, N. Y., 1972), p. 469 ff

    Google Scholar 

  14. G. Papini andM. Weiss:Phys. Lett. A,89, 329 (1982);Lett. Nuovo Cimento 44, 83, (1985).

    Article  MathSciNet  ADS  Google Scholar 

  15. G. F. R. Ellis:Relativistic cosmology, inProc. S.I.F., Course XLVII (Academic Press, New York, N. Y., 1971).

    Google Scholar 

  16. B. L. Hu:Phys. Lett. A,90, 375 (1982).

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Physics and Astronomy, University of Begina, S4S 0A2, Regina, Sask., Canada

    G. Papini & M. Weiss

Authors
  1. G. Papini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. Weiss
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Work partially supported by the Natural Sciences and Engineering Research Council of Canada.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Papini, G., Weiss, M. Bulk viscosity and the inflationary universe. Lett. Nuovo Cimento 44, 612–616 (1985). https://doi.org/10.1007/BF02746773

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02746773

PACS

Search

Navigation