Nonlinear evolution analysis of T-S disturbance wave at finite amplitude in nonparallel boundary layers

Abstract

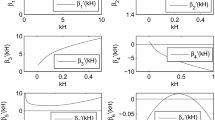
The nonlinear evolution problem in nonparallel boundary layer stability was studied. The relative parabolized stability equations of nonlinear nonparallel boundary layer were derived. The developed numerical method, which is very effective, was used to study the nonlinear evolution of T-S disturbance wave at finite amplitudes. Solving nonlinear equations of different modes by using predictor-corrector and iterative approach, which is uncoupled between modes, improving computational accuracy by using high order compact differential scheme, satisfying normalization condition, determining tables of nonlinear terms at different modes, and implementing stably the spatial marching, were included in this method. With different initial amplitudes, the nonlinear evolution of T-S wave was studied. The nonlinear nonparallel results of examples compare with data of direct nemerical simulations (DNS) using full Navier-Stokes equations.