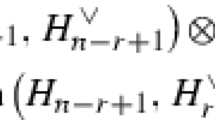Skip to main content
SpringerLink
Log in

Instantons and jumping lines

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

We study the behaviour under deformation of holomorphic bundles of rank 2 over ℙ1 (ℂ). This is then applied to the description of the moduli space\(\tilde M_n \) of framed SU(2) instantons of chargen;\(\tilde M_n \) is shown to map to ℂn, with equidimensional fibers. We use this to provide a stratification of\(\tilde M_n \) and compute the strata explicitly to codimension 4. This then yields π1(\(\tilde M_n \))=ℤ2, and, for the standard moduli spaceM n, π1(M n)=0 forn odd, ℤ2 forn even.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Atiyah, M.F.: The geometry of Yang-Mills fields. Accademia Nazionale dei Lincei, Scuola Normale Superiore, Pisa 1979

  2. Atiyah, M.F.: Instantons in two and four dimensions. Commun. Math. Phys.93, 437–451 (1984)

    Google Scholar 

  3. Atiyah, M.F., Drinfeld, V.G., Hitchin, N.J., Manin, Yu.I.: Construction of instantons. Phys. Lett.65A, 185–187 (1978)

    Google Scholar 

  4. Barth, W.: Some properties of stable rank-2 vector bundles on ℙ n . Math. Ann.226, 125–150 (1977)

    Google Scholar 

  5. Barth, W.: Moduli of vector bundles on the projective plane. Invent. Math.42, 63–91 (1977)

    Google Scholar 

  6. Donaldson, S.K.: Instantons and geometric invariant theory. Commun. Math. Phys.93, 453–461 (1984)

    Google Scholar 

  7. Grauert, H., Mülich, G.: Vektorbündel vom Rang 2 über demn-dimensionalem komplex projektiven Raum. Manuscr. Math.16, 75–100 (1975)

    Google Scholar 

  8. Grothendieck, A.: Eléments de géométrie algébrique. III. Publ. Math. IHES

  9. Grothendieck, A.: Sur la classification des fibres holomorphes sur la sphère de Riemann. Am. J. Math.79, 121–138 (1957)

    Google Scholar 

  10. Hartshorne, R.: Algebraic geometry. Berlin, Heidelberg, New York: Springer GTM (1978)

    Google Scholar 

  11. Hartshorne, R.: Stable vector bundles and instantons. Commun. Math. Phys.59, 1–15 (1978)

    Google Scholar 

  12. Horrocks, G.: Vector bundles on the punctured spectrum of a local ring. Proc. Lond. Math. Soc. (3)14, 689–713 (1964)

    Google Scholar 

  13. Okonek, C., Schneider, M., Spindler, H.: Vector bundles on complex projective spaces. Prog. Math. 3. Boston: Birkhäuser 1980

    Google Scholar 

  14. Le Potier, J.: Fibres stables de rang 2 sur ℙ2(ℂ). Math. Ann.241, 217–256 (1979)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Département de Mathématiques, U.Q.A.M., Succursale “A”, C.P.8888, H3C 3P8, Montreal, Quebec, Canada

    Jacques Hurtubise

Authors
  1. Jacques Hurtubise
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Communicated by A. Jaffe

Research supported in part by NSERC grant A8361 and by FCAR grant EQ2354

Rights and permissions

Reprints and permissions

About this article

Cite this article

Hurtubise, J. Instantons and jumping lines. Commun.Math. Phys. 105, 107–122 (1986). https://doi.org/10.1007/BF01212344

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01212344

Keywords

Search

Navigation