Abstract
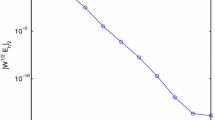
This paper presents a fully spectral discretization method for solving KdV equations with periodic boundary conditions: Chebyshev pseudospectral approximation in the time direction and Fourier Galerkin approximation in the spatial direction. The expansion coefficients are determined by minimizing an object functional. Rapid convergence of the method is proved.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
B. C.Jiang, et al., Spectral Methods in Computational Physics, Hunan Science and Technology Press, Changsha, (1989) (in Chinese)
G.Ierley, et al., Spectral methods in time for a class of parabolic partial differential equations, J. Comp. Phys., 102 (1992), 88–97.
R. K.Dodd, Solitons and Nonlinear Wave Equations, Academic Press, London (1982).
D. R. Wang, Methods for Solving Nonliear Equations and Optimization Methods, People's Education Press, (1979). (in Chinese)
R. G.Voigt, et al., Spectral Method for PDE, SIAM Philadelpia (1984).
C.Canuto, et al., Analysis of the combinated finite element and Fourier interpolation, Numer. Math., 39 (1982), 205–220.
D. Q. Li, et al., Nonlinear Evolutional Equations, Science Press, (1980). (in Chinese)
P.Dutt, Spectral methods for initial boundary value problem — an alternative approach, SIAM J. Numer. Anal., 27, 4 (1990), 885–903.
D. Gottlieb, Numerical analysis of spectral method, CBMS-NSF, Regional Conference Series in Applied Math., 26 (1977)
R. A. Adams, Sobolev Spaces, Academia Press (1975).
Author information
Authors and Affiliations
Additional information
Communicated by Liu Ciqun
Rights and permissions
About this article
Cite this article
Shengchang, W., Xiaoqing, L. Spectral method in time for KdV equations. Appl Math Mech 17, 373–378 (1996). https://doi.org/10.1007/BF00193801
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF00193801